The radius of a pipe decreases according to r=roe−∝x , where α=0.50m−1andx is the distance of a cross - section from the first end (x=0) . Find the ratio of Reynolds number for two cross -sections lying at the distance of 2m from each other . (take e=2.718)Solution in Gujrati
Reynold number NR=ρvDη
∴ For a given liquid NR∝vD
∴(NR)1(NR)2=v1v2×D1D2 ….(1)
According to the equation of continuity,
A1v1=A2v2
∴πr21v1=πr22v2
∴v1v2=r22r21=(D2D1)2 ....(2)
From equation (1) and (2) ,
(NR)1(NR)2=roe−∝x2r−∝x1o=e−α(x2−x1)
=e−(0.5)(2)
=e−1
12.718
∴(NR)1(NR)2=0.368
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDS BOOK - KUMAR PRAKASHANCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - SECTION -C (Objective Questions) (VSQs)
100 Videos
- MECHANICAL PROPERTIES OF FLUIDS BOOK - KUMAR PRAKASHANCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - SECTION -C (Objective Questions) (VSQs) Fill in the Blank
9 Videos
- MECHANICAL PROPERTIES OF FLUIDS BOOK - KUMAR PRAKASHANCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - SECTION - B Numerical From Textual Exercise
44 Videos
- MACHANICAL PROPERTIES OF SOLIDS BOOK - KUMAR PRAKASHANCHAPTER - MACHANICAL PROPERTIES OF SOLIDS EXERCISE - QUESTION PAPER
11 Videos
- MOTION IN A STRAIGHT LINE BOOK - KUMAR PRAKASHANCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - QUESTION PAPER
11 Videos
Similar Questions
Bag and suitcase have broad (wide) handle why ?
View SolutionAngle of contact increases with rising temperature of liquid .
View SolutionExplain why
(a) The blood pressure in humans is greater at the feet than at the brain.
View SolutionWhat is called brittle material ?
View SolutionDefine frame of reference and give its types.
View SolutionWater is flowing with a speed of 2m//s in a horizontal pipe with cross-sectional area decreasing from 2xx10^(-2) m^(2) to 0.01 m^(2) at pressure 4xx10^(4) pascal. What will be the pressure at smaller cross-section?
View SolutionThe cross-sectional radius of a pipeline decreases graudally as r=r_0e^(-alphax) , where alpha=0.50ms^-1 , x is the distance from the pipeline inlet. Find the ratio of Reynolds numbers of two cross-sections separated by Deltax=3.2m .
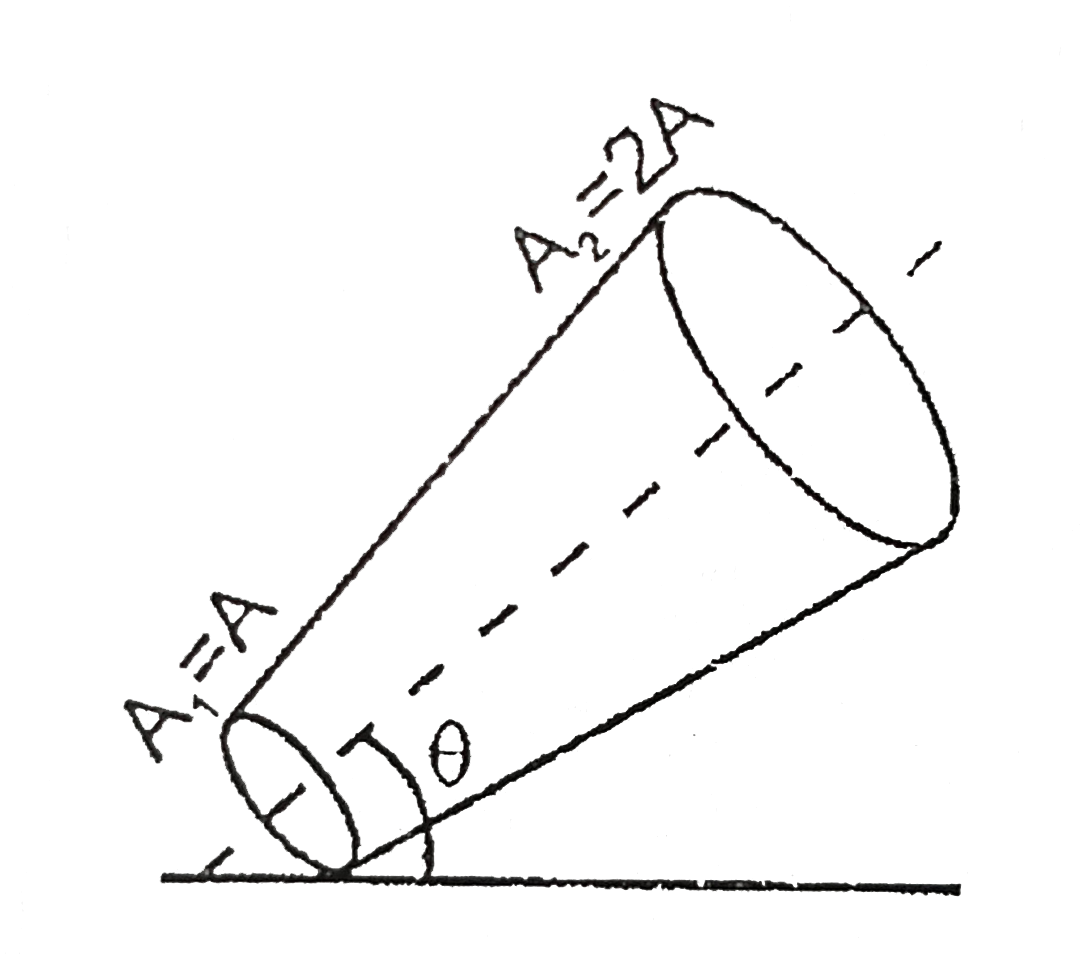
View SolutionA portion of a tube is shown in the figure. Fluid is flowing from cross-section area A1 to A2. The two cross-sections are at distance 'l' from each other. The velocity of the fluid at section A2 is √gl2. If the pressures at A1 & A2 are same, then the angle made by the tube with the horizontal will be:
View SolutionIn a long wire of square cross-section of side length l, current density varies with distance x from one edge of cross-section as
J=aehxA/m2
Where a and b are positive constants. Find the current flowing in wire.
[alb(e−1)]
View SolutionAssertion : When an ideal fluid flows through a pipe of non-uniform cross-section, then pressure is more at that section where area is more if the pipe is horizontal. Reason : According to Bernoulli's theorem speed at broader cross-section will be less.
View SolutionA current is flowing through a cylinderical conductor of radius R, such that current density at any cross-section is given by J=J0(1−rR), where r is radial distance from axis of the cylinder .Calculate the total current through the cross-section of the conductor.
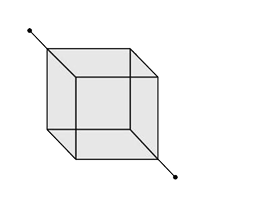
View SolutionTwelve wires of equal length and same cross-section are connected in the form of a cube. If the resistance of each of the wires is R, and the effective resistance between the two diagonal ends is Re, then the ratio RRe is equal to __.
View SolutionR त्रिज्या के बेलनाकार चालक के सिरों पर धारा घनत्व का परिमाण समीकरण J=J0(1−rR) के अनुसार बदलता है जहाँ केन्द्रीय अक्ष से दूरी r है। मान उस अक्ष (r = 0) पर J0 हे = रूप से घटकर शून्य जाता है। चालक के अनुप्रस्थ काट हो क्षेत्रफल A तथा J0 के पदों में धारा का मान होगा :
View SolutionA horizontal pipe of area of cross-section a and 3a respectively, then the ratio of velocity of flow at two different cross-section (If flow is streamline) is
View SolutionAssertion : When an ideal fluid flows through a pipe of non-uniform cross-section, then pressure is more at that section where area is more if the pipe is horizontal.
Reason : According to Bernoulli's theorem speed at broader cross-section will be less.
View Solution
