The specific resistance ρ of a thin wire of radius r cm, resistance R ohm and length L is given by ρ=πr2RL.IfL=78±0.01cm r=0.26±0.02andR=32±1Ω, What is the percentage error in ρ?
The correct Answer is:18.51%
Max. percentage error in ρ=πr2RLis
Δρρ×100=±(2Δrr+ΔRR+ΔLL)×100
=±(2×0.020.26+132+0.0178)×100
=±(15.38+3.12+0.012)=±18.51%
Topper's Solved these Questions
- PHYSICAL WORLD AND MEASUREMENTBOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Test Your Grip
50 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Fill in the blanks
50 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Value Based Questions
5 Videos
- OSCILLATIONS AND WAVESBOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - multiple choice Questions
13 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Multiple choice questions
7 Videos
Similar Questions
The specific resistance ρ of a circular wire is given by ρ=πr2R/l . Given: radius r=0.20±0.02cm , resistance R=25+1Ω , and length 1=5.00±0.01cm. The percentage error in ρ is ______ %.
View SolutionGiven R1=5.0±0.2Ω,andR2=10.0±0.1Ω. What is the total resistance in parallel with possible % error?
View Solution
Knowledge Check
The specific resistance ρ of a circular wire of radius r, resistance R, and length l is given by ρ=πr2R/l. Given : r=0.24±0.02cm,R=30±1Ω , and l=4.80±0.01cm. The percentage error in ρ is nearly
A7%B9%C13%D20%For finding the specific resistance of a wire, a student took the following readings by using a metre scale, a micrometer and a resistance box.
Length of the wire (L) = 100.0 cm
Radius (r ) = 0.100 cm and R = 10Ω
What is the maximum possible percentage error in the value of the specific resistance?A9.8%B12.1%C15.5%D18%The radius (r), length (l) and resistance (x) of a thin wire are
(0.2±0.02)cm,(80±0.1)cm, and (30±1)Ω respectively. The percentage error in the specific resistance isA23.4%B25.4%C26%D27.5%
If L= inductance and R = resistance, then the unit of L/R is:
View SolutionThe resistance between ends will be (ifρLπR2=R0)
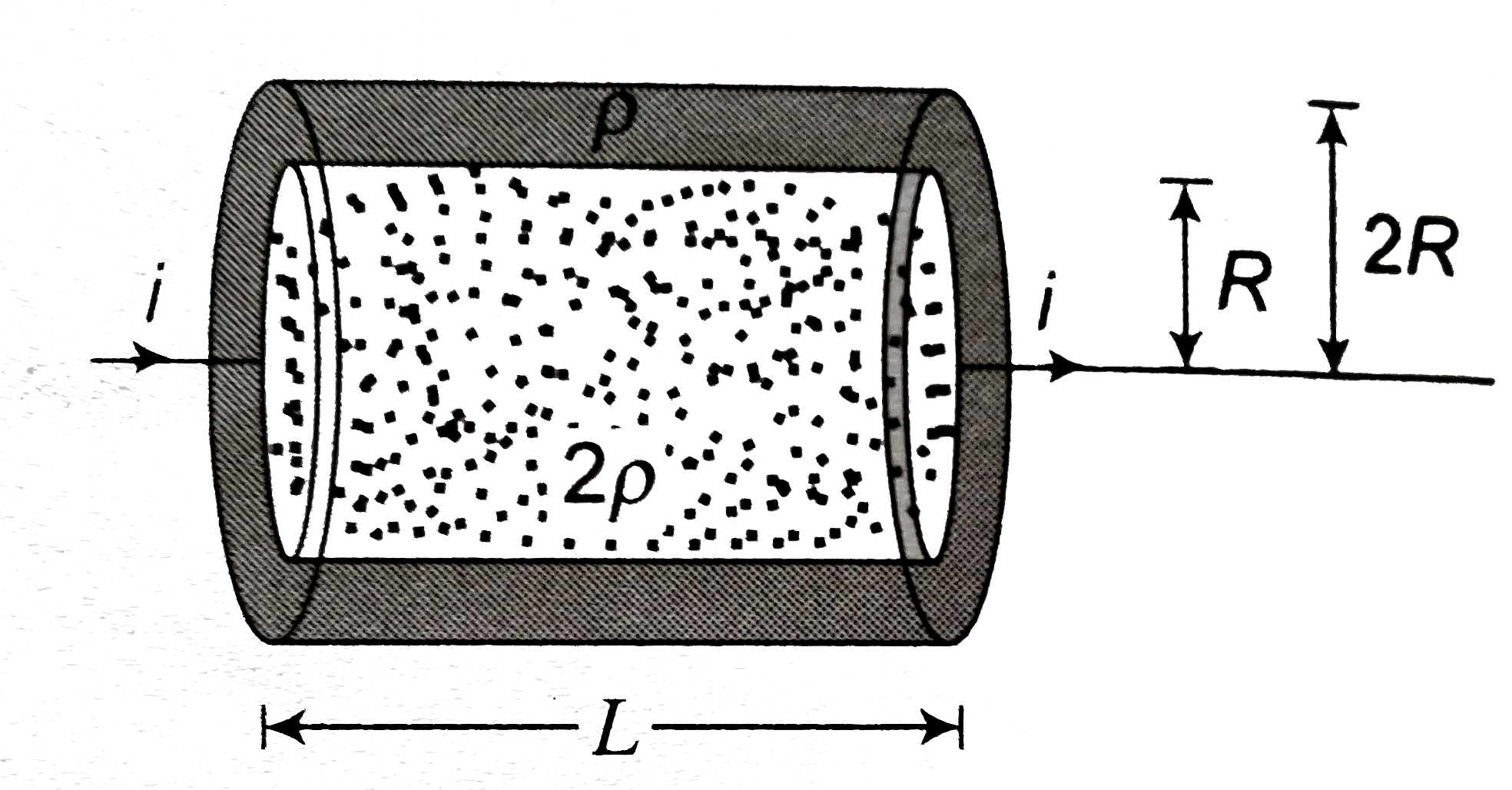 .
.
View SolutionA uniform wire of length l and radius r has a resistance of 100Ω. It is recast into wire of radius R2
View SolutionA wire of length l=6±0.06cm and radius r=0.5±0.005cm and m=0.3±0.003g. Maximum percentage error in density is:
View SolutionA wire of length l=6±0.06cm and radius r=0.5±0.005cm and mass m=0.3±0.003g. Maximum percentage error in density is :
View Solution
