The string of a pendulum is long. The bob is pulled sideways so that the string becomes horizontal and then the bob is released. What is the speed with which the bob arrives at the lowest point ? Assume theat of initial energy is dissipated against air resistance. Take
To find the speed of the pendulum bob at the lowest point after being released from a horizontal position, we can follow these steps:
Step 1: Understand the Initial Energy
When the bob is pulled to the horizontal position, it has potential energy due to its height. The height (h) from which the bob is released is equal to the length of the string (L) since it is horizontal.
- Initial Potential Energy (PE_initial) = mgh
- Here, h = L = 2.0 m, and g = 10 m/s².
Step 2: Calculate Initial Potential Energy
Using the formula for potential energy:
PE_initial=m⋅g⋅h=m⋅10⋅2=20mJoules
Step 3: Account for Energy Loss
It is given that 10% of the initial energy is dissipated due to air resistance. Therefore, only 90% of the initial potential energy will be converted into kinetic energy at the lowest point.
- Energy dissipated = 10% of PE_initial = 0.1 × 20m = 2m Joules
- Remaining Energy (E_final) = 90% of PE_initial = 0.9 × 20m = 18m Joules
Step 4: Relate Remaining Energy to Kinetic Energy
At the lowest point, all the remaining energy is converted into kinetic energy (KE):
KE=12mv2
Setting KE equal to the remaining energy:
12mv2=18m
Step 5: Solve for Speed (v)
We can cancel mass (m) from both sides since it is not zero:
12v2=18
v2=36
v=√36=6m/s
Conclusion
The speed of the bob when it arrives at the lowest point is 6 m/s.
---
When the bob is pulled to the horizontal position, it has potential energy due to its height. The height (h) from which the bob is released is equal to the length of the string (L) since it is horizontal.
- Here, h = L = 2.0 m, and g = 10 m/s².
Using the formula for potential energy:
PE_initial=m⋅g⋅h=m⋅10⋅2=20mJoules
It is given that 10% of the initial energy is dissipated due to air resistance. Therefore, only 90% of the initial potential energy will be converted into kinetic energy at the lowest point.
- Remaining Energy (E_final) = 90% of PE_initial = 0.9 × 20m = 18m Joules
At the lowest point, all the remaining energy is converted into kinetic energy (KE):
KE=12mv2
Setting KE equal to the remaining energy:
12mv2=18m
We can cancel mass (m) from both sides since it is not zero:
12v2=18
v2=36
v=√36=6m/s
The speed of the bob when it arrives at the lowest point is 6 m/s.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBook:PRADEEPChapter:WORK, ENERGY AND POWERExercise:NCERT( Multiple choide questions-1)
Explore 21 Videos
- WORK, ENERGY AND POWERBook:PRADEEPChapter:WORK, ENERGY AND POWERExercise:JEE (Main and Advanced)
Explore 82 Videos
- WORK, ENERGY AND POWERBook:PRADEEPChapter:WORK, ENERGY AND POWERExercise:Fill in the blanks
Explore 10 Videos
- THERMODYNAMICSBook:PRADEEPChapter:THERMODYNAMICSExercise:Assertion- Reason Type Questions
Explore 19 Videos
Similar Questions
The string of a pendulum is 2.0m long. The bob is pulled sideways so that the string becomes horizontal and then the bob is released. What is the speed with which the bob arrives at the lowest point ? Assume theat 10% of initial energy is dissipated against air resistance. Take g=10m/s2
View SolutionThe bob A of a simple pendulum is released from a horizontal position A as shownin in figure. If the length of the pendulum is 1.5m , what is the speed with which the bob arrives at the lowermost point B, given that it dissipates 5% of its initial energy against air resistance ?
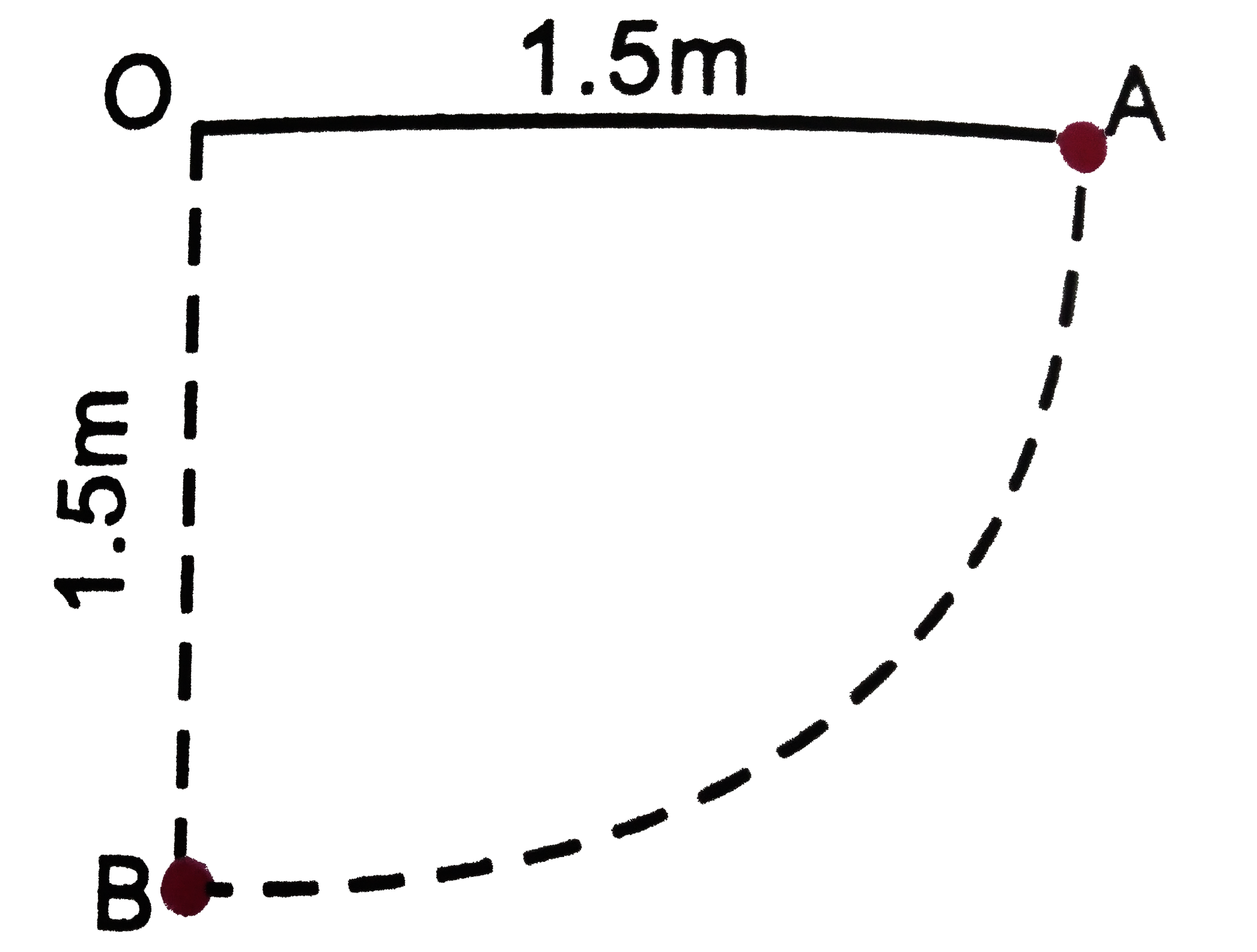
View Solution
Knowledge Check
The bob of a pendulum is released from a horizontal position. If the length of pendulum is 2 m, what is the speed with which the bob arrives at the lower most point. Assume that 10% of its energy is dissipated against air resistance.
(Take g=10ms−2)A4ms−1B6ms−1C8ms−1D10ms−1A pendulum bob on a 2 m string is displaced 60∘ from the vertical and then released. What is the speed of the bob as it passes through the lowest point in its path
A√2m/sB√9.8m/sC4.43m/sD1/√2m/sA pendulum bob on a 2 m string is displaced 60∘ from the verticle and then released . What is the speed of the bob as it passes through the lowest point in its path?
A√2m/sB√9.8m/sC4.43m/sD1/√2m/s
The string of a pendulum is horizontal. The mass of the bob is m. Now the string is released. The tension in the string in the lowest position is -
View SolutionChoose the most appropriate option.
The bob of a pendulum is released from a horizontal position A as shown in the figure. If the length of the pendulumis 1.5 m, what is the speed with which the bob arrives at the lower most point B, given that it dissipated 5% of its initial energy against air resistance?
View SolutionThe bob of a pendulum has its rest point 1 m below the support. The bob is pulled aside until the string makes an angle of 60∘ with the vertical line. On release with what speed does the bob swing pass its rest point?
View SolutionThe bob of a simple pendulum of length L, is displaced through 90∘, from its mean position and then released. What will be the tension in the string when the bob of mass m will be its lowest position ?
View SolutionThe bob of a simple pendulum of length L, is displaced through 90∘, from its mean position and then released. What will be the tension in the string when the bob of mass m will be at its lowest position?
View Solution