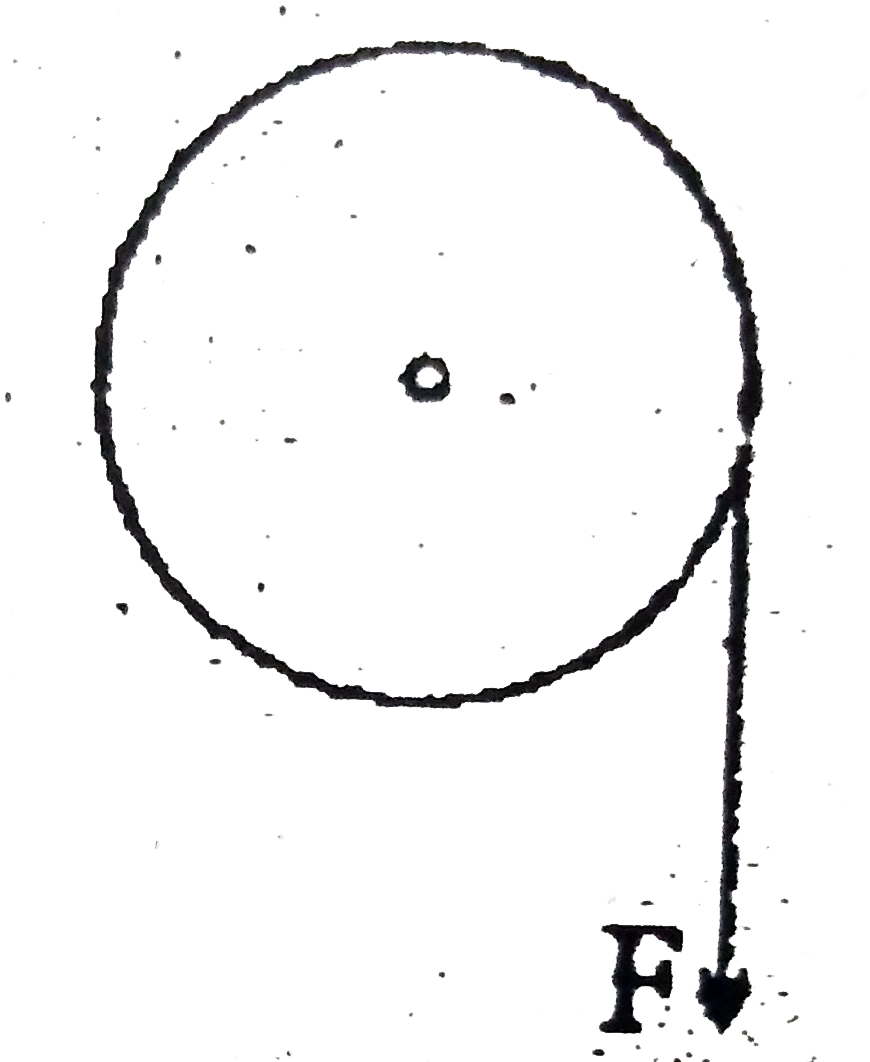
The top is figure has moment of inertia equal to and is initially at rest. It is free to rotate about the stationary axis . A string wrapped around a ped alonng the axis of the top is pulled in such a manners as to maintain a constant tension of .If the string does not slip while it is unwound from the peg, what is the angular speed of the top after of string has been pulled of the peg?

Work done
and work
(The last term is zero because the top starts from rest.)
Thus,
and from this
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Solved Examples
12 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.1
11 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICSCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
The toy-top (initially at rest) shown in the figure, has a moment of inertia 4×10−4kg m2 and it is free to rotate about the stationary axis AA'. The string wrapped around the peg of the toy-top is pulled outwards without letting it slip and simultaneously maintaining a constant tension of 5.625N. If ω is the angular speed (in rad s−1) of the top after 80 cm of string has been pulled of the peg, then mark your answer as ω100.

View SolutionSolve the previous problem if the pulley has a moment of inertia I about its axis and the string does not slip over it.
View Solution
Knowledge Check
The top in figure has a moment of inertia of 4.00×10−4kg.m2 and is initially at rest. It is free to rotate about the stationary axis ∀'. A string, wrapped around a peg along the axis of the top, is pulled in such a manner as to maintain a constant tension of 2.5N.If the string does not slip while it is unwound from the peg. What the angular speed of the top after 80.0cm of string has been pulled off the peg ?
 .A75rad/sB50rad/sC125rad/sD100rad/s
.A75rad/sB50rad/sC125rad/sD100rad/sA flywheel of moment of inertia 0.4 kg m2 and radius 0.2 m is free to rotate about a central axis. If a string is wrapped around it and it is pulled with a force of 10N. Then its angular velocity after 4s will be
A10rads−1B5rads−1C20rads−1DNoneA uniform cylinder free to turn around a fixed axis has a string wrapped around it as shown. The string is pulled with a force F equal to the cylinders weight. What is the acceleration of the string?
 A2gBgCg/2Dg/4
A2gBgCg/2Dg/4
A string is wrapped around a log of wood and it is pulled with a force F as shown in
(b) How does the value of tension T in the string change with θ when will the tension T be greater than applied force?
View SolutionA wheel of moment of inertia I and rdius r is free to rotate about its centre as shown in figure. A string is wrapped over its rim and a block of mass m is attached to the free end of the string. The system is released from rest. Find the speed of the block as it descends through a height h.

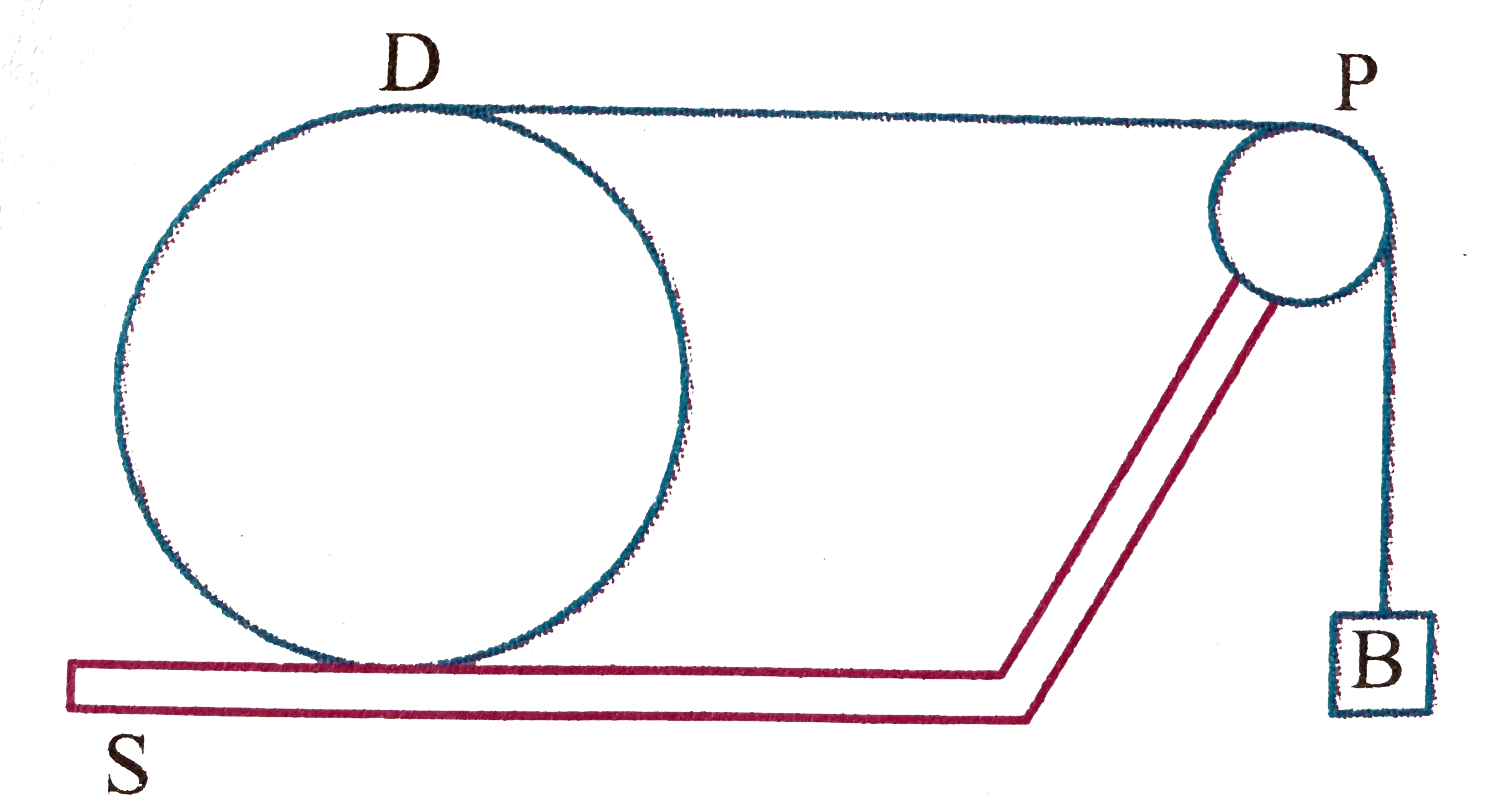
View SolutionIn the figure, the disc D does not slip on the surface S, the pulley P has mass and the string does not slip on it. The string is wound around the disc.
View SolutionA string is wrapped around a cylinder of mass M and radius R. The string is pulled vertically upwards to prevent the centre of mass from falling as the cylinder unwinds the string. The tension in the string is
View SolutionA string is wrapped around a cylinder of mass M and radius R. The string is pulled vertically upwards to prevent the centre of mass from falling as the cylinder unwinds the string. The tension in the string is
View Solution
