
The total force acting on an object as a function of time is given in the graph above. What is the magnitude of the change in momentum of the object between t=0 and t=0.4 sec?
2kg⋅m/s
5kg⋅m/s
10kg⋅m/s
15kg⋅m/s
The correct Answer is:D
The impulse-momentum theorem says that the change in momentum is equal to the impulse, which is the area under the force versus time graph. The region under the graph from t=0 to t=0.4 s is composed of a right triangle (from t=0 to t=0.2s) plus a rectangle (from t=0.2 s to t=0.4s), so the total area under the graph from t==0 to t=0.4 s is (1/2)(0.2)(100)+(0.4-0.2)(25)=10+5=15kg⋅m/s.
Topper's Solved these Questions
Similar Questions
A force F=(2tˆi+3t2ˆj)N acts on an object moving in XY-plane. Find the magnitude of change in momentum of the object in the time interval t=0 to t=2s.
View SolutionA force F=(2tˆi+3t2ˆj)N acts on an object moving in XY-plane. Find the magnitude of change in momentum of the object in the time interval t=0 to t=2s.
View SolutionA 0.25 kg puck is initially stationary on an ice surface with negligible friction. At time t = 0, a horizontal force begins to move the puck. The force is given by →F=(12.0−3.00t2)ˆi, with →F in newtons and t in seconds, and it acts until its magnitude is zero. (a) What is the magnitude of the impulse on the puck from the force between t= 0.750 s and t = 1.25 s? (b) What is the change in momentum of the puck between t=0 and the instant at which F=0?
View SolutionThe force acting on a particle of mass 20 kg is indicated by force - time graph as shown in figure. The magnitude of change in linear momentum of particle during time interval 0 to 6 second is
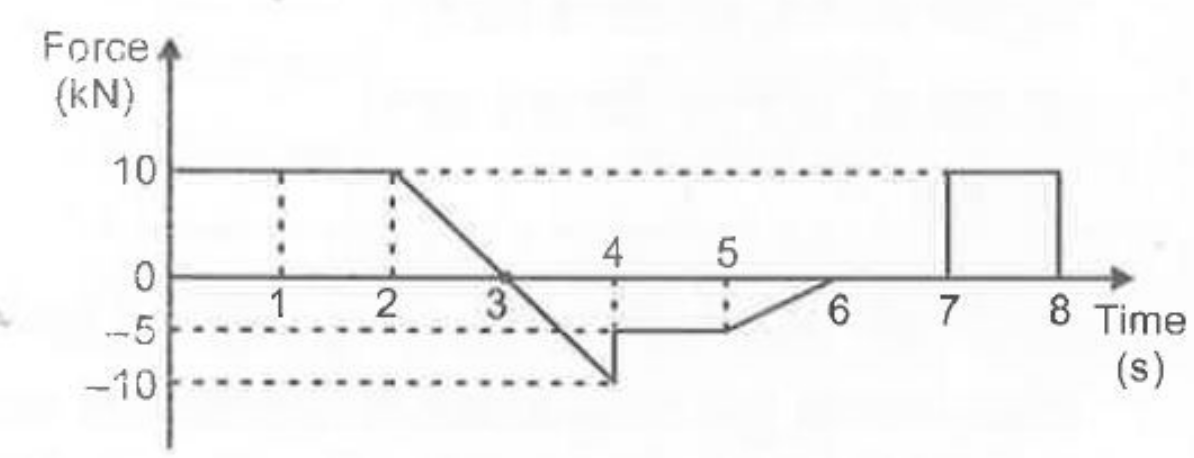
View SolutionThe force acting on a particle of mass 20 kg is indicated by force - time graph as shown in figure. The magnitude of change in linear momentum of particle during time interval 0 to 6 second is

View SolutionThe displacement x of an object is given as a function of time x=2t+3t2
Calculate the instantaneous velocity of the object at t = 2 s
View SolutionThe displacement x of an object is given as a function of time x=2t+3t2
Calculate the instantaneous velocity of the object at t = 2 s
View SolutionThe force(F) acting on a particle of mass m changing with time(t) is as shown in (F−t) graph. The change in linear momentum from t=0 to t=6 s (in kN s) is

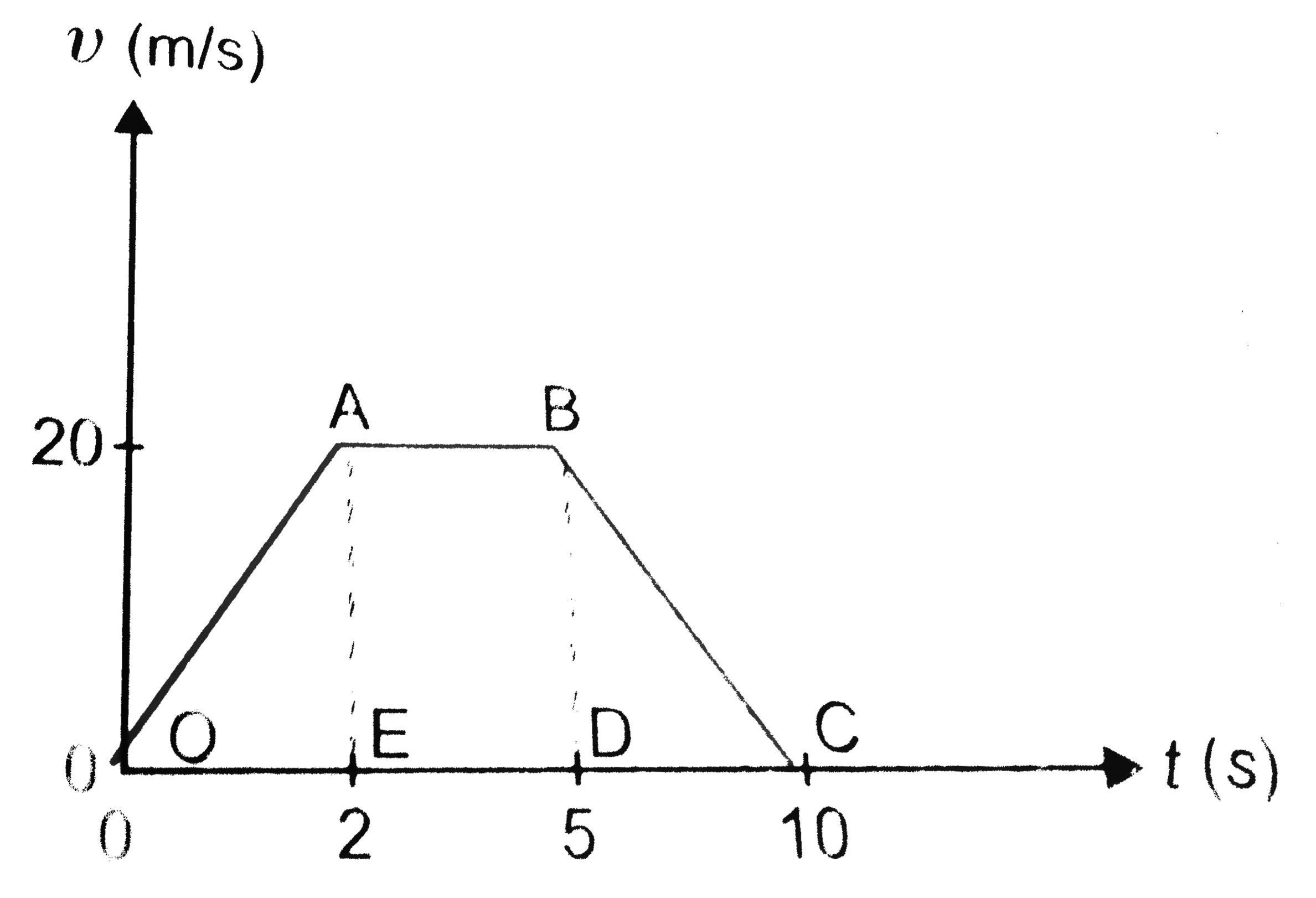
View SolutionThe velocity (u)-time (t) graph of an object moving along a straight line is as shown is Fig. 2 (b) . 30. Calculate the distance covered by object between (i)t=0→t=5s (ii) t=0→t=10s.
 .
.
View SolutionThe velocity (u)-time (t) graph of an object moving along a straight line is as shown is Fig. 2 (b) . 30. Calculate the distance covered by object between (i)t=0→t=5s (ii) t=0→t=10s.
 .
.
View Solution
