The work done by a fielder when he takes a catch in a cricket match is negative. Explain
To explain why the work done by a fielder when he takes a catch in a cricket match is negative, we can follow these steps:
Step 1: Understand the Work Done Formula
The work done (W) is calculated using the formula:
W=F×d×cos(θ)
where:
- W = work done
- F = force applied
- d = displacement of the object
- θ = angle between the force and the displacement direction
Step 2: Analyze the Situation
When a fielder catches a ball, the ball is moving towards him. To catch the ball, the fielder exerts a force in the opposite direction to the motion of the ball.
Step 3: Determine the Angle θ
In this case:
- The displacement of the ball is in one direction (let's say towards the fielder).
- The force exerted by the fielder is in the opposite direction (away from the ball).
Thus, the angle θ between the force exerted by the fielder and the direction of the ball's displacement is:
θ=180∘
Step 4: Calculate the Cosine of the Angle
Using the cosine function:
cos(180∘)=−1
Step 5: Substitute Values into the Work Done Formula
Now substituting θ into the work done formula:
W=F×d×cos(180∘)
W=F×d×(−1)
W=−F×d
Step 6: Conclusion
Since the work done is negative, it indicates that the force exerted by the fielder is in the opposite direction to the displacement of the ball. This negative work done signifies that the fielder is taking energy out of the ball (slowing it down) as he catches it.
Final Answer
Therefore, the work done by a fielder when he takes a catch is negative because he applies a force in the opposite direction to the motion of the ball, resulting in a negative value for work.
---
The work done (W) is calculated using the formula:
W=F×d×cos(θ)
where:
- W = work done
- F = force applied
- d = displacement of the object
- θ = angle between the force and the displacement direction
When a fielder catches a ball, the ball is moving towards him. To catch the ball, the fielder exerts a force in the opposite direction to the motion of the ball.
In this case:
- The displacement of the ball is in one direction (let's say towards the fielder).
- The force exerted by the fielder is in the opposite direction (away from the ball).
θ=180∘
Using the cosine function:
cos(180∘)=−1
Now substituting θ into the work done formula:
W=F×d×cos(180∘)
W=F×d×(−1)
W=−F×d
Since the work done is negative, it indicates that the force exerted by the fielder is in the opposite direction to the displacement of the ball. This negative work done signifies that the fielder is taking energy out of the ball (slowing it down) as he catches it.
Therefore, the work done by a fielder when he takes a catch is negative because he applies a force in the opposite direction to the motion of the ball, resulting in a negative value for work.
Topper's Solved these Questions
- WORK ENERGY AND POWERBOOK - ICSECHAPTER - WORK ENERGY AND POWEREXERCISE - EXERCISE-2(A) (MULTIPLE CHOICE TYPE )
2 Videos
- WORK ENERGY AND POWERBOOK - ICSECHAPTER - WORK ENERGY AND POWEREXERCISE - EXERCISE-2(A) (NUMERICALS)
16 Videos
- WORK ENERGY AND POWERBOOK - ICSECHAPTER - WORK ENERGY AND POWEREXERCISE - EXERCISE-2(C ) NUMERICALS
6 Videos
- CALORIMETRYBOOK - ICSECHAPTER - CALORIMETRYEXERCISE - EXERCISE-11(B) (NUMERICALS)
15 Videos
Similar Questions
Knowledge Check
When is the work - done by a force is positive and when is it negative ?
Adistance is positiveBmaximum distance travelled along direction of force appliedCdisplacement is in direction of force appliedDdistance is negative
How is work done by a force measured when the force :
is in the direction of displacement.
View SolutionHow is work done by a force measured when the force :
is at an angle of displacement
View SolutionWhat is the work done by a force when the force is:
Normal to the displacement produced.
View SolutionA plank of mass M and length L is placed at rest on a smooth horizontal surface. A small block of mass m is projected with a velocity v0 from the left end of it as shown in figure. The coefficient of friction between the block and the plank is m, and its value is such that the block becomes stationary with respect to the plank before it reaches the other end.
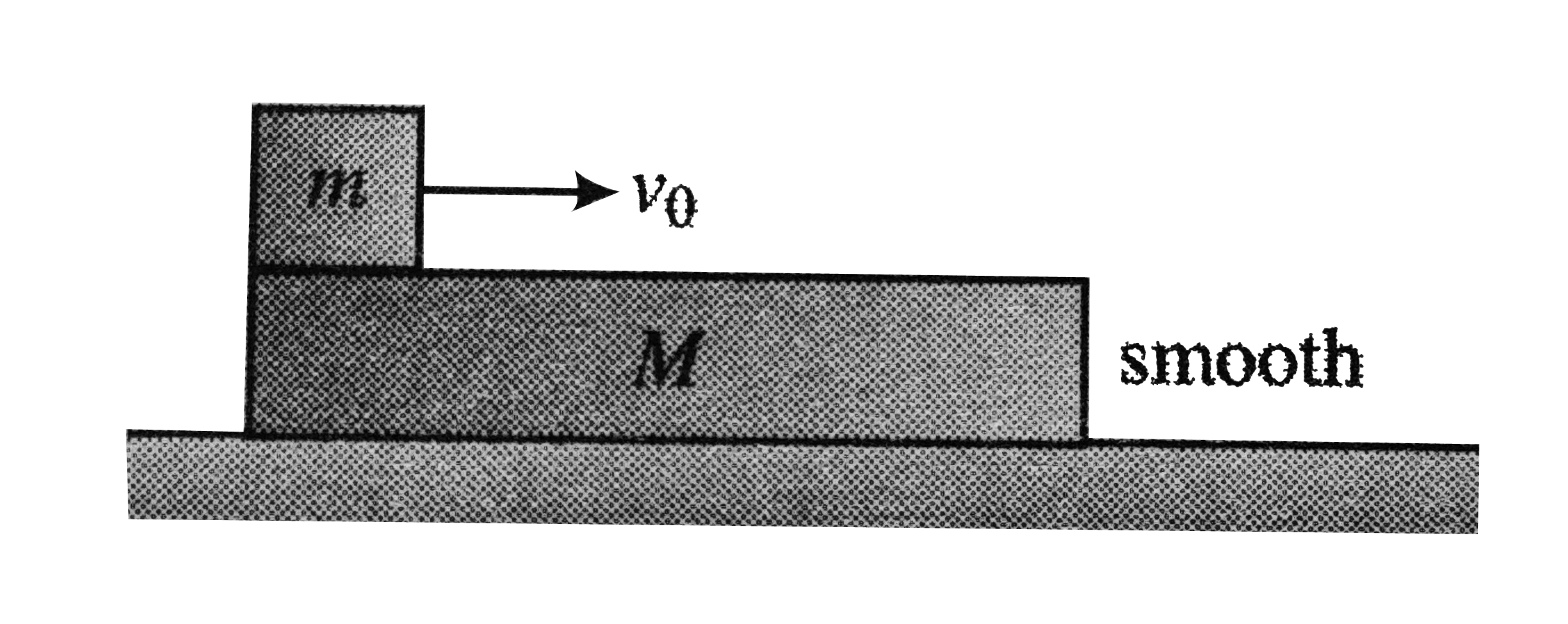
a. Find the time and common velocity when relative sliding between the block and the plank stops.
b. Find the work done by the friction force on the block during the period it slides on the plank. Is the work positive or negative?
c. Calculate the work done on the plank during the same period. Is the work positive or negative?
d. Also, determine the net work done by friction, Is it positive or negative?
View SolutionHow is the work done by a force measured when (i) force is in the direction of displacement, (ii) force is at an angle to the direction of displacement ?
View SolutionWhen is the work done by a force is positive and when is it negative ?
View SolutionWhen the work is done by the system, w is taken as ......
View Solution
