There is a fixed sphere of radius R having positive charge Q uniformly spread in its volume. A small particle having mass m and negative charge (–q) moves with speed V when it is far away from the sphere. The impact parameter (i.e., distance between the centre of the sphere and line of initial velocity of the particle) is b. As the particle passes by the sphere, its path gets deflected due to electrostatics interaction with the sphere.
(a) Assuming that the charge on the particle does not cause any effect on distribution of charge on the sphere, calculate the minimum impact parameter b0 that allows the particle to miss the sphere. Write the value of b0 in term of R for the case
12mV2=100.(kQqR)
(b) Now assume that the positively charged sphere moves with speed V through a space which is filled with small particles of mass m and charge – q. The small particles are at rest and their number density is n [i.e., number of particles per unit volume of space is n].
The particles hit the sphere and stick to it. Calculate the rate at which the sphere starts losing its positive charge dQdt. Express your answer in terms of b0
![There is a fixed sphere of radius R having positive charge Q uniformly spread in its volume. A small particle having mass m and negative charge (– q) moves with speed V when it is far away from the sphere. The impact parameter (i.e., distance between the centre of the sphere and line of initial velocity of the particle) is b. As the particle passes by the sphere, its path gets deflected due to electrostatics interaction with the sphere. (a) Assuming that the charge on the particle does not cause any effect on distribution of charge on the sphere, calculate the minimum impact parameter b0 that allows the particle to miss the sphere. Write the value of b(0) in term of R for the case 1/2mV^(2)=100.((kQq)/(R)) (b) Now assume that the positively charged sphere moves with speed V through a space which is filled with small particles of mass m and charge – q. The small particles are at rest and their number density is n [i.e., number of particles per unit volume of space is n]. The particles hit the sphere and stick to it. Calculate the rate at which the sphere starts losing its positive charge (dQ)/(dt). Express your answer in terms of b(0)](https://d10lpgp6xz60nq.cloudfront.net/physics_images/IJA_PHY_V02_C09_E01_029_Q01.png)
The correct Answer is:(a)b0=R√1+2KQqmRV2 b0≅1.02R<br>(b) qnpib_(0)^(2)VB
Step by step video, text & image solution for There is a fixed sphere of radius R having positive charge Q uniformly spread in its volume. A small particle having mass m and negative charge (– q) moves with speed V when it is far away from the sphere. The impact parameter (i.e., distance between the centre of the sphere and line of initial velocity of the particle) is b. As the particle passes by the sphere, its path gets deflected due to electrostatics interaction with the sphere. (a) Assuming that the charge on the particle does not cause any effect on distribution of charge on the sphere, calculate the minimum impact parameter b0 that allows the particle to miss the sphere. Write the value of b_(0) in term of R for the case 1/2mV^(2)=100.((kQq)/(R)) (b) Now assume that the positively charged sphere moves with speed V through a space which is filled with small particles of mass m and charge – q. The small particles are at rest and their number density is n [i.e., number of particles per unit volume of space is n]. The particles hit the sphere and stick to it. Calculate the rate at which the sphere starts losing its positive charge (dQ)/(dt). Express your answer in terms of b_(0) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ARIHANTCHAPTER - MOTION OF CHARGE IN MAGNETIC FIELDEXERCISE - Level-213videos
MOTION OF CHARGE IN MAGNETIC FIELD
- BOOK - ARIHANTCHAPTER - MAGNETIC EFFECT OF CURRENTEXERCISE - Magnetic effect of Current45videos
MAGNETIC EFFECT OF CURRENT
- BOOK - ARIHANTCHAPTER - NUCLEAR PHYSICSEXERCISE - All Questions54videos
NUCLEAR PHYSICS
Similar Questions
A source(s) of electrons is kept at origin of the co- ordinate system and it shoots out electrons in xy plane with very small range of velocities. In the region x = 0 to x = d there is a uniform electric field −−→E=−Eˆi which accelerates the electrons to speeds much larger than their original speed when they are ejected by the source. The electrons emerge from the field region, beyond x = d, travelling in straight lines. Prove that paths of the electrons, after they emerge out of the field, appear to be diverging from a point P having co-ordinates (–d,0).
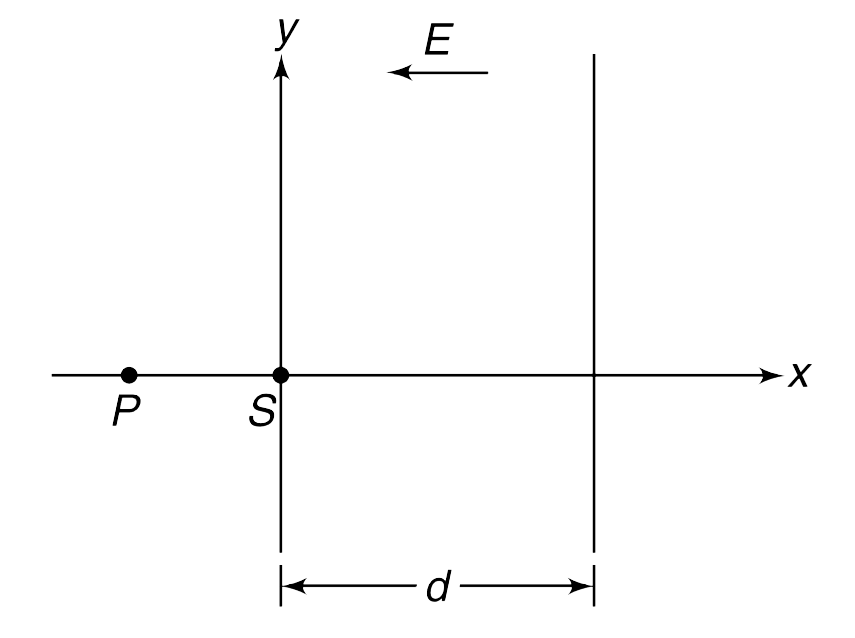03:30View SolutionSketch the magnetic field lines in xy plane for a pair of long parallel wires laid along z direction if-
(a) Both wires carry current in same direction.
(b) Both wire carry current in opposite directions.05:43View SolutionAssume that an electron and a position pair is formed from a gamma ray photon having energy 3.0 MeV. Find the total kinetic energy of the positron and the electron that is formed.
03:52View SolutionA smooth chute is made in a dielectric sphere of radius R and uniform volume charge density. rho . A charge particle of mass m and charge -q is placed at the centre of the sphere. Find the time period of motion of the particle?
04:57View SolutionA positive charge Q is uniformly distributed throughout the volume of a dielectric sphere of radius R. A point mass having charge +q and mass m is fired toward the center of the sphere with velocity v from a point at distance r(r>R) from the center of the sphere. Find the minimum velocity v so that it can penetrate (R/2) distance of the sphere. Neglect any resistance other than electric interaction. Charge on the small mass remains constant throughout the motion.
03:13View SolutionA charged particle having a positive charge q approaches a grounded metallic spheres of raidus R with a constant small speed v as shown in figure. In this situation
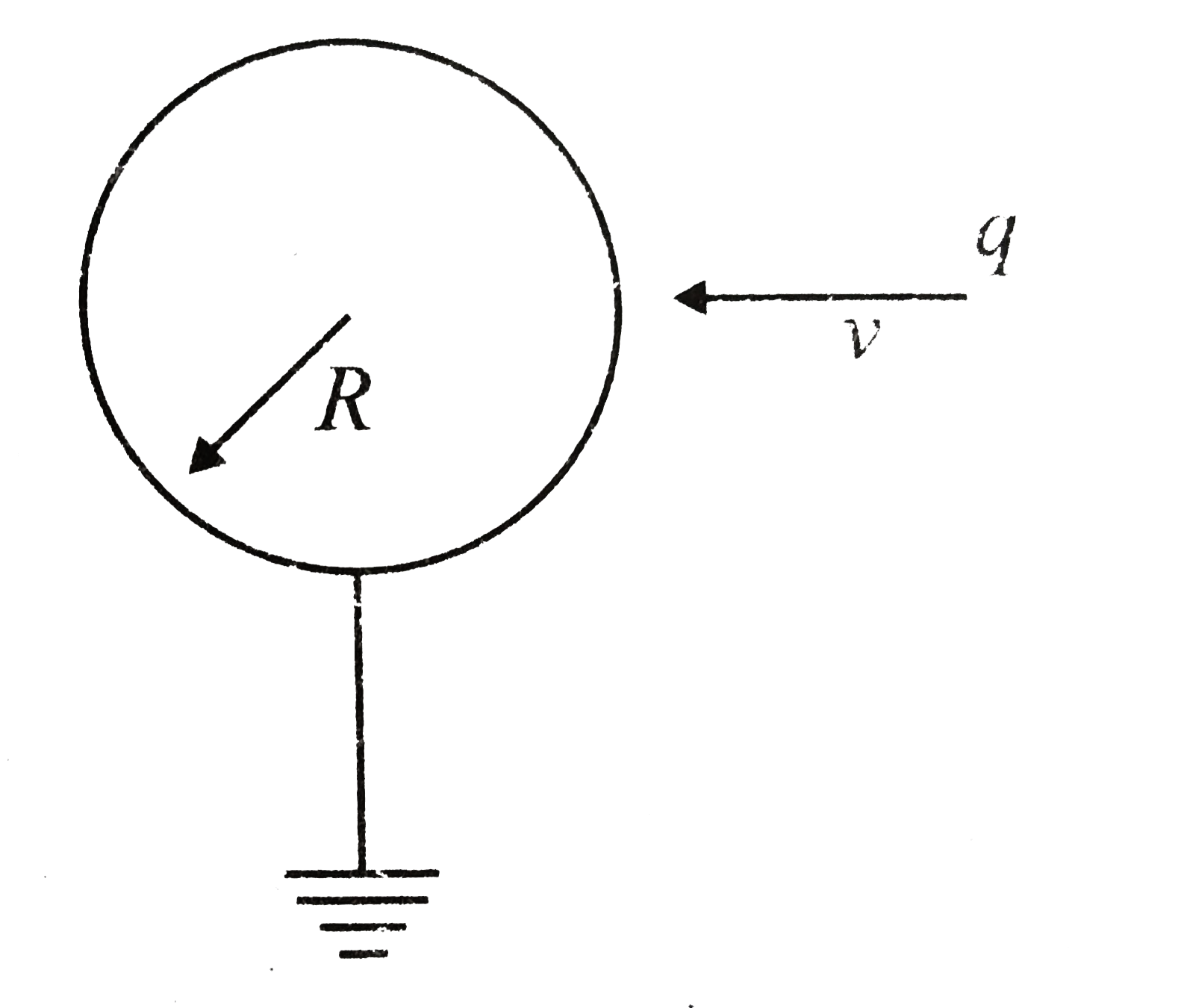03:21View SolutionA large solid sphere with uniformly distributed positive charge has a smooth narrow tunnel along its direction. A small particle with negative charge, initially at rest far from the sphere, approaches it along the line of the tunnel, reaches its surface with a speed v, and passes through the tunnel. Its speed at the centre of the sphere will be
02:27View SolutionA particle A of mass m and charge Q moves directly towards a fixed particle B , which has charge Q . The speed of A is a v when it is far away from B . The minimum separtion between the particles is not proportional to
08:01View SolutionA uniformly charged sphere has radius R and charge Q on it. A negatively charged particle having mass m and charge – q shoots out of the surface of the sphere with speed V. The minimum speed (V0) for which the particle escapes the attraction of the sphere may be called as escape speed. Will the value of V0 depend on magnitude of q? [Recall that escape speed of a body from the surface of the earth does not depend on its mass].
View SolutionA smooth sphere of radius R is moving in a straight line with an acceleration a. A particle is released from top of the sphere from rest. Find the speed of the particle when it is at an angular position θ from the initial position relative to the sphere.
07:15View SolutionA uniformly charged sphere of charge Q and radius R is placed at some height from ground surface and sphere is fixed. Now a charged particle of mass m and charge q is released form rest just below to sphere what will be speed of particle after travelling Y-direction
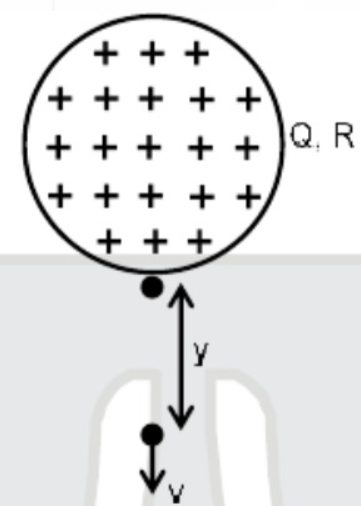02:02View Solutiona large solid sphere with uniformly distributed positive charge has a smooth narrow tunnel through its centre . A small particle with negative charge, initially at far from the sphere, approaches it along the line of the tunnel, reaches its surface with a speed u, and pass through the tunnel . Its speed at the centre of the sphere will be :
View SolutionA uniformly charged sphere of charge Q and radius R is placed at some height from ground surface and sphere is fixed. Now a charged particle of mass m and charge q is released form rest just below to sphere what will be speed of particle after travelling Y-direction
04:45View SolutionA uniformly charged solid sphere a mass M, radius R having charge Q is rotated about its diameter with frequency f0. The magnetic moment of the sphere is
03:28View Solutionएक कण जिसका द्रव्यमान m है एवं आवेश -q है एक समान आवेशित गोले जिसकी त्रिज्या R है एवं कुल आवेश + Q के व्यास के अनुदिश गतिशील है। कण के S.H.M. की आवृति ज्ञात करें यदि आयाम R से अधिक नही है।
03:15View Solution