Three charged particles are initially in position 1, "They are free to move and they come in position" 2 "after some time. Let" U1 and U2 be the electrostatic potential energies in position 1and2. Then
U1>U2
U2>U1
U1=U2
U2≥U1
The correct Answer is:A
Step by step video, text & image solution for Three charged particles are initially in position 1, "They are free to move and they come in position" 2 "after some time. Let" U_(1) and U_(2) be the electrostatic potential energies in position 1 and 2. Then by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced
44 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Comprehension
36 Videos
- ELASTICITYBOOK - DC PANDEYCHAPTER - ELASTICITYEXERCISE - Medical entrances s gallery
21 Videos
- EXPERIMENTSBOOK - DC PANDEYCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
Similar Questions
A particle is moving with SHM in a straight line. When the distance of the particle from the equilibrium position has valuesx1 andx2, the corresponding value of velocities are u1 and x1. Show that the time period of oscillation is given by t = 2....
View SolutionThree charged particles held in fixed positions by forces that are not shown. What is the electric potential energy U of this system of charges? Assume that d = 12 cm and that
q1=+q,q2=4q,andq3=+2q,
in which q=150 n C.
View Solution
Knowledge Check
Three large conducting plates carrying charges Q,2Qand3Q on them, are placed parallel to each other as shown in the figure. If U1andU2 denote electrostatic potential energy in the space between 1st and 2nd plane and 2nd and 3rd plate respectively as indicated, then U2U1 equals
A1B0C2/3D3/2Let ΔU1 and ΔU2 be the changes in internal energy of an ideal gas in the processes A and B then
AΔU1>ΔU2BΔU1=ΔU2CΔU1<ΔU2DΔU1≠ΔU2A charge particle q1, is at position (2, -1, 3). The electrostatic force on another charged particle q2 at (0, 0, 0) is :
Aq1q256π∈0(2ˆi−ˆj+3ˆk)Bq1q256√14π∈0(2ˆi−ˆj+3ˆk)Cq1q256π∈0(ˆj−2ˆi−3ˆk)Dq1q256√14π∈0(ˆj−2ˆi−3ˆk)
(a) The motion of the particle in simple harmonic motion is given by x=asinωt. If its speed is u, when the displacement is x1 and speed is v, when the displacement is x2, show that the amplitude of the motion is
A=[v2x21−u2x22v2−u2]1/2
(b) A particle is moving with simple harmonic motion is a straight line. When the distance of the particle from the equilibrium position has the values x1 and x2 the corresponding values of velocity are u1 and u2, show that the period is
T=2π[x22−x21u21−u22]1/2
View SolutionA body is executing a simple harmonic motion such that its potential energy is U1at U2aty When the displacement is x+y the potential energy will be
View SolutionTrajectories of two projectiles are shown in figure.Let T1 and T2 be the time periods and u1 and u2 their speeds of projection.Then
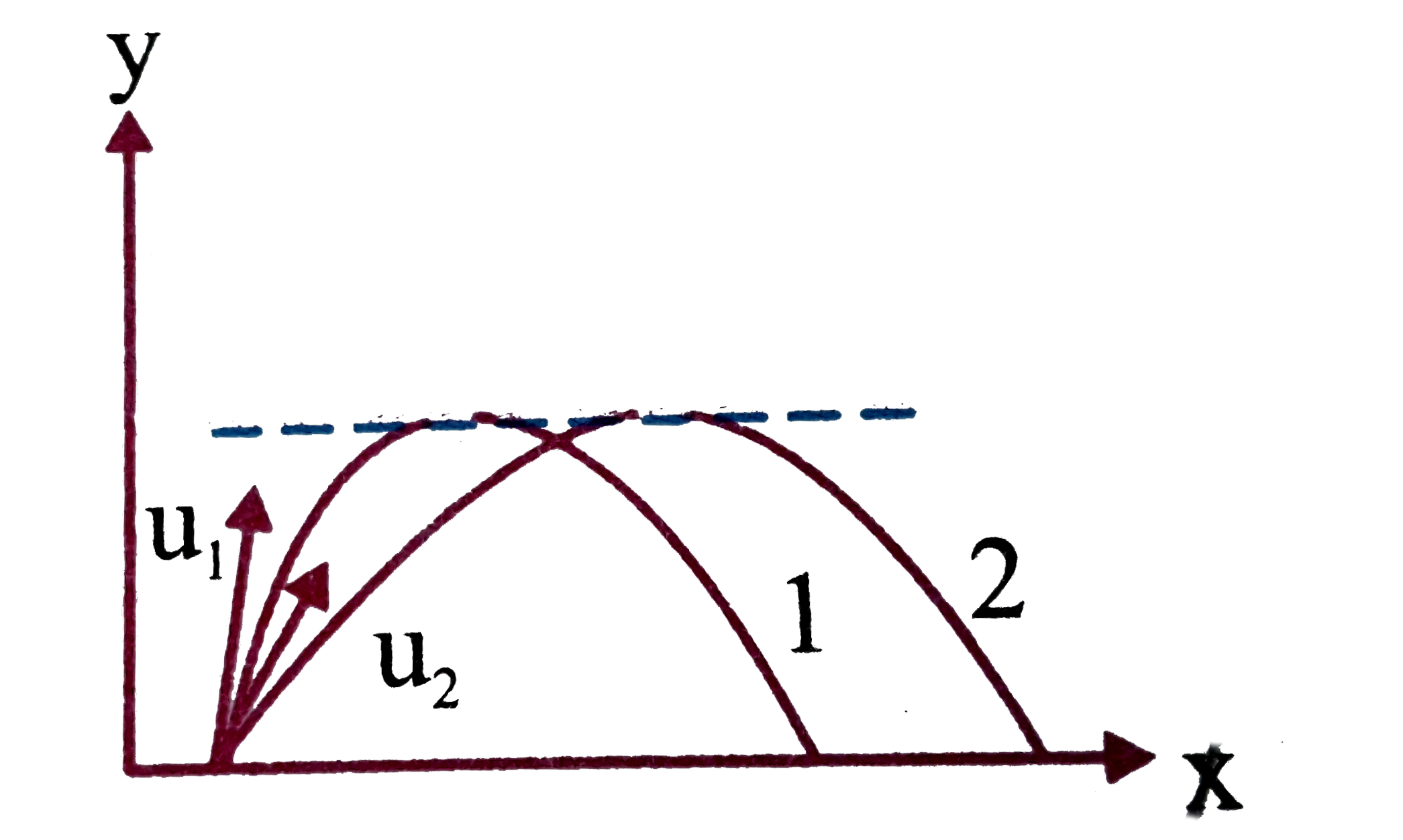
View SolutionTrajectories of two projectiles are shown in the figure. Let T1 and T2 be the time period and u1 & u2 speeds of projection. Then

View SolutionA positive charged particle when moves from potential to lower potential
View Solution
