Three charges q1=1μC,q2=−2μC,andq3=−1μC are placed at A(0,0,0),B(−1,2,3,)andC(2,−1,1). Find the potential of the system of three charges at P(1,−2,−1).
To find the electric potential at point P(1, -2, -1) due to the three charges q1=1μC, q2=−2μC, and q3=−1μC located at points A(0, 0, 0), B(-1, 2, 3), and C(2, -1, 1) respectively, we can follow these steps:
Step 1: Calculate the distances from point P to each charge.
We will use the distance formula in three-dimensional space, which is given by:
r=√(x2−x1)2+(y2−y1)2+(z2−z1)2
Distance r1 from A to P:
Coordinates of A: (0,0,0)
Coordinates of P: (1,−2,−1)
r1=√(1−0)2+(−2−0)2+(−1−0)2=√12+(−2)2+(−1)2=√1+4+1=√6
Distance r2 from B to P:
Coordinates of B: (−1,2,3)
Coordinates of P: (1,−2,−1)
r2=√(1−(−1))2+(−2−2)2+(−1−3)2=√(1+1)2+(−4)2+(−4)2=√22+16+16=√4+32=√36=6
Distance r3 from C to P:
Coordinates of C: (2,−1,1)
Coordinates of P: (1,−2,−1)
r3=√(1−2)2+(−2−(−1))2+(−1−1)2=√(−1)2+(−2+1)2+(−2)2=√1+(−1)2+4=√1+1+4=√6
Step 2: Calculate the electric potential at point P.
The electric potential V due to a point charge is given by:
V=kQr
where k=9×109N m2/C2 is Coulomb's constant.
The total potential at point P due to the three charges is:
VP=V1+V2+V3
Calculating each potential:
1. For q1=1μC:
V1=kq1r1=9×1091×10−6√6=9×103√6
2. For q2=−2μC:
V2=kq2r2=9×109−2×10−66=−3×103
3. For q3=−1μC:
V3=kq3r3=9×109−1×10−6√6=−9×103√6
Step 3: Combine the potentials.
Now, we can sum up the potentials:
VP=9×103√6−3×103−9×103√6
The terms 9×103√6 cancel out:
VP=−3×103V
Final Answer:
The potential at point P(1, -2, -1) is −3000V.
---
We will use the distance formula in three-dimensional space, which is given by:
Coordinates of A: (0,0,0)
Coordinates of P: (1,−2,−1)
Coordinates of B: (−1,2,3)
Coordinates of P: (1,−2,−1)
Coordinates of C: (2,−1,1)
Coordinates of P: (1,−2,−1)
The electric potential V due to a point charge is given by:
V1=kq1r1=9×1091×10−6√6=9×103√6
V2=kq2r2=9×109−2×10−66=−3×103
V3=kq3r3=9×109−1×10−6√6=−9×103√6
Now, we can sum up the potentials:
The potential at point P(1, -2, -1) is −3000V.
Topper's Solved these Questions
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Examples
9 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Exercise 3.1
23 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s
38 Videos
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q
2 Videos
Similar Questions
Three point charge q1=1μC,q2=−2μC and q3=3μC are placed at (1m,0,0),(0,2m,0) and (0,0,3m) respectively. Find the electric potential at as origin.
View SolutionThree charges q1=1μC,q2=−2μC and q3=3μC are placed on the vertices of an equilateral triangle of side 1.0 m. find the net electric force acting on charge q1.
How to proceed Charge q2 will attract charge q1 (along the line joining them) and charge q3 will repel charge q1. Therefore, two forces will act on q1, one due to q2 and another due to q3. Since , the force is a vector quantity both of these force (say F1 and F2) will be added by vector method. Following are two methods of their addition
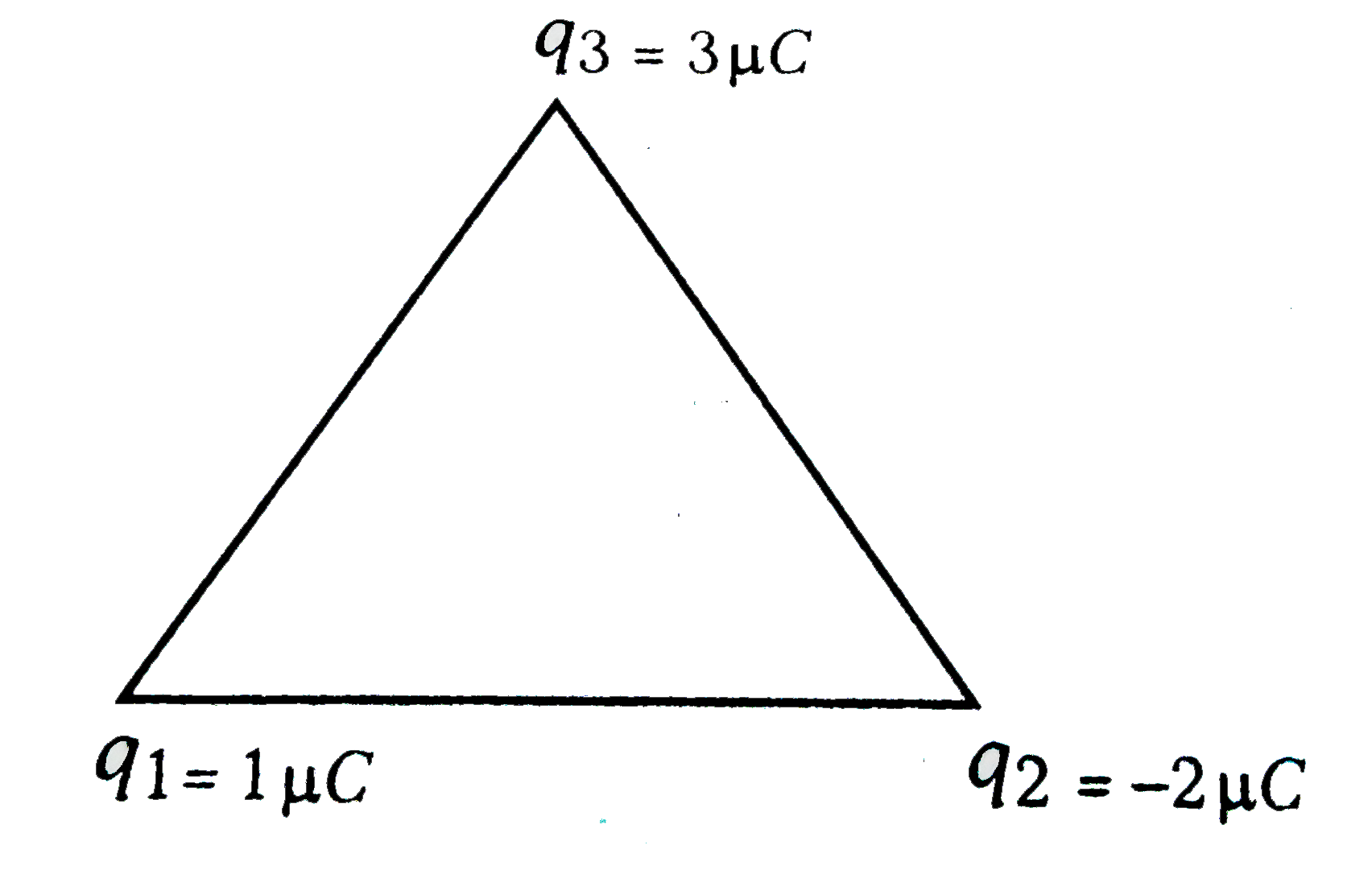
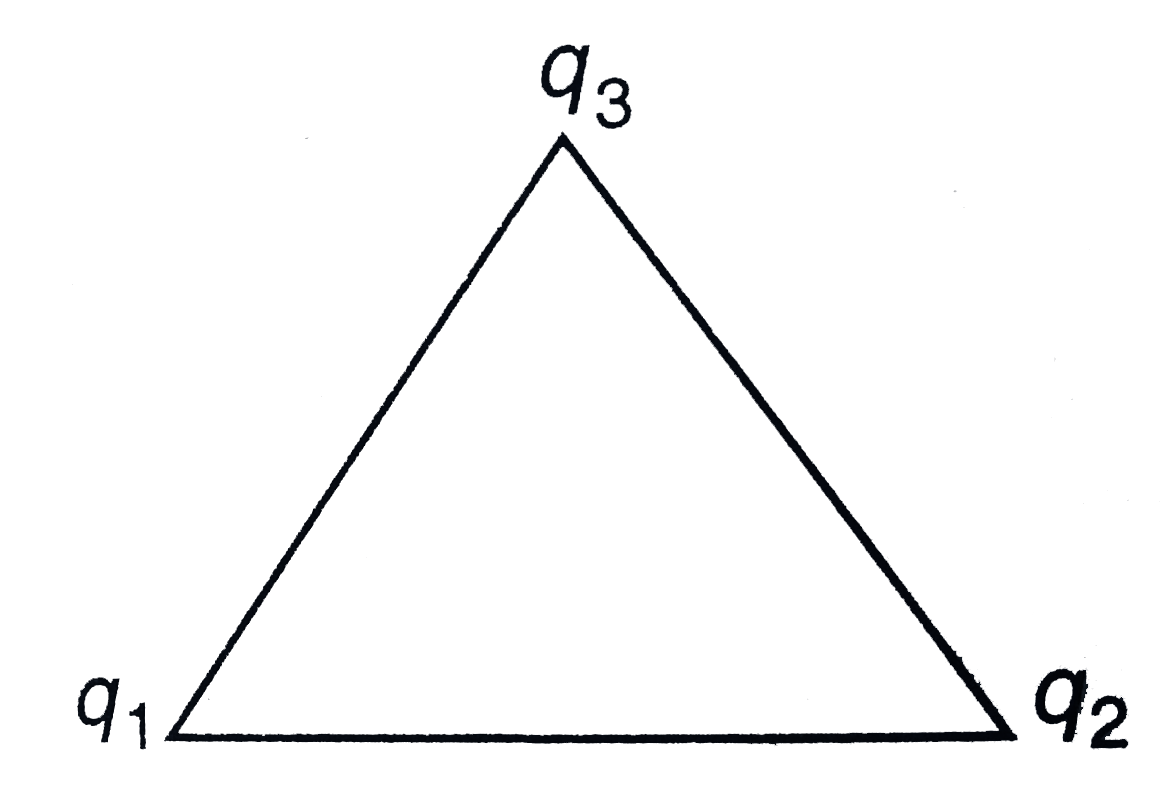
View SolutionThree charges q1=1μC,q2=2μC and q3=3μC are placed on the vertices of an equilateral triangle of side 1.0 m. Find the net electric force acting on charge q1
View SolutionThree charges 2 μC , -4 μC and 8 μC are places at the three vertices of an equilateral triangle of side 10 cm. The potential at the centre of triangle is
View SolutionA charge q1=2.00μC is located at the origin , and a charge q2=−6.00μC is located at (0,3.00)m.
(a) Find the total electric potential due to these charges at the point P hose coordinates are ( 4.00,0) m .
( b) Find the change in potential energy of the system of two charges plus a charge q3=3.00μC as the latter charge moves from infinity to point P .
View SolutionSix charges , q1=+1 μC , q2=+3 μC , q3=+4 μC , q4=−2 μC , q5=−3 μC and q6=−3 μC are placed on a sphee of radius 10 cm. The potential at centre of sphere is
View SolutionIf P(0, 1, 2), Q(4, -2, 1) and R(0, 0, 0) are three
points, then ∠PRQ is
View SolutionPoint charge q1=2μC and q2=−1μC are kept at points x=0 and x=6 respectively. Electrical potential will be zero at points
View SolutionFour point charges qA=2μC,qB=−5μC,qC=2μCandqD=−5μC are located at the corners of a square ABCD of side 10 cm. What is the force on a charge of 1μC placed at the center of the square ?
View SolutionThree charges q1=1×10−6C,q2=2×10−6C,andq3=−3×10−6C have been placed as shown in figure. Then the net electric flux will be maximum for the surface
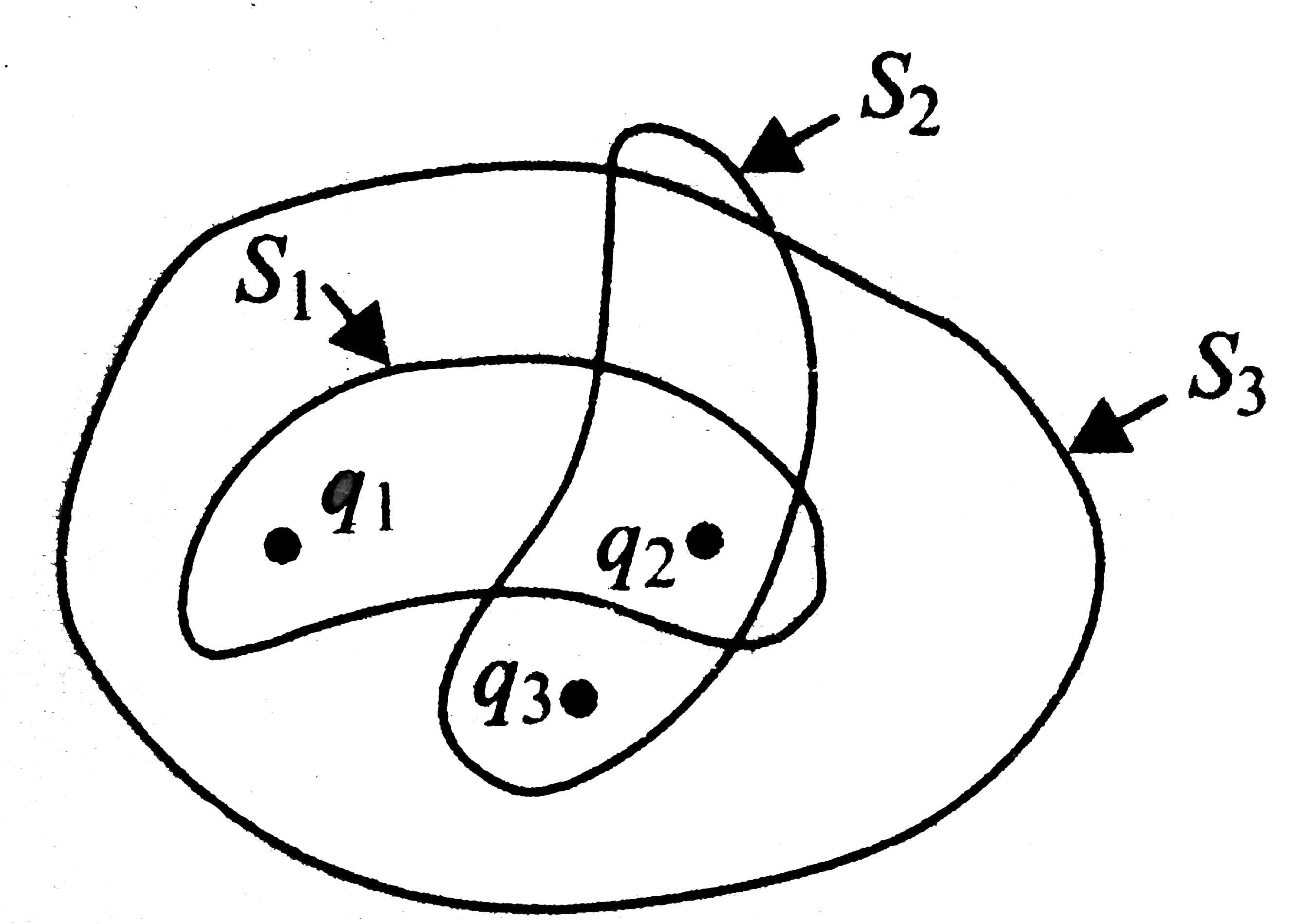
View Solution
