Three particles of masses100 g, 150 g and 200 g are placed at the vertices of an equilateral △ABC of each side 50 cm. Find the centre of mass of the system of three particles.Solution in Punjabi
Step by step video & image solution for Three particles of masses100 g, 150 g and 200 g are placed at the vertices of an equilateral /_\ABC of each side 50 cm. Find the centre of mass of the system of three particles. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- Centre of massBOOK - MODERN PUBLICATIONCHAPTER - Centre of massEXERCISE - Exercise
30 Videos
- Acceleration due to gravityBOOK - MODERN PUBLICATIONCHAPTER - Acceleration due to gravityEXERCISE - Exercise
29 Videos
- Competitive Exam QuestionBOOK - MODERN PUBLICATIONCHAPTER - Competitive Exam QuestionEXERCISE - Exercise
301 Videos
Similar Questions
What is centre of mass of a system?
View SolutionState Keplers' laws of planetary motion.
View SolutionGive the magnitude and direction of the net force acting on:- a drop of rain falling down with a constant speed.
View SolutionFind the centre of mass of three particle at the vertices of an equilateral triangle. The masses of the particle are 100g, 150 g, and 200g respectively. Each side of the equilateral triangle is 0.5m along.
View SolutionFind the centre of mass of three paritcles at the vertices of an equilateral triangle. The masses of the particles are 100 g. 150g and 200 g respectively. Each side of the equilateral triangle is 0.5 m long.
View Solution10 g 20 g तथा 30 g के तीन कण एक समबाहु त्रिभुज ABC के शीर्षो पर इसी क्रम से रखे गए है| त्रिभुज की प्रत्येक भुजा की लम्बाई 1 m है | इस संहति का द्रव्यमान केंद्र निकाले |
View SolutionThree particles each of mass 100 g are placed at the vertices of an equilateral triangle of side length 10 cm. Find the moment of inertia of the system about an axis passing through the centroid of the triangle and perpendicular to its plane.
View SolutionThree balls A,B and C of masses 50 g , 100 g and 150 g respectively are placed at the verticles of an equilateral triangle . The length of each side is 1 m . If A is placed at (0,0) and B is placed at (1,0) m , find the coordinates , (x,y) for the centres of mass of this system of balls
View SolutionThe velocities of three particles of masses 20 g, 30 g and 50 g are 10ˆi, 10ˆj and 10ˆk respectively. The velocity of the centre of mass of the three particles is
View SolutionThree particles of massses 50 g, 100 g and 150 g are placed at the vertices of an equilateral triangle of side 1m ( as shown in the figure ) . The ( x, y) coordinates of the centre of mass will be :
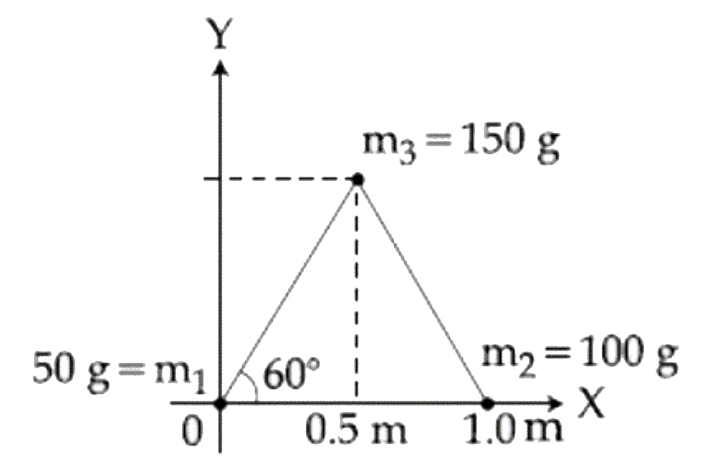
View SolutionThree particles of masses 1 g, 2g, and 3g are placed at the vertices of an equilateral triangle of side 1 m. Locate the centre of mass of the system.
View SolutionFind the centre of mass of three particle at the vertices of an equilateral triangle. The masses of the particle are 100g,150g,and200g respectively. Each side of the equilateral triangle is 0.5m along.
View SolutionThree particles each of mass 100 g are placed at the vertices of an equilateral triangle of side length 10 cm. Find the moment of inertia of the system about an axis passing through the centroid of the triangle and perpendicular to its plane.
View SolutionThe velocities of three particles of masses 20 g, 30 g and 50 g are 10ˆi, 10ˆj and 10ˆk respectively. The velocity of the centre of mass of the three particles is
View SolutionThree particles of massses 50 g, 100 g and 150 g are placed at the vertices of an equilateral triangle of side 1m ( as shown in the figure ) . The ( x, y) coordinates of the centre of mass will be :

View SolutionThree particles of masses 100 g, 150 g and 200.gare placed at the vertices of an equilateral triangle. Each side of the triangle is 0.5 m long. The centre of mass of the three particle is
View Solution
