Three stones are projected simultaneously with same speed u from the top of a tower. Stone 1 is projected horizontally and stone 2 and stone 3 are projected making an angle q with the horizontal as shown in fig. Before stone 3 hits the ground, the distance between 1 and 2 was found to increase at a constant rate u.
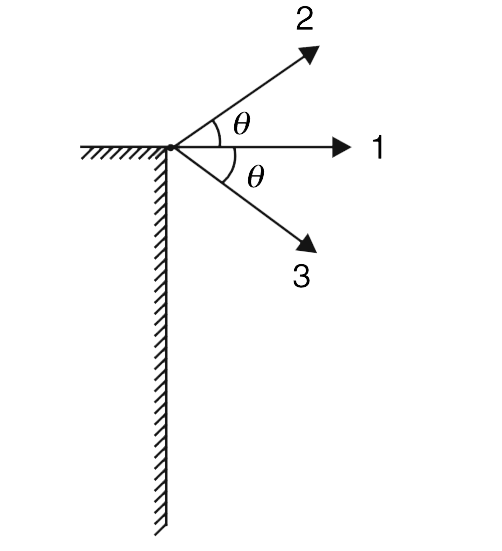
(a) Find θ
(b) Find the rate at which the distance between 2 and 3 increases.
The correct Answer is:(a) θ=60∘ (b) √3u
Topper's Solved these Questions
- BOOK - ARIHANTCHAPTER - KINEMATICSEXERCISE - Level 333videos
KINEMATICS
- BOOK - ARIHANTCHAPTER - KINEMATICSEXERCISE - Level 333videos
KINEMATICS
- BOOK - ARIHANTCHAPTER - HEAT TRANSFEREXERCISE - Heat Transfer45videos
HEAT TRANSFER
- BOOK - ARIHANTCHAPTER - KINETIC THEORY OF GASESEXERCISE - Level 34videos
KINETIC THEORY OF GASES
Similar Questions
Two stones AandB are projected simultaneously from the top of a 100−m high tower. Stone B is projected horizontally with speed 10ms−1, and stone A is droppd from the tower. Find out the following :
(a) Time of flight of the two stone
(b) Distance between two stones after 3s
(c ) Angle of strike with ground
(d) Horizontal range of particle B.View SolutionTwo stones AandB are projected simultaneously from the top of a 100−m high tower. Stone B is projected horizontally with speed 10ms−1, and stone A is droppd from the tower. Find out the following :
(a) Time of flight of the two stone
(b) Distance between two stones after 3s
(c ) Angle of strike with ground
(d) Horizontal range of particle B.View Solution
Knowledge Check
- Question 1 - Select One
Two stones are projected simultaneously from a tower a differenct angles of projection with same speed 'u'. The distance between two stones is increasing at constant rate 'u'. Then the angle between the initial velocity vectors of the two stones is :
A30∘B60∘C45∘D90∘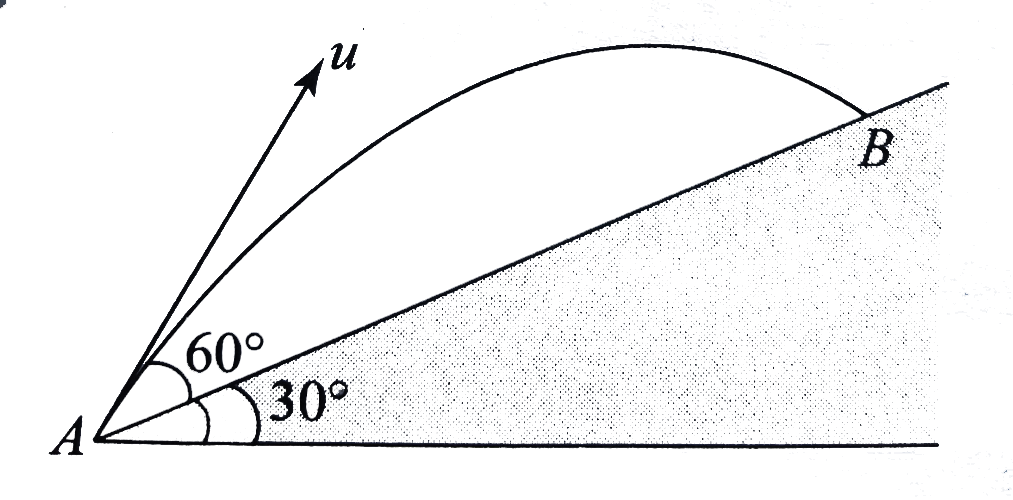 - Question 2 - Select One
A stone is projected from point A with speed u making an angle 60∘ with horizontal as shown. The fixed inclined suface makes an angle 30∘ with horizontal. The stone lands at B after time t. Then the distance AB is equal toAut√3B√32C√3utD2ut - Question 3 - Select One
Two stones are thrown from the top of a tower, one straight down with an initial speed u and the second straight up with the same speed u. When the two stones hit the ground they will have speeds in the ratio.
A2:3B2:1C1:2D1:1
Two stones are projected simultaneously from a tower a differenct angles of projection with same speed 'u'. The distance between two stones is increasing at constant rate 'u'. Then the angle between the initial velocity vectors of the two stones is :
View Solution-
A stone is projected from point A with speed u making an angle 60∘ with horizontal as shown. The fixed inclined suface makes an angle 30∘ with horizontal. The stone lands at B after time t. Then the distance AB is equal toView Solution -
A stone is projected from point A with speed u making an angle 60∘ with horizontal as shown. The fixed inclined suface makes an angle 30∘ with horizontal. The stone lands at B after time t. Then the distance AB is equal toView Solution A stone projected vertically up with velocity v from the top of a tower reaches the ground with velocity 2 v The height of the tower
View SolutionA stone is projected with speed of 50ms−1 at an angle of 60∘ with the horizontal. The speed of the stone at highest point of trajectory is
View Solution