A wall OP is inclined to the horizontal ground at an angle α. Two particles are projected from points A and B on the ground with same speed (u) in directions making an angle θ to the horizontal (see figure). Distance between points A and B is x0=24m. Both particles hit the wall elastically and fall back on the ground. Time of flight (time required to hit the wall and then fall back on to the ground) for particles projected from A and B are 4 s and 2 s respectively. Both the particles strike the wall perpendicularly and at the same location. [In elastic collision, the velocity component of the particle that is perpendicular to the wall gets reversed without change in magnitude]
(a) Calculate maximum height attained by the particle projected from A.
![A wall OP is inclined to the horizontal ground at an angle alpha. Two particles are projected from points A and B on the ground with same speed (u) in directions making an angle theta to the horizontal (see figure). Distance between points A and B is x(0) = 24 m. Both particles hit the wall elastically and fall back on the ground. Time of flight (time required to hit the wall and then fall back on to the ground) for particles projected from A and B are 4 s and 2 s respectively. Both the particles strike the wall perpendicularly and at the same location. [In elastic collision, the velocity component of the particle that is perpendicular to the wall gets reversed without change in magnitude] (a) Calculate maximum height attained by the particle projected from A. (b) Calculate the inclination of the wall to the horizontal (alpha) [g =10 m//s^(2)]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/IJA_PHY_V01_C02_E01_080_Q01.png)
(b) Calculate the inclination of the wall to the horizontal (α)[g=10m/s2]
The correct Answer is:(a) 11.25m (b) tan−(85)
Topper's Solved these Questions
- BOOK - ARIHANTCHAPTER - KINEMATICSEXERCISE - Level 333videos
KINEMATICS
- BOOK - ARIHANTCHAPTER - KINEMATICSEXERCISE - Level 333videos
KINEMATICS
- BOOK - ARIHANTCHAPTER - HEAT TRANSFEREXERCISE - Heat Transfer45videos
HEAT TRANSFER
- BOOK - ARIHANTCHAPTER - KINETIC THEORY OF GASESEXERCISE - Level 34videos
KINETIC THEORY OF GASES
Similar Questions
A car manufacturer usually tells a optimum speed (V0) at which the car should be driven to get maximum mileage. In order to find the optimum speed for a new model, an engineer of the car company experimented a lot and finally plotted a graph between the extreme time t (defined as number of hours a tank full of petrol lasts) vs the constant speed V at which car was run.
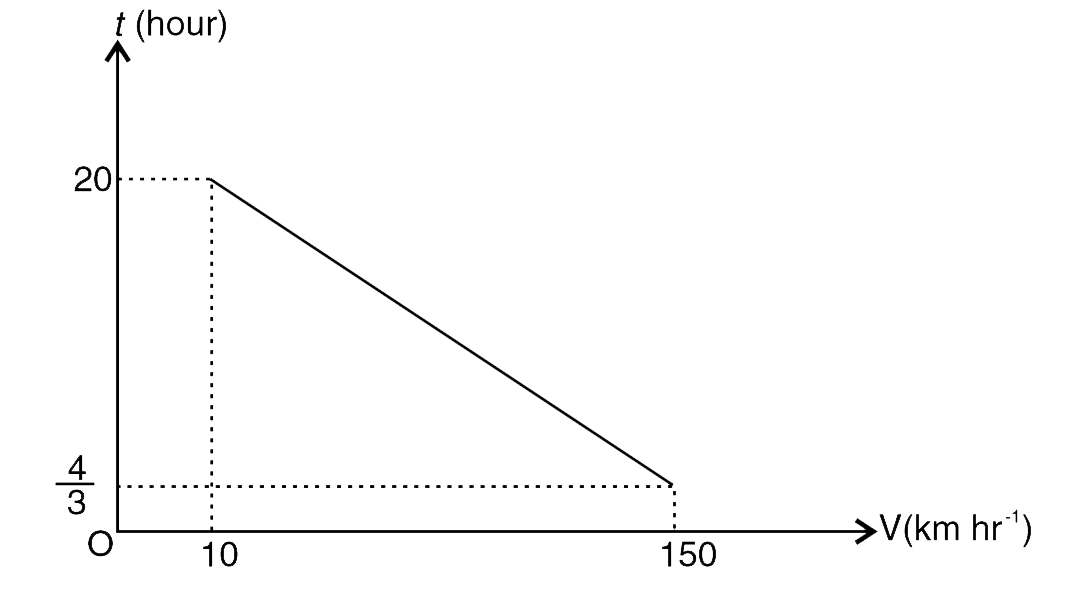
(a) Calculate the optimum speed V0 for this new model.
(b) If the fuel tank capacity of this car is 50 litre, what maximum mileage can be obtained from this car?07:17View SolutionA car manufacturer usually tells a optimum speed (V0) at which the car should be driven to get maximum mileage. In order to find the optimum speed for a new model, an engineer of the car company experimented a lot and finally plotted a graph between the extreme time t (defined as number of hours a tank full of petrol lasts) vs the constant speed V at which car was run.
(a) Calculate the optimum speed V0 for this new model.
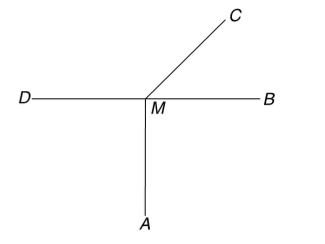
(b) If the fuel tank capacity of this car is 50 litre, what maximum mileage can be obtained from this car?06:27View SolutionFour metal rods each of length L and cross sectional area A are joined at point M. Thermal conductivities of MA, MB and MD are equal and that of MC is thrice that of MA. The end points A, B, C and D are kept in large reservoirs. Heat folws into the junction from B at a rate of P(Js−1) and C from at a rate of 3P. Heat flows out of D at a rate of 5P.
(a) Find the relation between temperatures of points A, B and C.
(b) Find temperature of D if temperature of A and M and TA and TM respectively.
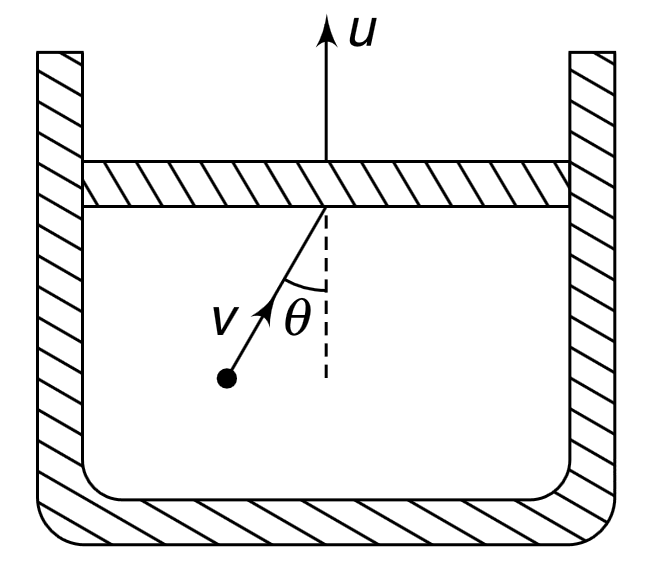
05:28View SolutionAn ideal gas is inside a cylinder with a piston that can move freely. The walls of the cylinder and piston are non- conducting. The piston is being moved out of the cyl- inder at a constant speed u.
(a) Consider a gas molecule of mass m moving with speed v(>>u). It hits the piston elastically at an angle of incidence q. Calculate the loss in kinetic energy of the molecule.
(b) If the area of piston is A and pressure of the gas is P, calculate the rate of decreases of molecular kinetic energy of the gas sample.
(c) Ifu>> molecular velocities, at what rate will the gas lose its molecular kinetic energy
08:58View SolutionA particle at a height h from the ground is projected with an angle 30^@ from the horizontal, it strikes the ground making angle 45^@ with horizontal. It is again projected from the same point with the same speed but with an angle of 60^@ with horizontal. Find the angle it makes with the horizontal when it strikes the ground
04:34View SolutionA particle is projected from the ground with an initial speed of v at an angle θ with horizontal. The average velocity of the particle between its point of projection and highest point of trajectroy is :
00:47View SolutionFind (a) time of flight , (b) Max.height (c ) Horizontal range of projectile projected with speed (v) making an angle θ with the horizontal direction from ground.
02:29View SolutionA particle is thrown with speed u at an angle α with horizontal from the ground. After how much time, the velocity of particle will make an angle β with horizontal.
01:57View SolutionTwo particles A and B are projected simultaneously from a fixed point of the ground. Particle A is projected on a smooth horizontal surface with speed v, while particle B is projected in air with speed v, while particle B is projected in air with speed (2v)/(sqrt(3)) . If particle B hits the particle A, the angle of projection of B with the vertical is
View SolutionA particle is projected from horizontal ground at angle 'θ' with speed 'u'. In same plane of motion a horizontal acceleration 'a' exists so that projected particle returns back to point of projection. Find time of flight.
04:21View SolutionA particle having mass m is projected with a velocity v_(0) from a point P on a horizontal ground making an angle theta with horizontal. Find out the torque about the point of projection acting on the particle when It is just about to hit the ground back ?
View SolutionParticle is projected from the ground at a certain angle with horizontal . If particle takes time T to hit the ground again , then maximum height of the projectile is
View SolutionA particle is projected from the ground with an initial speed of v at a angle θ with horizontal. The average velocity of the particle between its point of projection and highest point of trajectory is
06:18View SolutionTwo particles A and B are projected simultaneously from a fixed point of the ground. Particle A is projected on a smooth horizontal surface with speed v, while particle B is projected in air with speed v, while particle B is projected in air with speed (2v)/(sqrt(3)) . If particle B hits the particle A, the angle of projection of B with the vertical is
06:56View SolutionA particle is projected from the ground with an initial speed v at an angle θ with horizontal. The average velocity of the particle between its point of projection and highest point of trajectory is
06:18View Solution