Three waves from three coherent sources meet at some point. Resultant amplitude of each is A0. Intensity corresponding to A0isI0. Phase difference between first wave and second wave is 60∘. Path difference between first wave and third wave is λ3. The first wave lags behind in phase angle from second and third wave. Find resultant intensity at this point.
The correct Answer is:D
Here, the sources are three. So, we don't have any direct formula for finding the resultant intensity. First we will find the resultant amplitude by vector method and then by the relation I∝A2, we can also find the resulting intensity.
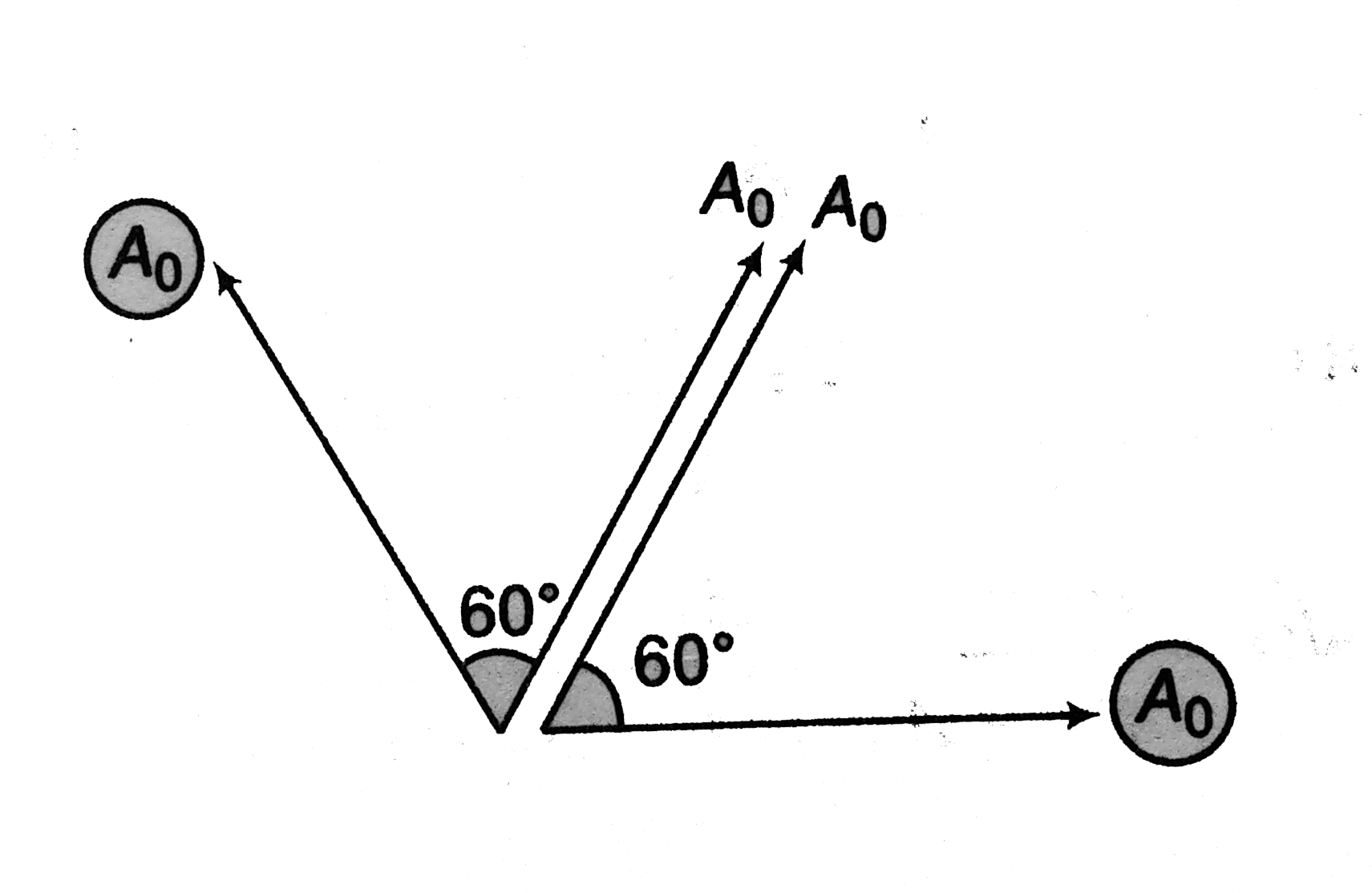
Further, a path difference of λ3 is equivalent to phase difference of 120∘(Δϕorϕ=2πλ.Δx).<br>Hence,thephased⇔erencefirstandsecondis60^(@)andbetweenfirstandthirdis120^(@).So,→−−→rdiagramforamplitudeisasshownbelow.<br> (##DCP_V03_C18_S01_002_S01.png" width="80%">
Now, resultant of first and third acting at 120∘ is also A0 (as A=2A0(cos)ϕ2andϕ=120∘) and
since the first and third are equal, so this resultant A0 passes through the bisector line of these two or in the direction of second amplitude vector. Therefore, the resultant amplitude is
A=A0+A0=2A0
and the resultant intensity is
I=4I0 (as I∝A2).
Topper's Solved these Questions
- SUPERPOSITION OF WAVESBOOK - DC PANDEYCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Example Type 1
1 Videos
- SUPERPOSITION OF WAVESBOOK - DC PANDEYCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Example Type 2
1 Videos
- SOUND WAVESBOOK - DC PANDEYCHAPTER - SOUND WAVESEXERCISE - Exercise 19.7
4 Videos
- THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESBOOK - DC PANDEYCHAPTER - THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESEXERCISE - Medical entrance gallary
30 Videos
Similar Questions
Three waves from three coherent sources meet at some point .
Resultant amplitude of each is A0. Intensity corresponding to A0isI0. Phase
difference between first wave and the second wave is 60∘ . Path difference
between first wave and the third wave is λ3. The first wave lags behind in phase
angle from second and the third wave. Find resultant intensity at this point.
View SolutionThree waves due to three coherent sources meet at one point. Their amplitudes are √2A0,3A0 and √2A0 . Intensity corresponding to A0 is l0. Phasse difference between first and second is 45∘. Path difference between first and third is λ4. In phase angle, first wave lags behind from the other two waves. Find resultant intensity at this point.
View Solution
Knowledge Check
The path difference between two waves meeting at a point is (11/4)λ. The phase difference between the two waves is…
A11 π/4B11π/2C11πD22πFor minimum intensity the phase difference between the two waves is
A2πnB(2n−1)πC(2n+3)πDπnTwo coherent waves of intensities I and 4I interfere at a point. If the resultant intensity is 3I, then the phase difference between te two waves at the point is
AzeroB60∘C120∘D90∘
In interference, two individual amplitudes are A0 each and the
intensity is I0 each. Find resultant amplitude and intensity at a point, where:
(a) phase difference between two waves is 60∘.
(b) path difference between two waves is λ3.
View SolutionIn interference, two individual amplitudes are A0each and the intensity is I0each. Find resultant amplitude and intensity at a point, where
(a) phase difference between two waves is 60∘.
(b) path difference between two waves is λ3.
View SolutionWhat is the phase difference corresponding to path difference λ of two waves reaching a point ?
View SolutionTwo sound waves travel in the same direction in a medium. The amplitude of each wave is A and the phase difference between the two waves is 120∘. The resultant amplitude will be
View SolutionLight waves of wavelength 5460 A, emitted by two coherent sources, meet at a point after travelling different paths. The path difference between the two wave trains at that point is 2.1μm. then phase difference will be ?
View Solution
