Two balls of mass each are placed at the two vertices of an equilateral triangle. Another ball of mass is placed at the third vertex of the triangle. Locate the centre of mass of the system.
To locate the center of mass of the system consisting of two balls of mass m at two vertices of an equilateral triangle and another ball of mass 2m at the third vertex, we can follow these steps:
Step 1: Define the Coordinates
Let's assume the vertices of the equilateral triangle are as follows:
- Vertex A (where the ball of mass 2m is located) at coordinates (0, 0)
- Vertex B (where one ball of mass m is located) at coordinates (l, 0)
- Vertex C (where the other ball of mass m is located) at coordinates (l/2, (√3/2)l)
Step 2: Identify the Masses
We have:
- Mass m1 = 2m at vertex A
- Mass m2 = m at vertex B
- Mass m3 = m at vertex C
Step 3: Calculate the Center of Mass
The formula for the center of mass (RCM) for a system of particles is given by:
\(
\text{R}_{cm} = \frac{m_1 \text{r}_1 + m_2 \text{r}_2 + m_3 \text{r}_3}{m_1 + m_2 + m_3}
\)
Where:
- \(\text{r}_1\), \(\text{r}_2\), and \(\text{r}_3\) are the position vectors of the masses.
Step 4: Substitute the Values
Substituting the coordinates and masses into the formula:
\(
\text{R}_{cm} = \frac{(2m)(0, 0) + (m)(l, 0) + (m)(\frac{l}{2}, \frac{\sqrt{3}}{2}l)}{2m + m + m}
\)
Calculating the numerator:
\(
= \frac{(0, 0) + (ml, 0) + \left(\frac{ml}{2}, \frac{m\sqrt{3}}{2}l\right)}{4m}
\)
Combining the terms in the numerator:
\(
= \frac{(ml + \frac{ml}{2}, \frac{m\sqrt{3}}{2}l)}{4m}
\)
\(
= \frac{(\frac{3ml}{2}, \frac{m\sqrt{3}}{2}l)}{4m}
\)
Step 5: Simplify the Expression
Now, simplifying the expression:
\(
= \left(\frac{3l}{8}, \frac{\sqrt{3}l}{8}\right)
\)
Step 6: Final Result
Thus, the center of mass of the system is located at:
\(
\text{R}_{cm} = \left(\frac{3l}{8}, \frac{\sqrt{3}l}{8}\right)
\)
Let's assume the vertices of the equilateral triangle are as follows:
- Vertex A (where the ball of mass 2m is located) at coordinates (0, 0)
- Vertex B (where one ball of mass m is located) at coordinates (l, 0)
- Vertex C (where the other ball of mass m is located) at coordinates (l/2, (√3/2)l)
We have:
- Mass m1 = 2m at vertex A
- Mass m2 = m at vertex B
- Mass m3 = m at vertex C
The formula for the center of mass (RCM) for a system of particles is given by:
\text{R}_{cm} = \frac{m_1 \text{r}_1 + m_2 \text{r}_2 + m_3 \text{r}_3}{m_1 + m_2 + m_3}
\)
- \(\text{r}_1\), \(\text{r}_2\), and \(\text{r}_3\) are the position vectors of the masses.
Substituting the coordinates and masses into the formula:
\text{R}_{cm} = \frac{(2m)(0, 0) + (m)(l, 0) + (m)(\frac{l}{2}, \frac{\sqrt{3}}{2}l)}{2m + m + m}
\)
= \frac{(0, 0) + (ml, 0) + \left(\frac{ml}{2}, \frac{m\sqrt{3}}{2}l\right)}{4m}
\)
= \frac{(ml + \frac{ml}{2}, \frac{m\sqrt{3}}{2}l)}{4m}
\)
= \frac{(\frac{3ml}{2}, \frac{m\sqrt{3}}{2}l)}{4m}
\)
Now, simplifying the expression:
= \left(\frac{3l}{8}, \frac{\sqrt{3}l}{8}\right)
\)
Thus, the center of mass of the system is located at:
\text{R}_{cm} = \left(\frac{3l}{8}, \frac{\sqrt{3}l}{8}\right)
\)
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SHORT ANSWER QUESTIONS II.
2 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - LONG ANSWER QUESTIONS
26 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SHORT ANSWER QUESTIONS I.
2 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
Two charge +Q each are placed at the two verticles of an equilateral triangle of side a .The intensity of electric field at the third vertex is
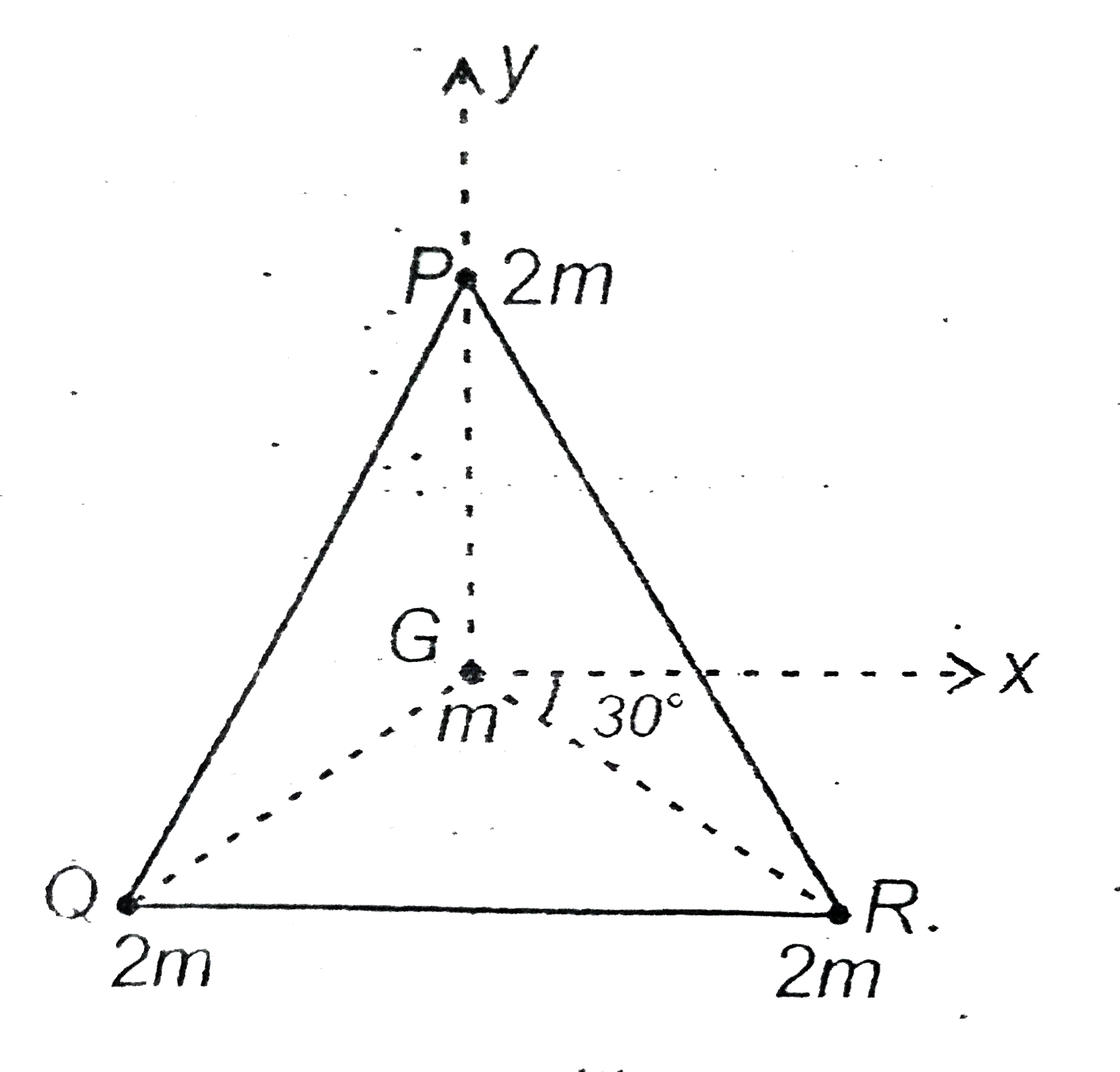
View SolutionThree equal masses 2m each are placed at the vertices an equilateral triangle PQR
(i) What is the force acting on a mass m placed at the centroid G of the triangle ?
(ii) What is the force on mass m if the mass at the vertex P is quadrupled ?
Take PG = QG = RG = 1m
View Solution
Knowledge Check
Three equal masses of 1 kg each are placed at the vertices of an equilateral triangle PQR and a mass of 2 kg is placed at the centroid O of the triangle which is at a distance of √2m from each of the vertices of the triangle. The force, in newton, acting on the mass of 2 kg is :
A2B1C3DzeroThree equal masses of 2kg each are placed at the vertices of an equilateral triangle and a mass of 4 kg is placed at the centroid of the triangle which is at a distance of √2 m from each of the vertices of the triangle. The force, (in newton) acting on the mass of 4 kg is
A2B√2C1DzeroThree identical spheres of mass m each are placed at the corners of an equilateral triangle of side 2m taking one of the corner as the origin the position vector of the centre of mass is
A√3(I∩−j∩)BI∩√3+j∩CI∩+j∩3DI∩+j∩√3
Two vertices of an equilateral triangle are (0,0)and(0,2√3). Find the third vertex
View SolutionTwo charge +Q,−Q are placed at the two verticels of an equilateral triangle of side 'a'. Then the intensity of electric field at the third vertex is
View SolutionIf two particles each of mass m are placed at the two vertices of an equilateral triangle of side a, then the resultant gravitational force on mass m placed at the third vertex is
View SolutionIf two particles each of mass m are placed at the two vertices of an equilateral triangle of side a, then the resultant gravitational force on mass m placed at the third vertex is
View SolutionThree identical spheres of mass m each are placed at the corners of an equilateral triangle of side 2m taking one of the corner as the origin the position vector of the centre of mass is
View Solution
