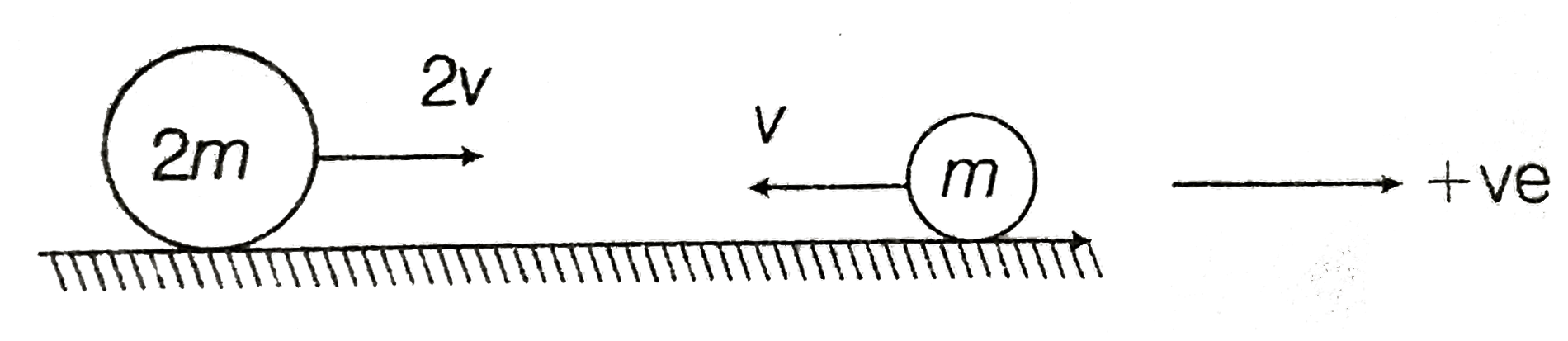
Two balls of masses m and 2m moving in opposite directions collide head on elastically with velocities v and 2v. Find their velocities after collision.
Here, v1=−v,v2=2v,m1=mandm2=2m
Substituting these values in Eqs. (iii) and (iv), we get
v′1=(m−2mm+2m)(−v)+(4mm+2m)(2v)
or v′1=(v3)+(8v3)=3v
and v′2=(2m−mm+2m)(2v)+(2mm+2m)(−v)
or v′2=(23)v−(23)v=0

i.e., the second particle (of mass 2m) comes to a rest while the first (of mass m) moves with velocity 3v in the direction. shown in figure given above.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - DC PANDEYCHAPTER - CENTRE OF MASSEXERCISE - Check points
50 Videos
- CENTRE OF MASSBOOK - DC PANDEYCHAPTER - CENTRE OF MASSEXERCISE - Taking it together
73 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEYCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
Similar Questions
Two particles of masses m and 2m moving in opposite directions collide elastically with velocity 2v and v, respectiely. Find their velocities after collision.

View SolutionTwo ball bearings of mass m each moving in opposite directions with each speeds v collide head on with each other . Predict the outcome of the collision , assuming it to the perfectly elastic.
View Solution
Knowledge Check
Two particles of mass m and 2 m moving in opposite directions collide elastically with velocities v and 2v. Find their velocities after collision.
A0, 3vB1, 3vC0, 1vD0, 4vTwo balls with masses in the ratio of 1:2 moving in opposite direction have a head-on elastic collision. If their velocities before impact were in the ration of 3:1, then velocities after impact will have the ratio :
A5:3B7:5C4:5D2:3Two balls with masses in the ratio of 1:2 moving in opposite direction have a head-on elastic collision. If their velocities before impact were in the ratio of 3:1, then velocities after impact will have the ratio :
A5:3B7:5C4:5D2:3
A ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View SolutionTwo identical bodies A and B moving with velocities 13 m/s and - 15 m/s respectively collide head-on elastically. What will be their velocities after collision?
View SolutionTwo ball bearings of mass m each moving in opposite directions with same speed v collide head on with each other. If the collision is perfectly elastic, what will be the outcome of the collision ?
View SolutionTwo masses m, and m, moving with velocities vaandvb in opposite direction collide elastically and after the collision maandmb move with velocities vbandva respectively. Then the ratio mamb is
View SolutionA ball of mass m1 is moving with velocity 3v. It collides head on elastically with a stationary ball of mass m2 . The velocity of both the balls become v after collision. Then the value of the ratio m2m1 is
View Solution
