Two particles of masses m and 2m moving in opposite directions collide elastically with velocity 2v and v, respectiely. Find their velocities after collision.

Let the final velocities of mass m and 2m be v1andv2 respectively as shown in figure.
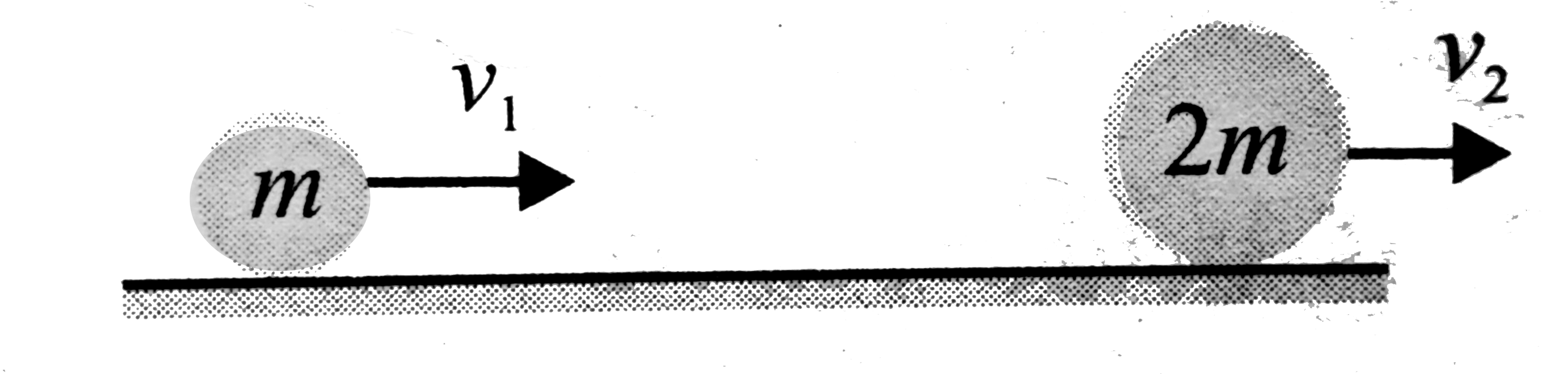
By conservation of momentum, we get
m(2v)+2m(−v)=m(v1)+2m(v2)
or 0=mv1+2mv2
or v1+2v2=0
and since the collision is elastic
v2−v1=2v−(−v)
or v2−v1=3v
Solving the above two equations we get
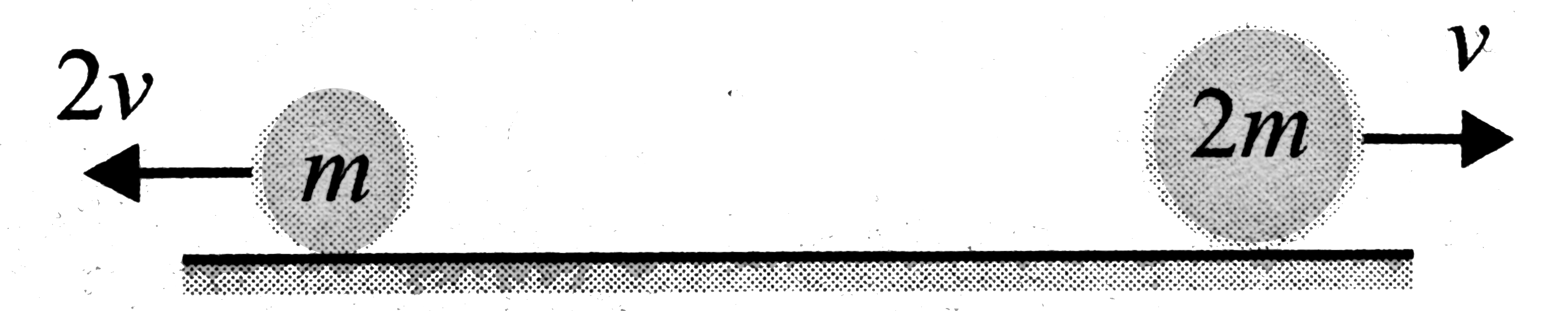
v2=v and v1=−2v
That is mass 2m returns with velocity v while mass m returns with velocity 2v in the direction shown in figure.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Solved Examples
13 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.1
20 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
Two balls of masses m and 2m moving in opposite directions collide head on elastically with velocities v and 2v. Find their velocities after collision.
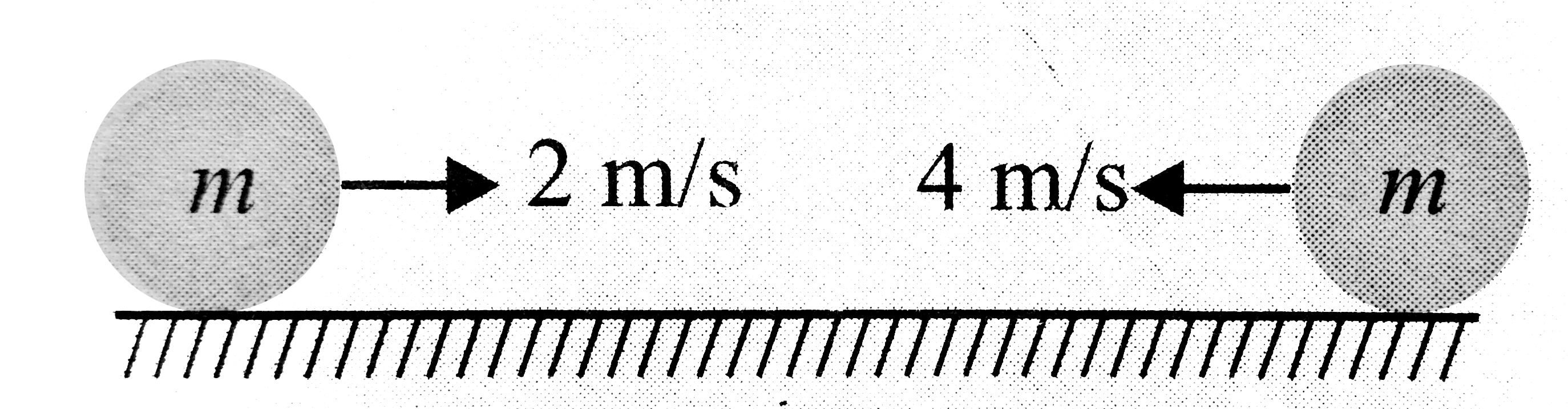
View SolutionTwo identical balls are approaching towards each other on a straight line with velocity 2m/s and 4m/s, respectiely. Find the final velocities after elastic collision between them.
View Solution
Knowledge Check
Two particles of mass m and 2 m moving in opposite directions collide elastically with velocities v and 2v. Find their velocities after collision.
A0, 3vB1, 3vC0, 1vD0, 4vA stationary partical explodes into two partical of a masses m1andm2 which move in opposite direction with velocities v1andv2. The ratio of their kinetic energies E1/E2 is
Am1/m2B1Cm1v2/m2v1Dm2/m1Two equal masses m1 and m2 moving along the same straight line with velocites +3m/s and −5m/s respectively collide elastically. Their velocities after the collision will be respectively.
A+4m/s for bothB−3m/sand+5m/sC−4m/sand+4m/sD−5m/sand+3m/s
A particle of mass m moving on a smooth surface with velocity 10m/s strikes another particle of mass 2m moving with 5m/s in the same direction . If the collision is elastic and head - on , find velocities of particles after the collision.
View SolutionA ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View SolutionTwo masses m, and m, moving with velocities vaandvb in opposite direction collide elastically and after the collision maandmb move with velocities vbandva respectively. Then the ratio mamb is
View SolutionA particle of mass m moving with velocity u1 collide elastically with another particle of same mass moving with velocity u2 in the same direction. After collision their speeds are v1 and v2 respectively then
(A) u1+v1=v2+u2, (B) u1−v1=v2+u2
View SolutionA particle of mass m1 moving with velocity v in a positive direction collides elastically with a mass m2 moving in opposite direction also at velocity v. If m2>>m1, then
View Solution
