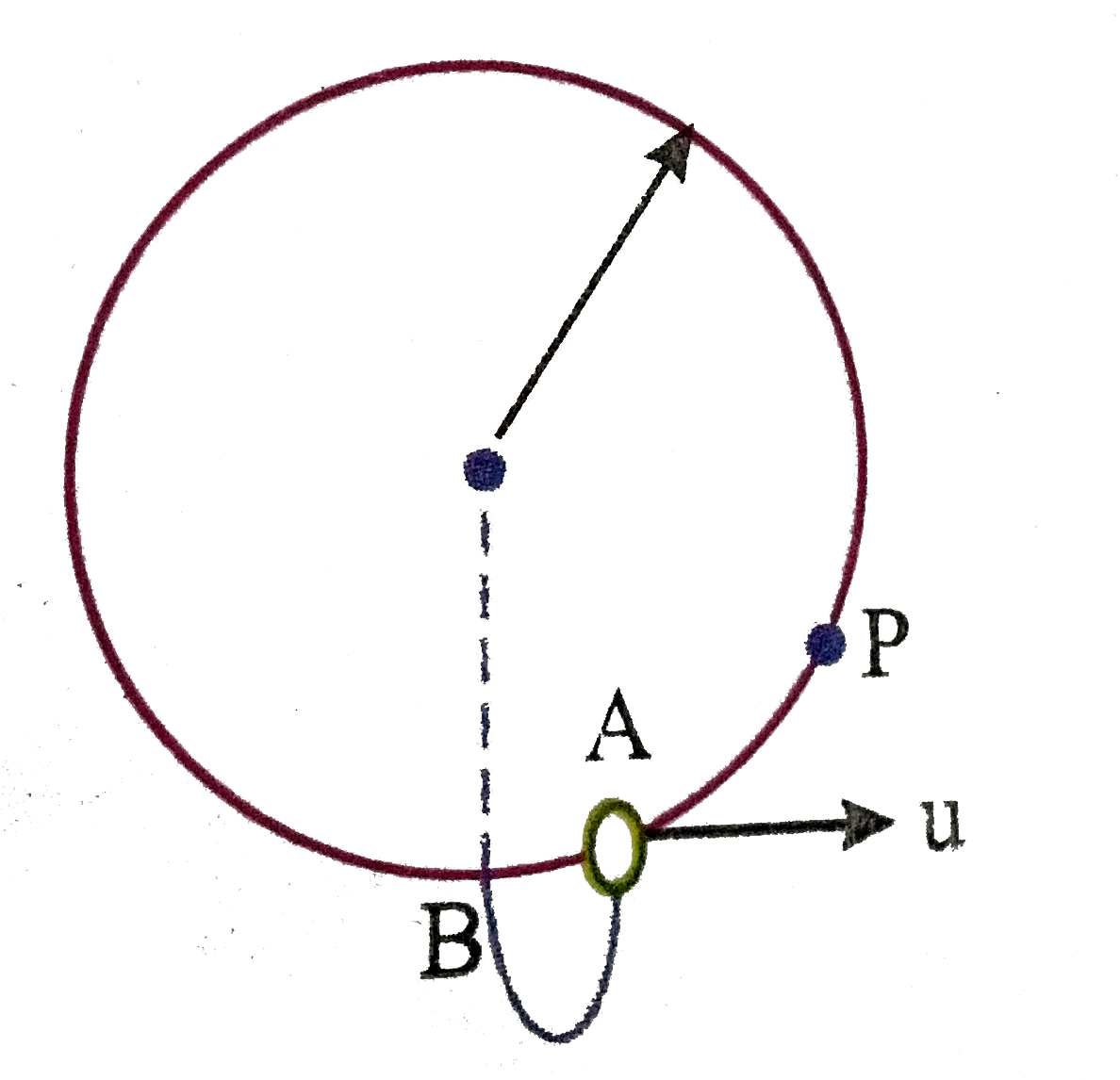
Two beads of masses $m_{1} and m_{2}$ are closed threaded on a smooth circular loop of wire of radius R. Intially both the beads are in position A and B in vertical plane .
Now , the bead A is pushed slightly so that it slide on the wire and collides with B . if B rises to the height of the centre , the centre of the loop O on the wire and becomes stationary after the collision , prove $m_{1} : m_{2} = 1 : \sqrt{2}$
Solution in Gujrati

Video Solution

Text Solution

Verified by Experts

Let $v_{1}$ be the velocity of head A of mass $m_{1}$ when it slide on the wire and collides with head B ,
$\frac{1}{2} m_{1} v_{1}^{2} = m_{1} g (2 R) (∴ h = 2 R)$
$∴ V_{1} = \sqrt{4 g} R \dots \dots . . (1)$
Collision being elastic , so the law of momentum is ,
$m_{1} v_{1} = m_{2} v_{2}$
$∴ v_{2} = \frac{m_{1}}{m_{2}} v_{1} = \frac{m_{1}}{m_{2}} (\sqrt{4 g} R) ... . . (2)$
After , collision bead of mass $m_{2}$ reaches to the height R ,
$∴ \frac{1}{2} m_{2} v_{2}^{2} = m_{2} g R (∴ h = R)$
$∴ v_{2} = \sqrt{2 g R} ... ... (3)$
Substituting the value of $v_{2}$ from equation (3) in euation (2) ,
$\sqrt{2 g R} = \frac{m_{1}}{m_{2}} \sqrt{4 g R}$
$∴ \frac{m_{1}}{m_{2}} = \frac{1}{\sqrt{2}}$
$∴ m_{1} : m_{2} = 1 : \sqrt{2}$

|

Updated on:21/07/2023

Class 11 PHYSICS WORK, ENERGY AND POWER

Topper's Solved these Questions

Similar Questions

KUMAR PRAKASHAN-WORK, ENERGY AND POWER -SECTION - B Numericals (Numerical From .DARPAN. Based On Textbook)

Home
Ask Doubt
Profile