Two blocks of mass 4kg and 6kg are connected by a string as shown in the figure. They are initially at rest on the floor. Calculate their acceleration when a force of (a) 50N (b) 100N is applied on the pulley.
To solve the problem of two blocks connected by a string and subjected to an external force, we will follow these steps:
Given:
- Mass of block 1 (m1) = 4 kg
- Mass of block 2 (m2) = 6 kg
- Force applied (F) = 50 N (in part a) and 100 N (in part b)
Step 1: Calculate the total mass of the system.
The total mass (M) of the system is the sum of the masses of the two blocks.
M=m1+m2=4kg+6kg=10kg
Step 2: Apply Newton's Second Law.
According to Newton's second law, the net force (F_net) acting on the system is equal to the mass of the system multiplied by its acceleration (a).
Fnet=M⋅a
Step 3: Determine the net force acting on the system.
The net force is the applied force minus the tension in the string (which we can assume to be negligible for this calculation since the blocks are connected and moving together). Therefore, we can consider the applied force as the net force.
For both cases:
1. When F=50N
2. When F=100N
Step 4: Calculate acceleration for each case.
Case (a): F = 50 N
Using the formula from Step 2:
Fnet=50N
50=10⋅a
a=5010=5m/s2
Case (b): F = 100 N
Using the same formula:
Fnet=100N
100=10⋅a
a=10010=10m/s2
Final Results:
- For F=50N, the acceleration a=5m/s2.
- For F=100N, the acceleration a=10m/s2.
- Mass of block 1 (m1) = 4 kg
- Mass of block 2 (m2) = 6 kg
- Force applied (F) = 50 N (in part a) and 100 N (in part b)
The total mass (M) of the system is the sum of the masses of the two blocks.
M=m1+m2=4kg+6kg=10kg
According to Newton's second law, the net force (F_net) acting on the system is equal to the mass of the system multiplied by its acceleration (a).
Fnet=M⋅a
The net force is the applied force minus the tension in the string (which we can assume to be negligible for this calculation since the blocks are connected and moving together). Therefore, we can consider the applied force as the net force.
1. When F=50N
2. When F=100N
Using the formula from Step 2:
Fnet=50N
50=10⋅a
a=5010=5m/s2
Using the same formula:
Fnet=100N
100=10⋅a
a=10010=10m/s2
- For F=50N, the acceleration a=5m/s2.
- For F=100N, the acceleration a=10m/s2.
Similar Questions
Two blocks of masses 3 kg and 6 kg are connected by a string as shown in the figure over a frictionless pulley. The acceleration of the system is

A. 4m/s2
B. 2m/s2
C. zero
D. 6m/s2
View SolutionTwo bodies of 5 kg and 4 kg are tied to a string as shown in the figure. If the surface and pulley both are smooth, acceleration of 5 kg body will be equal to :
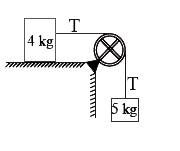
View Solution
Knowledge Check
Two blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.
 A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
Two blocks A and B of masses 2kg and 3kg are connected by a light string as shown in the figure and placed on a horizontal surface. μ between all surfaces is 0.1 and g = 10ms−1. The acceleration of the system is, when the force applied F = 45N
View SolutionTwo blocks of masses 6 kg and 4 kg are connected b a rope of mass 2 kg are resting on a frictionless floor as shown in the following figure:
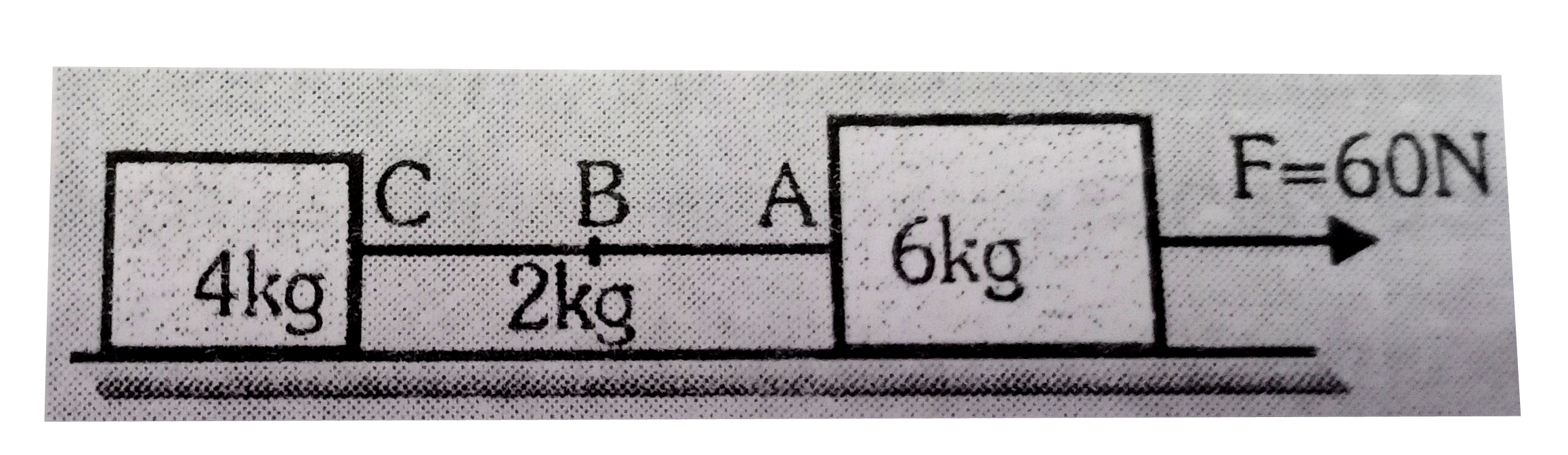
In a constant force of 60N is applied to 6 kg block then the tension in the rope at points A,B and C are respectively given by :
View SolutionTwo blocks of masses 5kg and 2 kg are initially at rest on the floor. They are connected by a light string. Passing over a light frictionless pulley. An upwards force F is applied on the pulley and maintained at a constant value. Calculate the acceleration a1 and a2 of the 5-kg and 2-kg masses, respectively, when F is (take g=10ms−2 )
(a) 30N, (b) 60N , (c) 140N
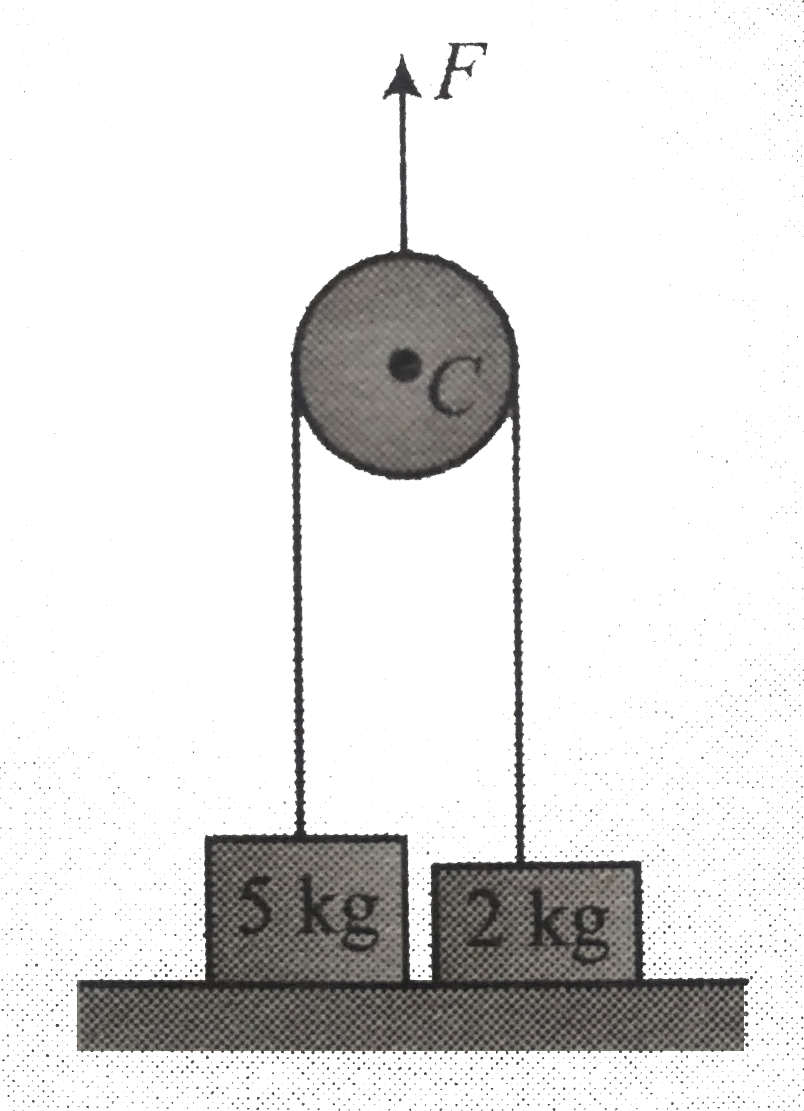 .
.
View SolutionTwo blocks of masses 4kg and 2kg are placed side on a smooth horizontal surface as shown in the figure. A horizontal force of 20N is applied on 4kgblock. Find
(a) the acceleration of each block.
(b) the normal reaction between two blocks.

View SolutionTwo blocks of masses 4kg and 2kg are placed side on a smooth horizontal surface as shown in the figure. A horizontal force of 20N is applied on 4kgblock. Find
(a) the acceleration of each block.
(b) the normal reaction between two blocks.

View SolutionTwo block of mass 4 kg and 2 kg are attached by an inextensible light string as shown in figure. Both the blocks are pulled vertically upwards by a force F=120N
Find
(a) the acceleration of the blocks
(b) tension in the string. (Take g=10ms−2)
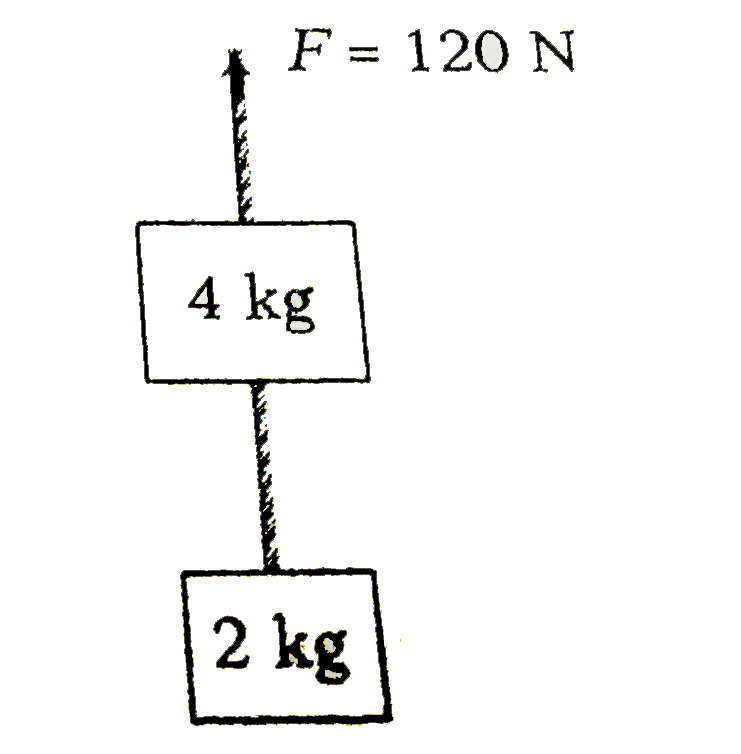
View SolutionTwo block of mass 4 kg and 2 kg are attached by an inextensible light string as shown in figure. Both the blocks are pulled vertically upwards by a force F=120N

Find
(a) the acceleration of the blocks,
(b) tension in the string. (Take g=10ms−2)
View Solution
