Two blocks of masses m and 3m, intially at rest, are pushed from line 1 to line 2 by applying an equal force F as shows in the following figure: Which of the following statements is correct regarding the kinetic energes of the two blocks when they reach the line 2? (a) Both blocks have equal kinetic energy , (b)block of mass 3m has greater kintic energy, (c) block of mass m has greater kinetic energy.

Equal force F is acting on both the blocks and displacement of both the blocks is also same. Hence, work done on each block is equal. As the worl done is equal to change in kinetic energy, the change in kinetic energy of both the blocks is also same. Since both the blocks started initially from rest, their initial kinetic energy is zero.so, W=Kf−Ki,Ki=0,W=Kf. W is same for both the blocks and hence, Kf , is also same . the carrect option is (a).
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Tough & Tricky (PROBLEMS)15videos
WORK, ENERGY AND POWER
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - NCERT FILE (NCERT Textbook Exercise)23videos
WORK, ENERGY AND POWER
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - PRACTICE PROBLEMS27videos
WORK, ENERGY AND POWER
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST14videos
WAVES
Similar Questions
Knowledge Check
- Question 1 - Select One
A block of mass m at rest is acted upon by a force F for a time t. The kinetic energy of block after time t is
AFt2mBF2t22mCFt22mDF2t23m - Question 2 - Select One

Two blocks of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is :Am2gm1+m2Bm1gm1+m2C4m2g−m1gm1+m2Dm2gm1+4m2 - Question 3 - Select One
A bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
A12mv2×m(m+M)B12mv2×M(m+M)C12mv2×(M+m)mD12Mv2×m(m+M)
A bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
View SolutionTwo block of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is:
 View Solution
View SolutionTwo blocks of mass m and M are lying on a smooth table. A spring is attached with the block of mass M (see fig). Block of mass m is given a velocity towards the other block. Find the value of Mm for which the kinetic energy of the system will never fall below one third of the initial kinetic energy imparted to the block of mass m.
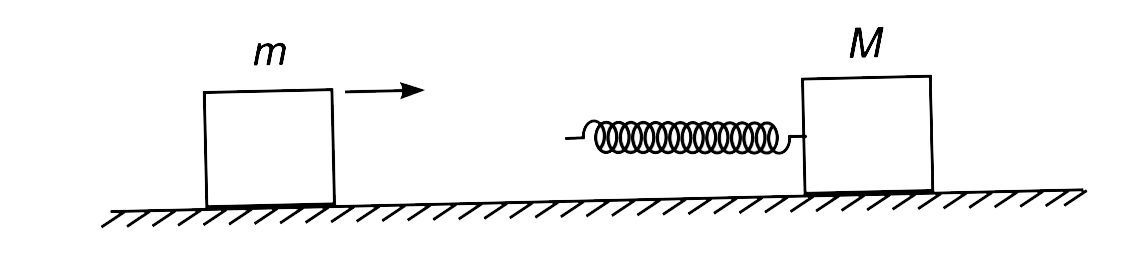 View Solution
View SolutionA bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
View SolutionTwo block of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is:
 View Solution
View Solution

