Two cells E1 (with emf 4V and internal resistance 2Ω) and E2 (with emf 2V and intenral resistnace 2Ω) are connected in parallel. The combination is connected in parallel with a 8Ω resistance R as shown in figure. Calcualte the currents passing through 2V cell and through the resistance R.
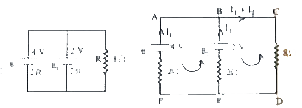
Applying Kirchhoff.s second law to the loop ABEFA.
then −2+2I2−2I1+4=0
2I1−2I2=2 ….(1)
Applying Kirchhoff.s seocnd law to the loop BCDEB, then −8(I1+I2)−2I2+2=0
8I1+10I2=2 ..........(2)
Solving equation (1) and (2), we get
I1=23A,I2=−13A,I1+I2=13A
Thus, the current passing through 2V cells is I2=−13A. That is 13A current flows though 2V cell from B to E direction. And 13A current flows through 8Ω resistance from C to D.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE - IA
2 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL -II PRACTICE SHEET (ADVANCED) (Linked Comprehension Type Questions))
9 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PROBLEMS (LEVEL-II)
27 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
42 Videos
Similar Questions
Two resistors of resistances 2Ω and 6Ω are connected in parallel. This combination is then connected to a battery of emf 2 V and internal resistance 0.5Ω. What is the current flowing through the battery ?
View SolutionA cell of emf 2V and internal resistance 4Ω is connected across a parallel combination of two resistors of resistance 10Ω and 20Ω. Find the current through each resistor using Kirchhoff's laws.
View Solution
Knowledge Check
Two batteries, one of emf 18 V and internal resistance 2 Ω and the other of emf 12 V and internal resistance 1Ω , are connected in parallel as shown in Fig. The voltmeter V will record a reading of
 A15VB14VC30VD18V
A15VB14VC30VD18VTwo batteries of emfs 2 V and 1 V of internal resistances 1Ωand2Ω respectively are connected in parallel. The effective emf of the combination is
A32VB53VC35VD2VTwo resistors of resistances 2Ω and 6Ω are connected in parallel. This combination is then connected to a battery of emf 2 V and internal resistance 0.5Ω. What is the current flowing thrpough the battery ?
A4AB43AC417AD1A
A 6 V cell with 0.5Ω internal resistance , a 10 v cell with 1Ω internal resistance and a 12Ω external resistance are connected in parallel . The current (in amper) through the 10 V cell is
View SolutionA cell of e.m.f. 1.8 V and internal resistance 2 Ω is connected in series with an ammeter of resistance 0.7 Ω and a resistor of 4.5 Ω as shown in Fig.
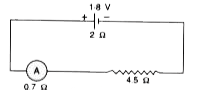
What would be the reading of the ammeter ?
View SolutionA cell E1 of emf 6V and internal resistance 2Ω is connected with another cell E2 of emf 4V and internal resistance 8Ω (as shown in the figure). The potential difference across points X and Y is :

View Solution4 cells each of emf 2 V and internal resistance of 1Ω are connected in parallel to a load resistor of 2Ω. Then the current through the load resistor is
View SolutionA cell of e.m.f. 2 V and internal resistance 2Ω and an external resistance 18Ω forms closed circuit. The current through the circuit is
View Solution
