Two containers of equal volume contain the same gas at pressure and and absolute temperature and , respectively. On joining the vessels, the gas reaches a common pressure and common temperature . The ratio is equal to
The correct Answer is:D
To solve the problem, we will use the ideal gas law and the principles of thermodynamics. Let's break down the steps:
1. Understand the Ideal Gas Law: The ideal gas law states that for a given amount of gas, the product of pressure (P), volume (V), and temperature (T) is a constant. Mathematically, it is expressed as:
PV=nRT
where n is the number of moles of gas and R is the universal gas constant.
2. Calculate Moles in Each Container:
- For the first container:
P1V=n1RT1⟹n1=P1VRT1
- For the second container:
P2V=n2RT2⟹n2=P2VRT2
3. Combine the Containers: When the two containers are joined, the total volume becomes 2V and the total number of moles of gas becomes n1+n2.
4. Total Moles Calculation:
n1+n2=P1VRT1+P2VRT2
5. Expressing Total Moles:
n1+n2=V(P1RT1+P2RT2)
6. Using Ideal Gas Law for Combined System: The pressure P and temperature T of the combined gas can be expressed as:
P(2V)=(n1+n2)RT
7. Substituting for n1+n2:
P(2V)=(V(P1RT1+P2RT2))RT
8. Simplifying the Equation:
2P=(P1T1+P2T2)T
9. Finding the Ratio PT:
PT=12(P1T1+P2T2)
10. Final Result: Therefore, the ratio PT is given by:
PT=P12T1+P22T2
PV=nRT
where n is the number of moles of gas and R is the universal gas constant.
- For the first container:
P1V=n1RT1⟹n1=P1VRT1
- For the second container:
P2V=n2RT2⟹n2=P2VRT2
n1+n2=P1VRT1+P2VRT2
n1+n2=V(P1RT1+P2RT2)
P(2V)=(n1+n2)RT
P(2V)=(V(P1RT1+P2RT2))RT
2P=(P1T1+P2T2)T
PT=12(P1T1+P2T2)
PT=P12T1+P22T2
Topper's Solved these Questions
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Multiple Corrects
29 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Assertion-Reasoning
6 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Subjective
22 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Multiple Correct Answer Type
9 Videos
Similar Questions
Two vessels of volume (V1)and(V2) contain the same ideal gas. The pressures in the vessels are (P1)and(P2) and the temperatures are (T1)and(T2) respectively . The two vessels are now connected to each other through a narrow tube. Assuming thar no heat is exchanged between the surrounding and the vessels, find the common pressure and temperature attained after the connection.
View SolutionTwo gases which are at pressure p1 volume V1 and temperature T1 and pressure p2 volume V2 and temperature T2, respectively are forced into a vessel of volume V at temperature T. Calculate pressure of the mixture.
View Solution
Knowledge Check
Two containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
Ap1T2+p2T1T1×T2Bp1T2+p2T1T1+T2C12[p1T2+p2T1T1T2]Dp1T2−p2T1T1×T2]Two identical vessels contain the same gas at pressure P1 and P2 at absolute temperature T1andT2, respectively. On joining the vessels with a small tube as shown in the figure. The gas reaches a common temperature T and a common pressure P. Determine the ratio P/T
 A[P1T1+P2T2T1T2]B12[P1T1+P2T2T1T2]C12[P1T2+P2T1T1T2]D[P1T2+P2T1T1T2]
A[P1T1+P2T2T1T2]B12[P1T1+P2T2T1T2]C12[P1T2+P2T1T1T2]D[P1T2+P2T1T1T2]Two idential container joined by a small pipe initially contain the same gas at pressure p0 and absolute temperature T0. One container is now maintained at the same temperature while the other is heated to 2T0. The common pressure of the gas
A32p0B43p0C5p0D2p0
An ideal gas in a thermally insulated vessel at internal pressure =P1, volume =V1 and absolute temperature =T1 expands irreversibly against zero external pressure, as shown in the diagram. The final internal pressure, volume and absolute temperature of the gas are P2,V2 and T2 respectively. For this expansion.
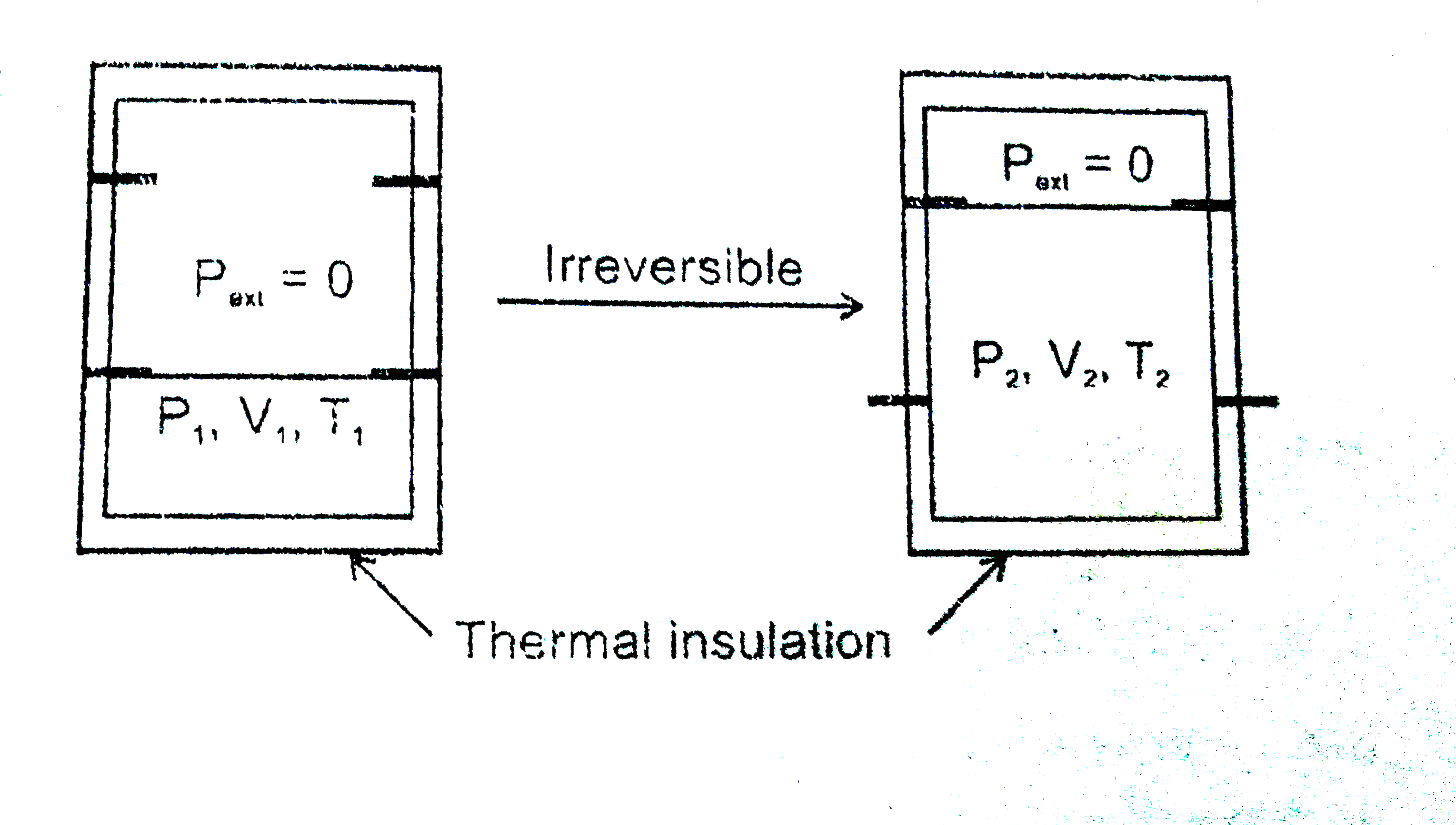
View SolutionAn insulated container of gas has two chambers separated by an insulating partition. One of the chambers has volume V1 and contains ideal gas at pressure P1 and temperature T1. The other chambers has volume V2 and contains ideal gas at pressure P2 and temperature T2. If the partion is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
View SolutionTwo identical containers joned by a small pipe initially contain the same gas at pressue po and abosolute temperature To. One container is now mantained at the same temperature while the other is heated to 2T0. The commmon pressure of the gases will be
View SolutionAn ideal gas in thermally insulated vessel at internal (pressure)=P1,(volume)=V1 and absolute temperature=T1 expands irreversiby against zero external, pressure , as shown in the diagram,
The final internal pressure, volume and absolute temperature of the gas are p2,V2andT2, respectively . For this expansion

View SolutionTwo adiabatic containers have volumes V1 and V2 respectively. The first container has monoatomic gas at pressure p1 and temperature T1. The second container has another monoatomic gas at pressure p2 and temperature T2. When the two containers are connected by a narrow tube, the final temperature and pressure of the gases in the containers are P and T respectively. Then
View Solution
