Two cylindrical vessels of the same type contain a liquid of density ρ. The bases of both the vessels lie on the same horizontal plane. The depth of the liquid in the left vessel is h1 and that in the right vessel is h2. The area of cross-section of the base of each vessel is A. If the vessels are connected by a tube, then how much is the work done by gravity to equalize the levels of the liquid in the vessels (suppose h1>h2)?
When the vessels are connected, the level of the liquid in the left-hand vessel will fall and that in the right-hand one will go up.

The initial difference in the liquid levels in the vessles = h1−h2. When the levels in both the vessels become the same, the level of liquid in the left-hand vessel will fall by 12(h1−h2) and that in the right-hand vessel will rise by 12(h1−h2).
Mass of this liquid = 12(h1−h2)Aρ.
The centre of gravity of this liquid moves up against gravity by 12(h1−h2).
∴ Increase in potential energy of this liquid
= work done against gravity
12(h1−h2)Aρ×g×12(h1−h2)=14Aρg(h1−h2)2.
Topper's Solved these Questions
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - SECTION RELATED QUESTIONS
12 Videos
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
37 Videos
- FRICTION BOOK - CHHAYA PUBLICATIONCHAPTER - FRICTION EXERCISE - CBSE SCANNER
7 Videos
- KINETIC THEORY OF GASESBOOK - CHHAYA PUBLICATIONCHAPTER - KINETIC THEORY OF GASESEXERCISE - CBSE Scanner
9 Videos
Similar Questions
Two identical cylindrical vessel with their bases at the same level each contain a liquid of density ρ. The height of the liquid in one vessel is h1 and in the other is h2 the area of either base is A. What is the work done by gravity is equalising the levels when the two vessels are connected?
View SolutionTwo identical cylindrical vessels with their bases at the same level each, contain a liquid of density ρ. The area of either base is A but in one vessel the liquid heigth is h1 and in the other liquid height is h2(h2<h1). If the two vessel are connected, the work done by gravity in equalizing the level is
View Solution
Knowledge Check
A liquid of density ρ is filled in two indentical cylindrical vessels with base area A and height of the liquid in one vessel is h1 and that in the other vessel is h2. If both the vesels are connected with their base at the same level then calculate the work done by gravity in equalizing the levels.
A(h1−h2)gρB(h1−h2)gAρC12(h1−h2)2gAρD14(h1−h2)2gAρTwo identical cylindrical vessels with their bases at the same level each, contain a liquid of density ρ. The area of either base is A but in one vessel the liquid height is h1 and in the other liquid height is h2(h2<h1). If the two vessel are connected, the work done by gravity in equalizing the level is
A12(h1−h2)2AρgB12(h1+h2)2AρgC12(h21−h22)2AρgD14(h1−h2)2Aρg
Two identical cylindrical vessels with their bases at the same level each, contain a liquid of density ρ. The area of either base is A but in one vessel the liquid heigth is h1 and in the other liquid height is h2(h2<h1). If the two vessel are connected, the work done by gravity in equalizing the level is
View SolutionTwo vessels A and B have same base area. Equal volumes of a liquid are poured in the two vessel to difference heights hA and hB(>hA. In which vessel, the force on the base of vessel will be more?
View SolutionCoefficient of volume expansion of a vessel is greater than the liquid it contains. If the vessel is heated for long time, the level of liquid in the vessel
View SolutionTwo vessels A and B have same base area. Equal volumes of a liquid are poured in the two vessel to different heights hA and hB(hB>hA). In which vessel, the force on the base of vessel will be more?
View SolutionA conical vessel wihout a bottom stands on a table. A liquid is poured with the vessel & as soon as the level reaches h, the pressure of the liquid raises the vessel. The radius of the base of the vessel is R and half angle of the cone α and the weight of the vessel is W. What is the density of the liquid ?
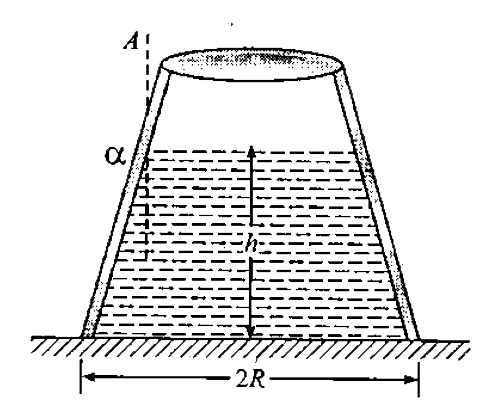
View SolutionA conical vessel wihout a bottom stands on a table. A liquid is poured with the vessel & as soon as the level reaches h, the pressure of the liquid raises the vessel. The radius of the base of the vessel is R and half angle of the cone α and the weight of the vessel is W. What is the density of the liquid ?

View Solution
