Two friends A and b playing a game of collision of balls and throwing balls from the top of two towers simultaneously as shown in the figure. If the balls collide in air at point P and point O is treated as origin then answer the following questions (g=10m/s2)

Distance D betwen the towers is:
(100,75)m
(100,125)m
(75,100)m
(175,100)m
The correct Answer is:A
Step by step video & image solution for Two friends A and b playing a game of collision of balls and throwing balls from the top of two towers simultaneously as shown in the figure. If the balls collide in air at point P and point O is treated as origin then answer the following questions (g=10m//s^(2)) Distance D betwen the towers is: by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - MATCH THE COLUMN
3 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - MCQ_TYPE
23 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
Similar Questions
A ball is dropped from the roof of a tower height h. The total distance covered by it in the last second of its motion is equal to the distance covered by it in first three seconds. The value of h in metre is (g=10m/s2)
View SolutionA ball is dropped from the roof of a tower height h. The total distance covered by it in the last second of its motion is equal to the distance covered by it in first three seconds. The value of h in metre is (g=10m/s2)
View Solution
Knowledge Check
A ball is thrown from the top of a tower with an initial velocity of 10m/s at an angle of 30∘above the horizontal. It hits the ground at a distance of 17.3 m from the base of the tower. The height of the tower (g=10m/s2) will be
A5 mB20 mC15 mD10 m
A ball is thrown from the top of a tower with an intial velocity of 10 m//s at an angle 37∘ above the horizontal, hits the ground at a distance 16 m from the base of tower. Calculate height of tower. [g=10m/s2]
View SolutionA ball projected upwards from the foot of a tower. The ball crosses the top of the tower twice after an interval of 6s and the ball reaches the ground after 12s. The height of the tower is (g=10m/s2) :
View SolutionA ball projected upwards from the foot of a tower. The ball crosses the top of the tower twice after an interval of 6s and the ball reaches the ground after 12s. The height of the tower is (g=10m/s2) :
View SolutionTwo balls are projected from points A and B in vertical plane as shown in figure. AB is a straight vertical line. The balls can collide in mid air if v1/v2 is equal to
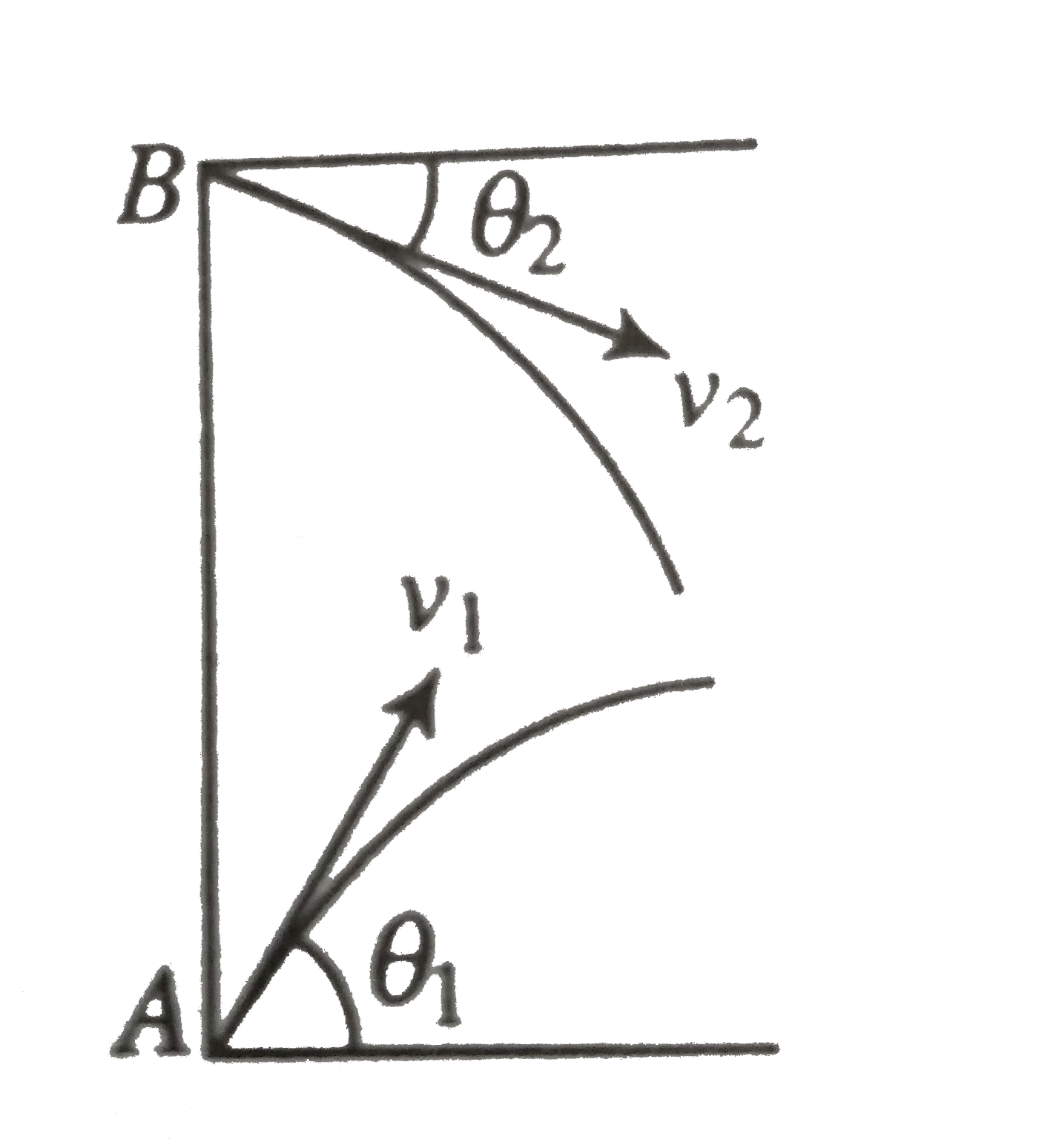
View SolutionTwo balls are projected from points A and B in vertical plane as shown in figure. AB is a straight vertical line. The balls can collide in mid air if v1/v2 is equal to

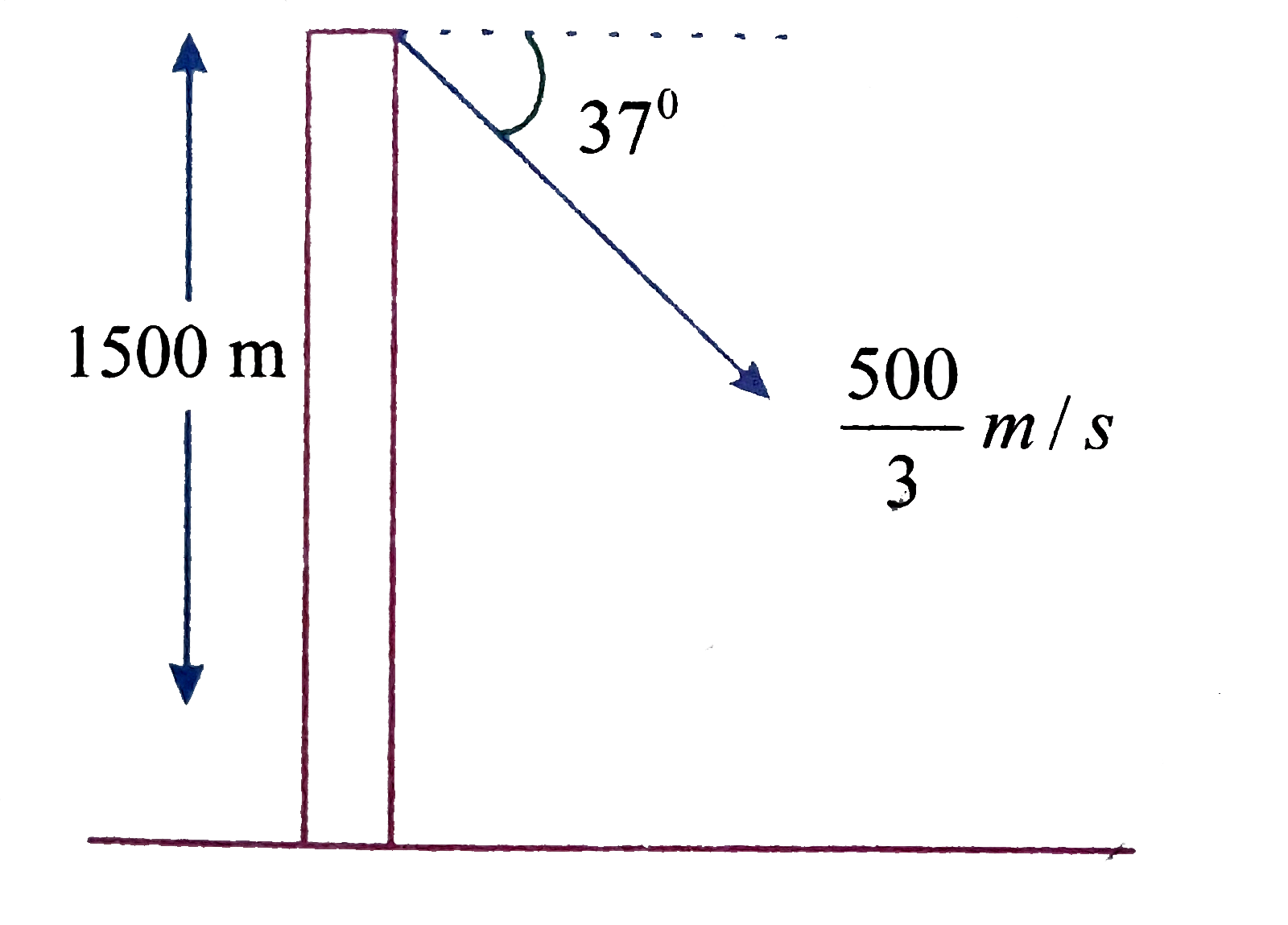
View SolutionA particle is projected from a tower as shown in figure, then find the distance from the foot of the tower where it will strike the ground. (g=10m/s2)
View SolutionA ball is thrown upwards from the top of a tower 40m high with a velocity of 10m/s. Find the time when it strikes the ground. Take g=10m/s2.
View Solution
