Two identical buggies move one after the other due to inertia (without friction) with the same velocity v0. A man of mass m rides the rear buggy. At a certain moment the man jumps into the front buggy with a velocity u relative to his buggy. Knowing that the mass of each buggy is equal to M, find the velocities with which the buggies will move after that.
If we take rear buggy + man as system. There is no external force acting on system is horizontal direction. Hence linear momentum of the system should be conserved. Let velocity of rear buggy just after jumping be →Vr, the velocity of man just after jumping will be (→Vr+→u)
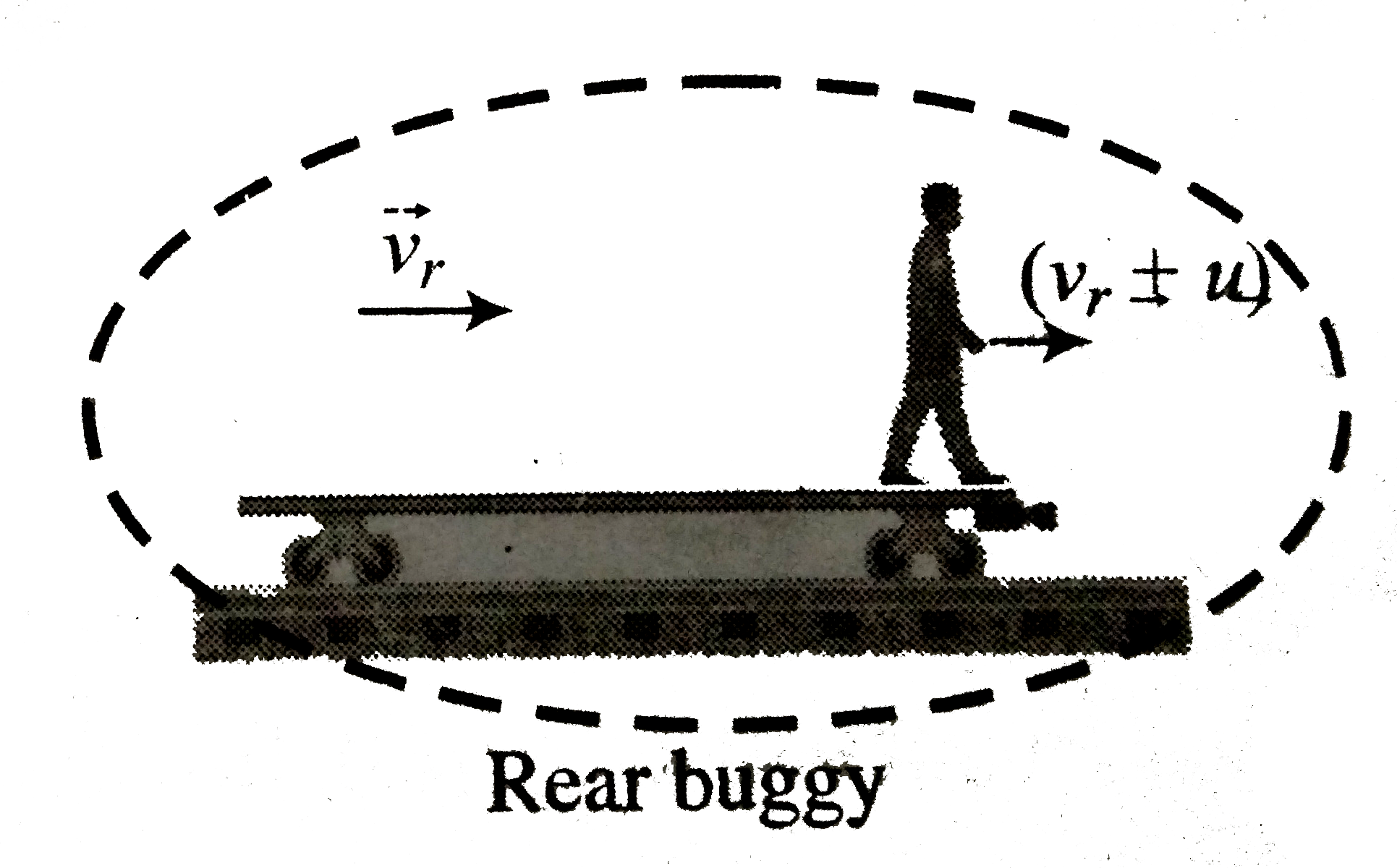
→pi=(M+m)→V0
→pf=M→Vr+m(→Vr+→u) and →pi=→pf
⇒(M+m)→V0=M→Vr+m(→Vr+→u)
→Vr=→V0−m→u(M+m)..............i

Now considering front buggy andn man as system, and again conserving linear momentum in horizontal direction.
M0→V0+m(→u+→Vr)=(m+M)→Vr
M→V0=m(→u+→V0−μ→u(M+m))=(m+M)→Vf
⇒→Vf=→V0=Mm→u(M+m)2
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Solved Examples
13 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.1
20 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
Two particles initially at rest move towards each other due to internal forces. Find the velocity of centre of mass when velocity of one particle is v and that of the other is 2v
View SolutionA man of mass m is standing on the floor of a lift. Find his apparent weight when the lift at rest or moving with uniform velocity v.
View Solution
Knowledge Check
Two identical buggies of each of mass 150kg move one after the other friction with same velocity 4m/s. A man of mass m rides the rear buggy. At a certain moment, the man jumps into the front buggy with a velocity v relative to his buggy. As a result of this process, real boggy stops. If the sum of kinetic energies of the man and the front buggy just after collision with the from buggy differs from that just before collision by 2700J then
The velocity v of the man relartive to the buggy isA16m/sB8m/sC10m/sD15m/sTwo identical buggies of each of mass 150kg move one after the other friction with same velocity 4ms. A man of mass m rides the rear buggy. At a certain moment, the man jumps into the front buggy with a velocity v relative to his buggy. As a result of this process, real boggy stops. If the sum of kinetic energies of the man and the front buggy just after collision with the from buggy differs from that just before collision by 2700J then
the mass m of the man isA60kgB75kgC50kgD90kgA man of mass m stands on a long flat car of mass M , moving with velocity V.If he now begins to run with velocity u, with respect to the car , in the same direction as V, the the velocity of the car will be
AV−mu/MBV−mu/(m+M)CV+mu/(m+m)DV−u(M−m)/(M+m)
A buggy of mass 100 kg is free to move on a frictionless horizontal track. Two men, each of mass 50 kg, are standing on the buggy, which is initially stationary. The men jump off the buggy with velocity =10m/s relative to the buggy. In one situation, the men jump one after the other. in another situation, the men jump simultaneously. what is the ratio of the velocities of the buggy in two cases?
View SolutionTwo men, each of mass m, stand on the edge of a stationary buggy of mass M. Assuming the friction to be negligible, find the velocity of the buggy after both men jump off with the same horizontal velocity u relative to the buggy: (1) simultaneously, (2) one after the other. In what case will the velocity of the buggy be greater and how many times?
View SolutionA flat car of mass M with a child of mass m is moving with a velocity v1. The child jumps in the direction of motion of car with a velocity u with respect to the car. Find the final velocities of the child and that of the car after jump.
View SolutionA man of mass m1 is standing on a platform of mass m2 kept on a smooth horizontal surface. The man starts moving on the platform with a velocity vr relative to the platform. Find the recoil velocity of platform.
View SolutionA rope thrown over a pulley has a ladder with a man of mass m on one of its ends and a counter balancing mass M on its other end. The man climbs with a velocity vr relative to ladder. Ignoring the masses of the pulley and the rope as well as the friction on the pulley axis, the velocity of the centre of mass of this system is:
View Solution
