Two identical springs have the same force constant of 147Nm−1. What elongation will be produced in each spring in each case shown in figure. Take g=9.8ms−2
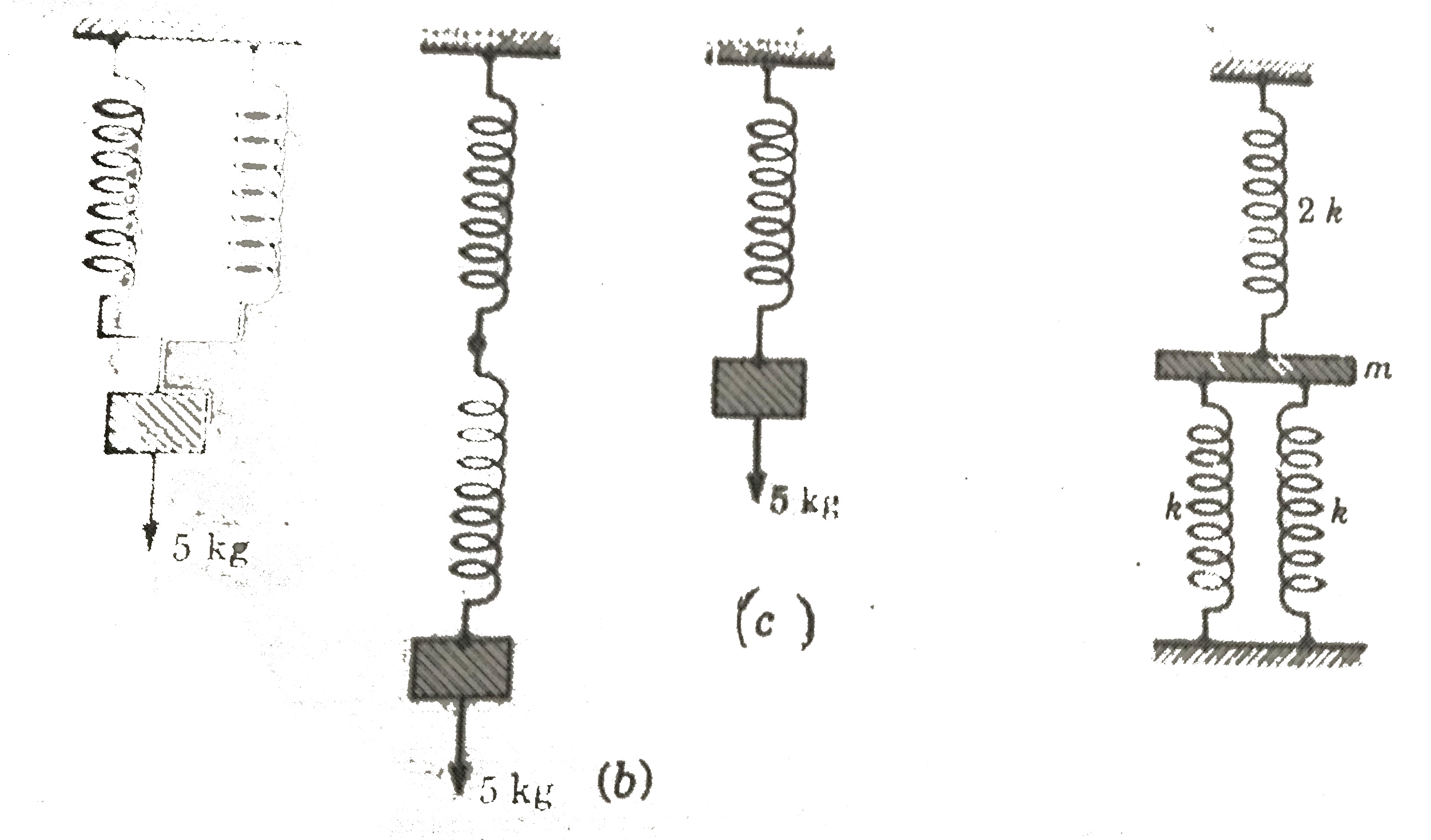
The correct Answer is:(a). 1/6,
(b). 1/3,
(c). 1/3 m
Step by step video, text & image solution for Two identical springs have the same force constant of 147Nm^(-1). What elongation will be produced in each spring in each case shown in figure. Take g=9.8ms^(-2) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - SL ARORACHAPTER - OSCILLATIONSEXERCISE - Exercise233videos
OSCILLATIONS
- BOOK - SL ARORACHAPTER - OSCILLATIONSEXERCISE - Problem23videos
OSCILLATIONS
- BOOK - SL ARORACHAPTER - MOTION IN ONE DIMENSIONEXERCISE - problems for self practice66videos
MOTION IN ONE DIMENSION
- BOOK - SL ARORACHAPTER - Physical worldEXERCISE - Exercise49videos
Physical world
Similar Questions
Two identical springs have the same force constant of 147Nm−1. What elongation will be produced in each spring in each case shown in figure? g=9.8ms−2.
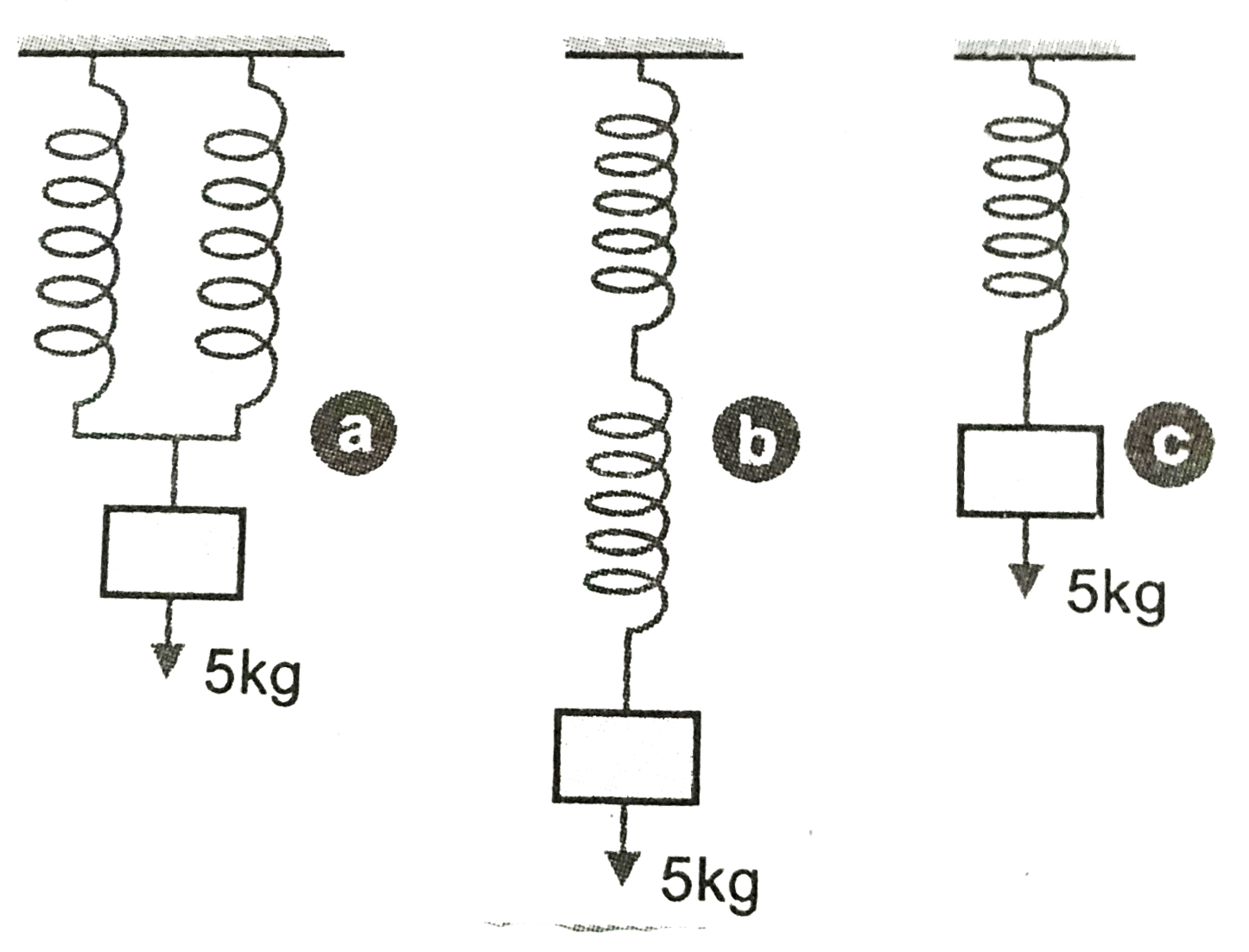 View Solution
View SolutionTwo identical springs, each of spring factor k, may be connected in the following ways. Deduce the spring factor of the oscillation of the body in each case.
View Solution
Knowledge Check
- Question 1 - Select One
What will be the force constant of the spring system shown in figure?
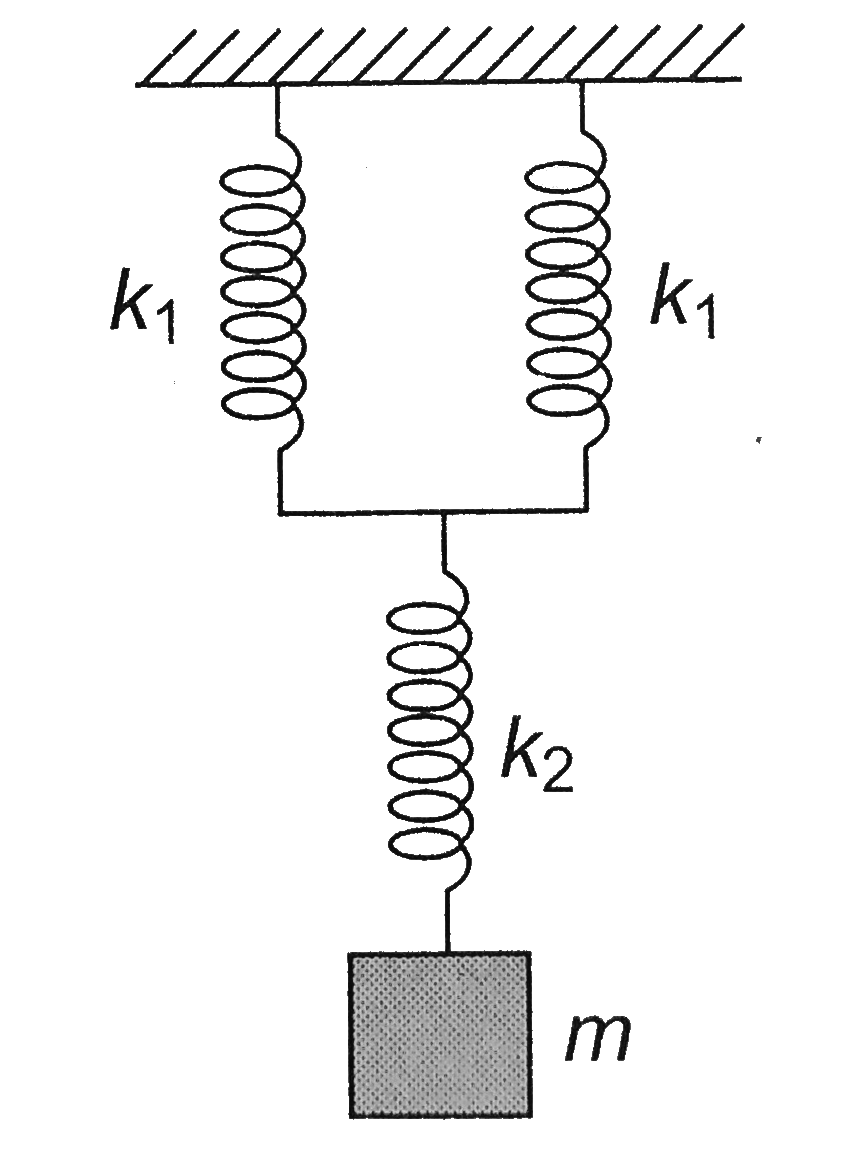 A[1k1+1k2]B[12k1+1k2]−1C[1k1+1k2]−1D[12k1+1k2]
A[1k1+1k2]B[12k1+1k2]−1C[1k1+1k2]−1D[12k1+1k2] - Question 2 - Select One
Natural length of a spring is 60 cm, and its spring constant is 4000 N/m, A mass of 20 kg is hung from it. The extension produced in the spring is (take g=9.8m/s2)
A4.9 cmB0.49 cmC9.4 cmD0.94 cm - Question 3 - Select One
Natural length of a spring is 60 cm and its spring constant is 4000 N/m. A mass of 20 kg is hung from it. The extension produced in the spring is (Take, g=9.8m/s2)
A4.9 cmB0.49 cmC9.4 cmD0.94 cm
In the situation shown in figure all contact surfaces are smooth. The force constant of the spring is K. Two forces F are applied as shown. The maximum elongation produced in the spring is how many times of F/K (initially the spring is relaxed)?
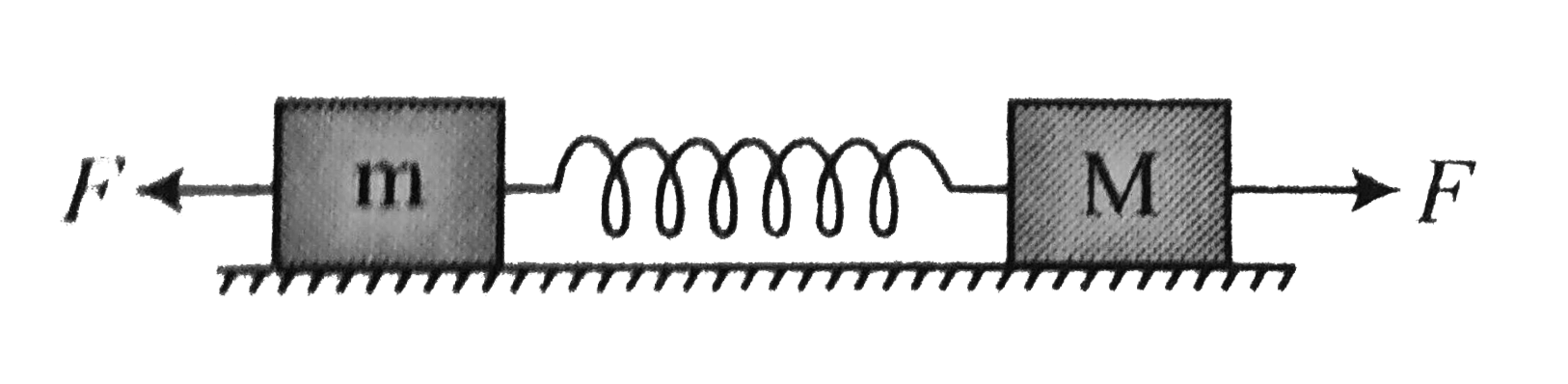 View Solution
View SolutionTwo springs have their force constant as K1 and K2(K1<K2). When they are stretched by the same force:
View SolutionTwo springs have their force constant as k1 and k2(k1>k2). When they are streched by the same force.
View SolutionNatural length of a spring is 60 cm, and its spring constant is 4000 N/m, A mass of 20 kg is hung from it. The extension produced in the spring is (take g=9.8m/s2)
View SolutionTwo springs have their force constant as k1 and k2(k1>k2). When they are stretched by the same force.
View Solution

