Two masses m1 and m2 at an infinite distance from each other are initially at rest, start interacting gravitationally. Find their velocity of approach when they are at a distance r apart.
The correct Answer is:A, B
To find the velocity of approach of two masses m1 and m2 when they are at a distance r apart, we can follow these steps:
Step 1: Understand the gravitational force
When two masses m1 and m2 are at a distance r apart, the gravitational force F acting between them can be expressed using Newton's law of gravitation:
F=Gm1m2r2
where G is the gravitational constant.
Step 2: Determine the acceleration of each mass
The acceleration a1 of mass m1 due to the gravitational force exerted by mass m2 is given by:
a1=Fm1=Gm2r2
Similarly, the acceleration a2 of mass m2 due to the gravitational force exerted by mass m1 is:
a2=Fm2=Gm1r2
Step 3: Find the relative acceleration
The relative acceleration a of the two masses towards each other is the sum of their accelerations:
a=a1+a2=Gm2r2+Gm1r2=G(m1+m2)r2
Step 4: Relate acceleration to velocity
The relative acceleration can also be expressed in terms of the relative velocity v and the distance r:
a=dvdt=dvdr⋅drdt
Since drdt is negative (as r decreases), we can write:
a=−vdvdr
Step 5: Set up the equation
Substituting the expression for acceleration:
−vdvdr=G(m1+m2)r2
Step 6: Rearrange and integrate
Rearranging gives:
vdv=−G(m1+m2)r2dr
Now, we can integrate both sides. The left side integrates from 0 to v and the right side from ∞ to r:
∫v0vdv=−G(m1+m2)∫r∞1r2dr
Step 7: Perform the integration
The left side becomes:
v22
The right side evaluates to:
−G(m1+m2)(−1r)r∞=G(m1+m2)(0−(−1r))=G(m1+m2)r
Step 8: Combine results
Thus, we have:
v22=G(m1+m2)r
Multiplying both sides by 2 gives:
v2=2G(m1+m2)r
Step 9: Solve for v
Taking the square root of both sides, we find the velocity of approach:
v=√2G(m1+m2)r
Final Answer
The velocity of approach of the two masses when they are at a distance r apart is:
v=√2G(m1+m2)r
When two masses m1 and m2 are at a distance r apart, the gravitational force F acting between them can be expressed using Newton's law of gravitation:
F=Gm1m2r2
where G is the gravitational constant.
The acceleration a1 of mass m1 due to the gravitational force exerted by mass m2 is given by:
a1=Fm1=Gm2r2
Similarly, the acceleration a2 of mass m2 due to the gravitational force exerted by mass m1 is:
a2=Fm2=Gm1r2
The relative acceleration a of the two masses towards each other is the sum of their accelerations:
a=a1+a2=Gm2r2+Gm1r2=G(m1+m2)r2
The relative acceleration can also be expressed in terms of the relative velocity v and the distance r:
a=dvdt=dvdr⋅drdt
Since drdt is negative (as r decreases), we can write:
a=−vdvdr
Substituting the expression for acceleration:
−vdvdr=G(m1+m2)r2
Rearranging gives:
vdv=−G(m1+m2)r2dr
Now, we can integrate both sides. The left side integrates from 0 to v and the right side from ∞ to r:
∫v0vdv=−G(m1+m2)∫r∞1r2dr
The left side becomes:
v22
The right side evaluates to:
−G(m1+m2)(−1r)r∞=G(m1+m2)(0−(−1r))=G(m1+m2)r
Thus, we have:
v22=G(m1+m2)r
Multiplying both sides by 2 gives:
v2=2G(m1+m2)r
Taking the square root of both sides, we find the velocity of approach:
v=√2G(m1+m2)r
The velocity of approach of the two masses when they are at a distance r apart is:
v=√2G(m1+m2)r
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Level 2 Single Correct
22 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Level 2 More Than One Correct
10 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Level 1 Single Correct
27 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
Similar Questions
Two particles of masses m1andm2 are intially at rest at an infinite distance apart. If they approach each other under their mutual interaction given by F=−Kr2. Their speed of approach at the instant when they are at a distance d apart is
View Solution10 Two particles of masses m1 and m2 initially at rest start moving towards each other under their mutual force of attraction. The speed of the centre of mass at any time t, when they are at a distance r apart, is
View SolutionTwo masses m1andm2 are placed at a distance r from each other. Find out the moment of inertia of system about an axis passing through their centre of mass.
View SolutionTwo particles of mass m and M are initialljy at rest at infinite distance. Find their relative velocity of approach due to gravitational attraction when d is their separation at any instant
View SolutionTwo particles of masses m and M are initially at rest at an infinite distance apart. They move towards each other and gain speeds due to gravitational attraction. Find their speeds when the separation between the masses becomes equal to d.
View SolutionBinary stars of comparable masses m1 and m2 rotate under the influence of each other's gravity with a time period T. If they are stopped suddenly in their motions, find their relative velocity when they collide with each constant of gravitation.
View SolutionTwo bodies of mass m1andm2 are initially at rest placed infinite distance apart. They are then allowed to move towards each other under mutual gravitational attraction. Show that their relative velocity of approach at separation r between them is:
View SolutionTwo points mass m and 2m are kept at a distance a. Find the speed of particles and their relative velocity of approach when separation becomes a/2.
View SolutionFind the force of interaction of two dipoles , if the two dipole moments are parallel to each other and placed at a distance x apart
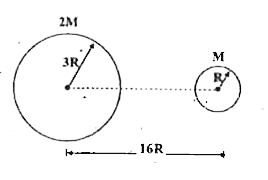
View SolutionTwo spherical bodies of masses 2M and M and radii 3R and R respectively are held at a distance 16R from each other in free space. When they are released they start approaching each other due to the gravitational force of attraction , then find
(a) the ratio of their accelerations during their motion
(b) their velocitites at the time of impact.
View Solution
