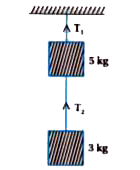
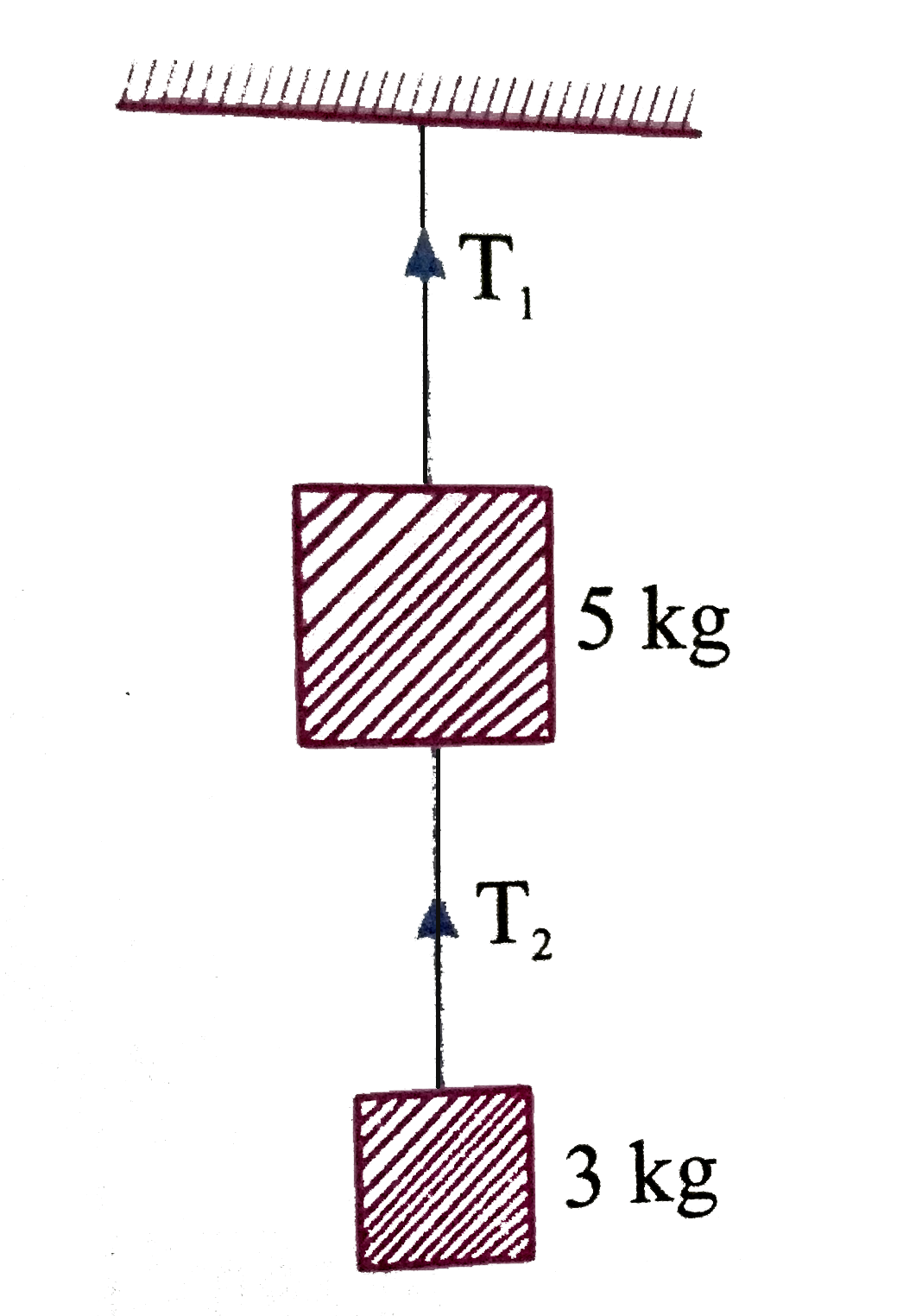
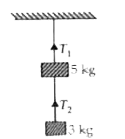
Two masses of 5 kg and 3 kg are suspended with help of massless inextensible strings as shown in figure. Calculate T1 and T2 when whole system is going upwards with acceleration = 2 ms2 2 (use g = 9.8 ms−2).
 Solution in Gujrati
Solution in Gujrati
Given m1=5kgm2=3kg
g=9.8m/s2 and a=m/s2
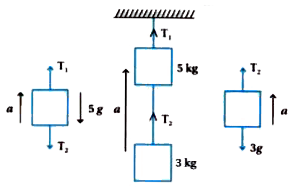
FBD force body diagram for block of 5 kg and 3 kg are shown in figure
For block of mass 5 kg
T1−T2−5g=5a
T1−T2=5g+5a
T1−T2=5(g+a)
For block of mass 3 kg
T2−3g=3a
T2=3g+3a
From equation (i )
T1−T2=5(g+a)
T2=T2+5(g+a)
=8(g+a)
=8(9.8+2)
=94.4N
Topper's Solved these Questions
- LAW OF MOTIONBOOK - KUMAR PRAKASHANCHAPTER - LAW OF MOTIONEXERCISE - SECTION -E(MULTIPLE CHOICE QUESTIONS) (MCQS)
69 Videos
- LAW OF MOTIONBOOK - KUMAR PRAKASHANCHAPTER - LAW OF MOTIONEXERCISE - SECTION-F (QUESTIONS FROM MODULE)
22 Videos
- LAW OF MOTIONBOOK - KUMAR PRAKASHANCHAPTER - LAW OF MOTIONEXERCISE - SECTION-C(OBJECTIVE QUESTIONS) (VSQS)
55 Videos
- GRAVITATIONBOOK - KUMAR PRAKASHANCHAPTER - GRAVITATIONEXERCISE - QUESTIONS PAPER Section - D
1 Videos
- MACHANICAL PROPERTIES OF SOLIDS BOOK - KUMAR PRAKASHANCHAPTER - MACHANICAL PROPERTIES OF SOLIDS EXERCISE - QUESTION PAPER
11 Videos
Similar Questions
The position (x) → time (t) graph of one dimensional motion of a body of mass 0.4 kg. What is the magnitude of impulse of force

View SolutionA block rests on a rough inclined plane making an angle of 30° with the horizontal. The coefficient of static friction between the block and the plane is 0.8. If the frictional force on the block is 10 N, the mass of the block ((in kg) is .......... (Take g = 10 m/s2)
View SolutionArea of F → t graph gives which physical quantity ?
View SolutionA spaceship is stationed on Mars. How much energy must be expended on the spaceship to launch it out of the solar system ? Mass of the spaceship = 1000 kg, mass of the sun =2×1030kg, mass of mars =6.4×1023kg, radius of mars = 3395 km, radius of the orbit of mars =2.28×108km,G=6.67×10−11Nm2kg2
View SolutionWhat is called brittle material ?
View SolutionIn the system shown in figure pully is smooth. Strings is massless and inextensible.Find acceleration of the system a, tension T_(1) and T_(2) (g=10m//s^(2))
View SolutionThe masses m_(1) m_(2) and m_(3) of the three bodies shown in fig . Are 5 , 2 and 3 kg respectively Calculate the valuse of tension T_(1) T_(2) and T_(3) when (i) the whole system is going upward with an acceleration of 2 m//s^(2) (ii) the whole system is stationary (g=9.8 m//s^(2)) . .
View SolutionTwo masses of 5 kg and 3 kg are suppended with help of massless inextensible strings as shown in Calculate T_(1) and T_(2) when whole system is going upwards with acceleration =2ms^(2) (us g= 9.8ms^(-2) ) .
View SolutionTwo masses of 5kg and 3kg are suspended with help of massless inextensible strings as shown in figure. Calculate T1and T2 when whole system is going upwards with acceleration =2m/s2(useg=9.8ms−2).
View SolutionThe figure shown below is a system of two masses 3 kg and 6 kg and massless pulley accelerating upwards with 2 m//s^(2) . If the value of T_(2) is (288)/(x)N , then the value of x is
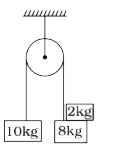
View SolutionA system is at rest as shown in figure. The tension in the string in T1. When the 2 kg block is removed, the system moves with some acceleration a and tension in string becomes T2 Find T1/T2 .
View SolutionTwo masses of 5 kg and 3 kg are suspended with help of mass less inextensible string as shown. Calculate T1andT2 when system is going upwards with acceleration m/s2.(Use g=9.8m/s2).
View SolutionThe masses M_(1), M_(2) and M_(3) are 5, 2 and 3 kg respectively. These have been joined using massless, inextensible pieces of strings as shown in the figure. If the whole system is going upward with an acceleration of 2ms^(-2) , then the value of tensions T_(1), T_(2) and T_(3) respectively are ["take "g=9.8ms^(-2)]
View Solutionचित्र में दर्शाए गए अनुसार 5 किग्रा व 3 किग्रा द्रव्यमानों को द्रव्यमानरहित अवितान्य डोरियों पर लटकाया गया है। सम्पूर्ण निकाय 2 मी से−2 के त्वरण से ऊपर की ओर जा रहा है। T1 व T2 तनाव क्रमश: हैं -
(g = 10 मी/सेकण्ड2)
View SolutionTwo masses of 5kg and 3kg are suspended with help of massless inextensible strings as shown in figure. Calculate T1and T2 when whole system is going upwards with acceleration =2m/s2(useg=9.8ms−2).

View Solution
