Two nicol prisms are inclined to each other at an angle 30∘. If I is the intensity of ordinary light incident on the first prism, then the intensity of light emerges from the second prism will be
3I/4
I/2
I/4
3I/8
The correct Answer is:D
To solve the problem of finding the intensity of light emerging from the second Nicol prism when two prisms are inclined at an angle of 30∘, we can follow these steps:
1. Understand the Problem: We have two Nicol prisms, and ordinary light of intensity I is incident on the first prism. We need to find the intensity of light I0 that emerges from the second prism after passing through the first.
2. Use the Intensity Relation: The relationship between the intensity of the incident light I and the emerging light I0 after passing through a Nicol prism inclined at an angle θ is given by:
I0I=12cos2θ
3. Substitute the Angle: In our case, the angle θ is 30∘. Therefore, we substitute θ into the equation:
I0I=12cos2(30∘)
4. Calculate cos(30∘): We know that:
cos(30∘)=√32
Thus,
cos2(30∘)=(√32)2=34
5. Substitute Back into the Intensity Relation: Now we substitute cos2(30∘) back into the intensity equation:
I0I=12⋅34=38
6. Find the Emerging Intensity: Now, we can express I0 in terms of I:
I0=I⋅38
7. Final Result: Therefore, the intensity of light emerging from the second Nicol prism is:
I0=3I8
Conclusion:
The intensity of light that emerges from the second Nicol prism is 3I8.
---
I0I=12cos2θ
I0I=12cos2(30∘)
cos(30∘)=√32
Thus,
cos2(30∘)=(√32)2=34
I0I=12⋅34=38
I0=I⋅38
I0=3I8
The intensity of light that emerges from the second Nicol prism is 3I8.
Topper's Solved these Questions
Similar Questions
The polariser and analyser are inclined to each other at 60∘. If I/2 is the intensity of the polarised light emergent from analyser. Then the intensity of the unpolarised light incident on the polariser is
View SolutionThe polariser and analyser are inclined to each other at 60∘. The intensity of polarised light emerging from polariser is I. The intensity of the unpolarised light incident on the polariser is
View SolutionWhat is the required condition, if the light incident on one face of a prism, does not emerge from the other face?
View SolutionAn analyser is inclined to a polariser at an angle of 30∘. The intensity of light emerging from the analyser is 1/nth of that is incident on the polariser. Then n is equal to
View SolutionAn analyser is inclined to a polariser at an angle of 30∘. The intensity of light emerging from the analyser is 1/nth of that is incident on the polariser. Then n is equal to
View SolutionTwo polaroids are placed such that their planes are parallel to each other with their access of transmission at 30°. If an unpolarised light of intensity I0 is incident on first polaroid polarizer then intensity of light that will be transmitted through the second polaroid is
View SolutionA beam of white light is incident on a hollow prism of glass. Then

View SolutionA direct-vision prism is made out of three prisms, each with a refracting angle of ϕ=60∘ , attached to each other as shown in Figure. Light of a certain wavelength is incident on the first prism. The angle of incidence is 30∘ and the ray leaves the third prism parallel to the direction of incidence. The refractive index of the glass of the first and third prisms, is 1.5. Find the refractive index of the material of the middle prism. (√6=2.45)
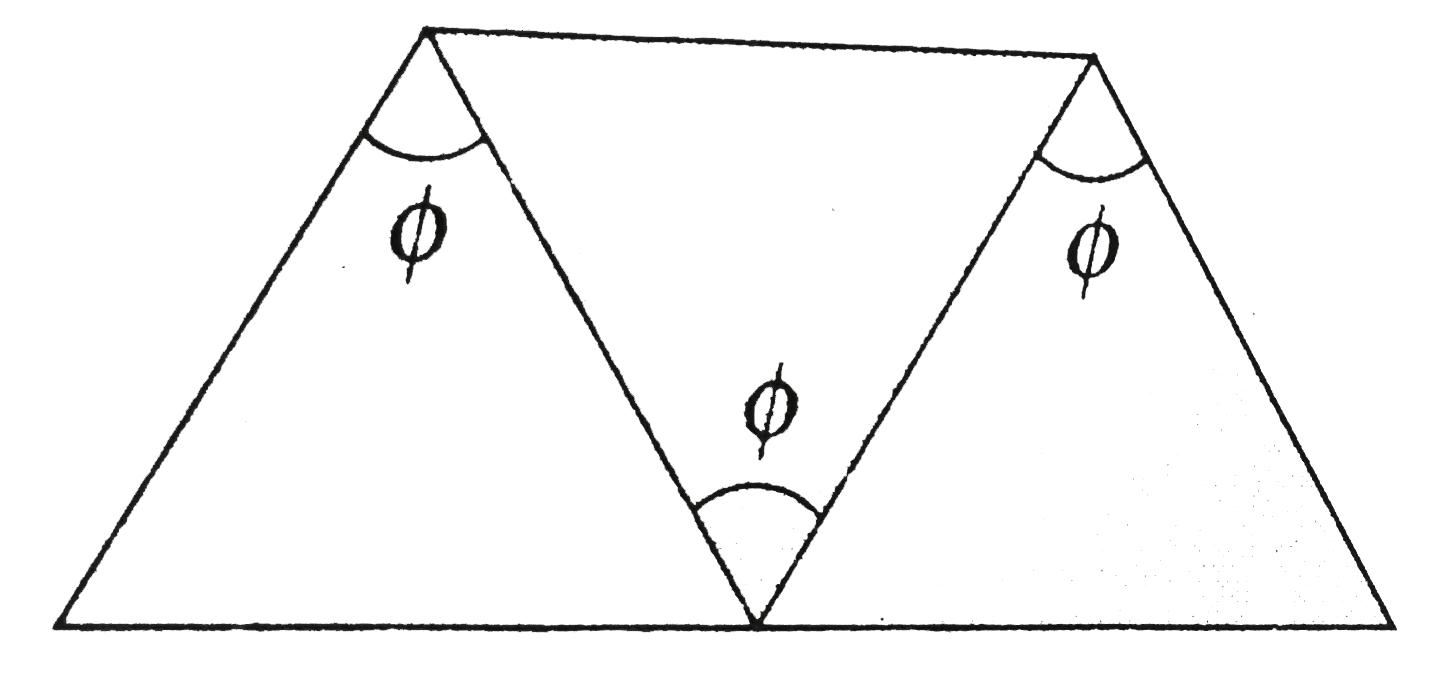
View SolutionLight is incident on a polarizer with intensity I0. A second prism called analyzer is kept at a angle of 15∘, from the first polarizer then the intensity of final emergent light will be

View SolutionA : Nicol prism is used to produce and analyse plane polarised light.
R : Nicol prism reduces the intensity of light to zero.
View Solution
