Two particles, 1 and 2, move with constant velocities v1 and v2. At the initial moment their radius vectors are equal to r1 and r2. How must these four vectors be interrelated for the particles to collide?
r1−r2|r1−r2|=v2−v1|v2−v1|
r2.v1=r2.r2
r1×v1=r2×v2
r1×v1=v1−v2
The correct Answer is:a
For two particle A and B move with constant velocity v1and v2 Such that two particle to collide, the direction of the velocity towards the relative position of the other particle
i.e r2−r2|r1−r2|→direction of relative position of 1w.r.t 2
Similarly,v1−v2|v1−v2|→ direction of velocity of 2 w.r.t. 1 So, for collision of A and B we get
r1−r2|r1−r2|=v2−v1|v2−v1|
Alternate Method As resultant displacement of a particle

i.e R=r1−v1t=r2−v2t
r1−r2=(v2−v1)t
r1−r2|r1−r2|=v2−v1t|v2−v1|t
r1−r2|r1−r2|=v2−v1|v2−v1|
Topper's Solved these Questions
Similar Questions
Two particles, 1 and 2, move with constant velocities v1 and v2 along two mutually perpendicular straight lines toward the intersection point O. At the moment t=0 the particles were located at the distances l1 and l2 from the point O. How soon will the distance between the particles become the smallest? What is it equal to?
View SolutionTwo particles 1and2 move with velocities →v1 and →v2 making the angles θ1andθ2 with the line joining them, respectively. Find angular velocity of relative→1.
 .
.
View Solution
Knowledge Check
Two particle A and B, move with constant velocities →v1and→v2. At the initial moment their position vectors are →r1 and →r2 respectively . The condition for particles A and B for their collision is
A→r1×→v1=→r2×→v2B→r1−→r2=→v1−→v2C→r1−→r2∣∣→r1−→r2∣∣=→v2−→v1∣∣→v2−→v1∣∣D→r1.→v1=→r2.→v2Two particles A and B, move with constant velocities →v1 and →v2. At the initial moment their position vectors are →r1 and →r2 respectively. The condition for particle A and B for their collision is
A→r1−→r2=→v1−→v2B→r1−→r2∣∣→r1−→r2∣∣=→v2−→v1∣∣→v2−→v1∣∣C→r1.→v1=→r2.→v2D→r1×→v1=→r2×→v2Two particles are moving with velocities v1andv2 . Their relative velocity is the maximum, when the angle between their velocities is
AzeroBπ/4Cπ/2Dπ
Two particles AandB are moving with constant velocities v1andv2. At t=0, v1 makes an angle θ0 with the line joining AandB and v2 makes an angle θ2 with the line joining AandB. Find their velocity of approach.
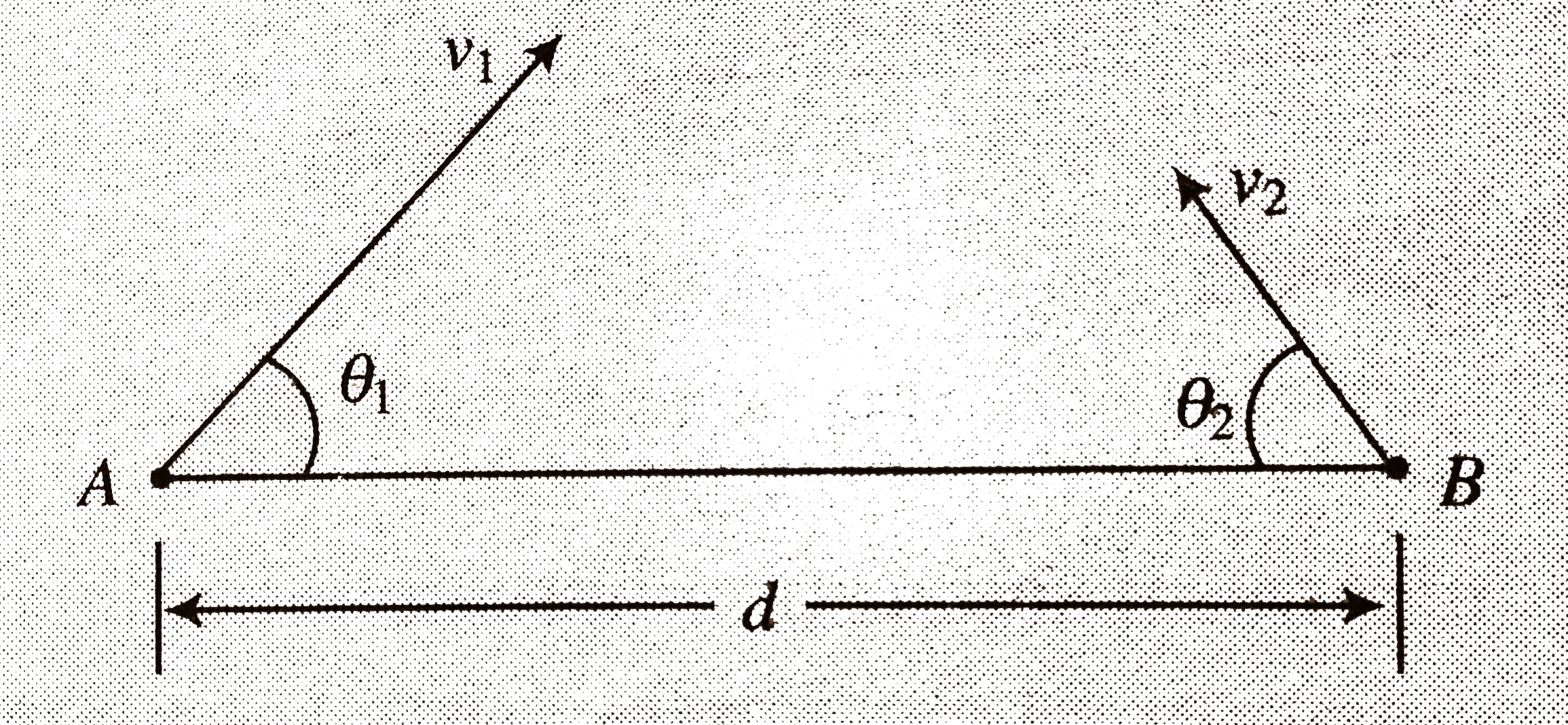 .
.
View SolutionA closed system consists of two particles of masses m1 and m2 which move at right angles to each other with velocities v1 and v2. Find:
(a) the momentum of each particle and
(b) the total kinetic energy of the two particles in the reference frame fixed to their centre of inertia.
View SolutionTwo point masses 1 and 2 move with uniform velocities →v1 and →v2, respectively. Their initial position vectors are →r1 and →r2, respectively. Which of the following should be satisfied for the collision of the point masses?
View SolutionTwo particles of masses m1,m2 move with initial velocities u1andu2. On collision , one of the particles get excited to higher level , after absorbing energy ε. If final velocities of particles be v1 and v2 then we must have
View SolutionTwo particles of mass m and 2 m moving in opposite directions collide elastically with velocities v and 2v. Find their velocities after collision.
View Solution
