Two particles are shown in figure. At a constant force starts acting on . Find the velocity of circle of mass of these particle at .
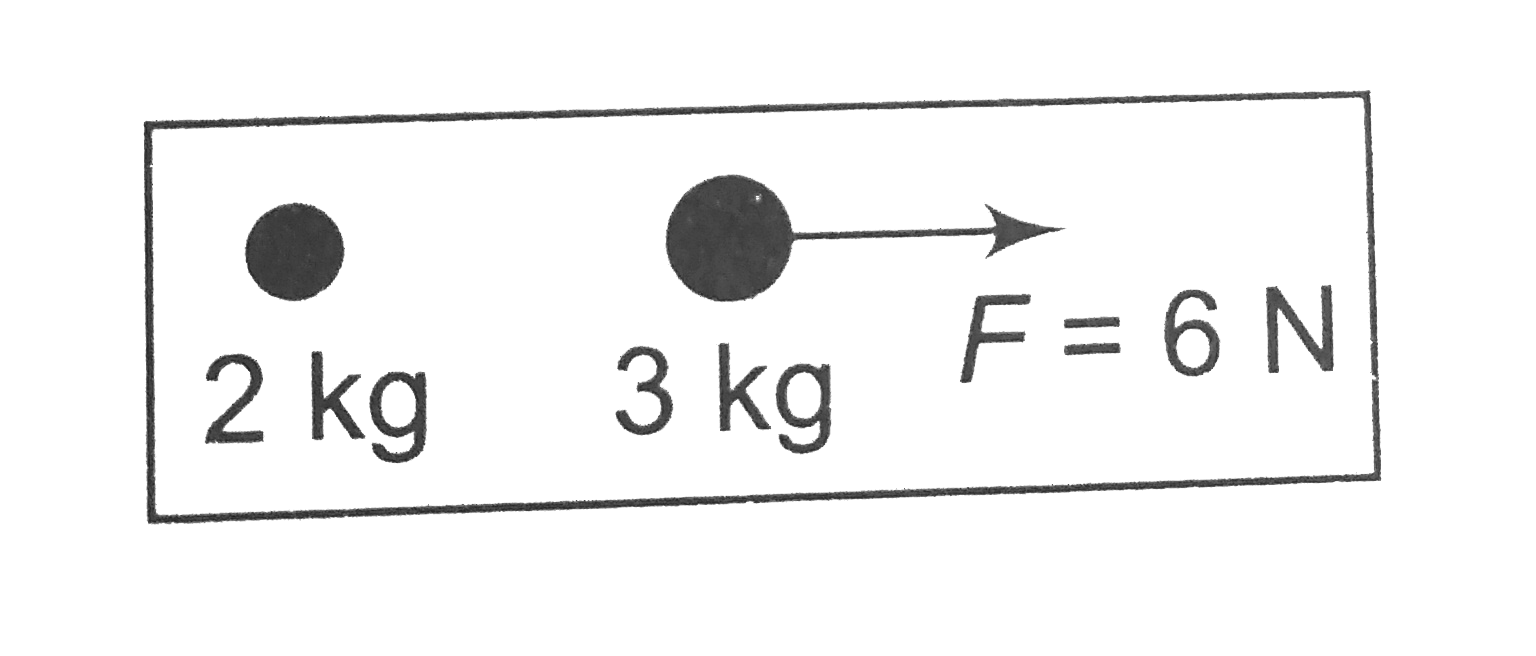 .
.
The correct Answer is:C
( c) particle remains at rest. So
for particle :
Velocity at :
.
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Moment Of Inertia39videos
ROTATIONAL DYNAMICS
- BOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Rotational Kinematics19videos
ROTATIONAL DYNAMICS
- BOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Chapter Test29videos
ROTATIONAL DYNAMICS
- BOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Chapter Test29videos
PROPERTIES OF MATTER
- BOOK - A2ZCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Chapter Test30videos
THERMAL PROPERTIES OF MATTER
Similar Questions
Knowledge Check
- Question 1 - Select One or More
A particle is resting over a smooth horizontal floor AT t = 0, a horizontal force starts acting on it. Magnitude of the force increases with time F = kt, where k is a constant. The two curves are drawn for this particle as shown
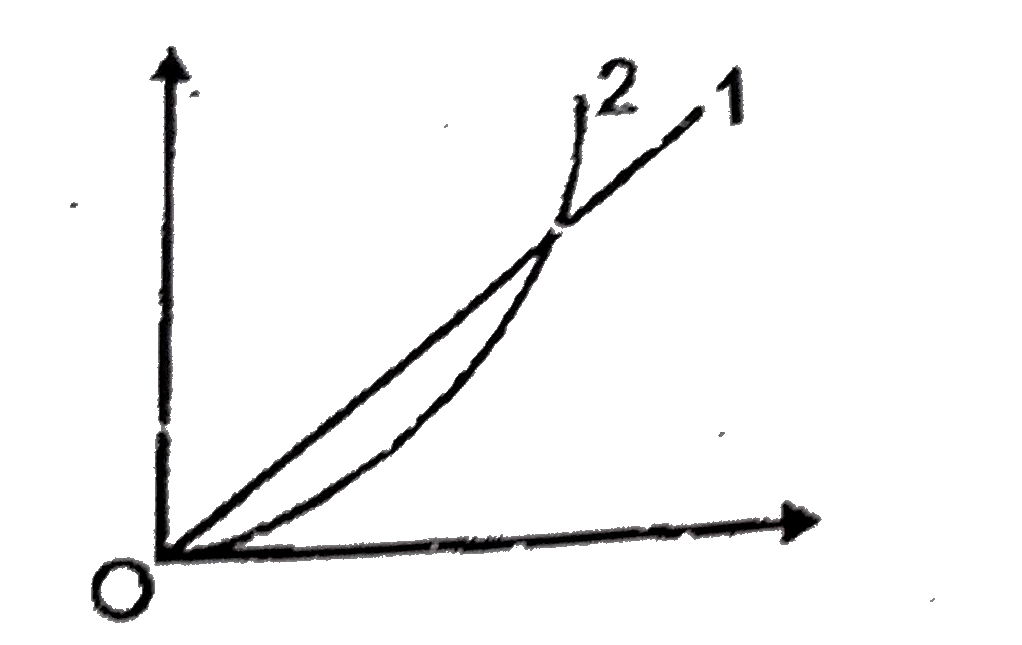 ACurve-1 shows acceleration versus timeBCurve-2 shows velocity versus timeCCurve-2 shows velocity versus accelerationDCurve-1 shows velocity versus acceleration
ACurve-1 shows acceleration versus timeBCurve-2 shows velocity versus timeCCurve-2 shows velocity versus accelerationDCurve-1 shows velocity versus acceleration - Question 2 - Select One
At t=0, the positions and velocities of two particles are as shown in the figure. They are kept on a smooth surface and being mutually attracted by gravitational force. Find the position of centre of mass at t=2s.
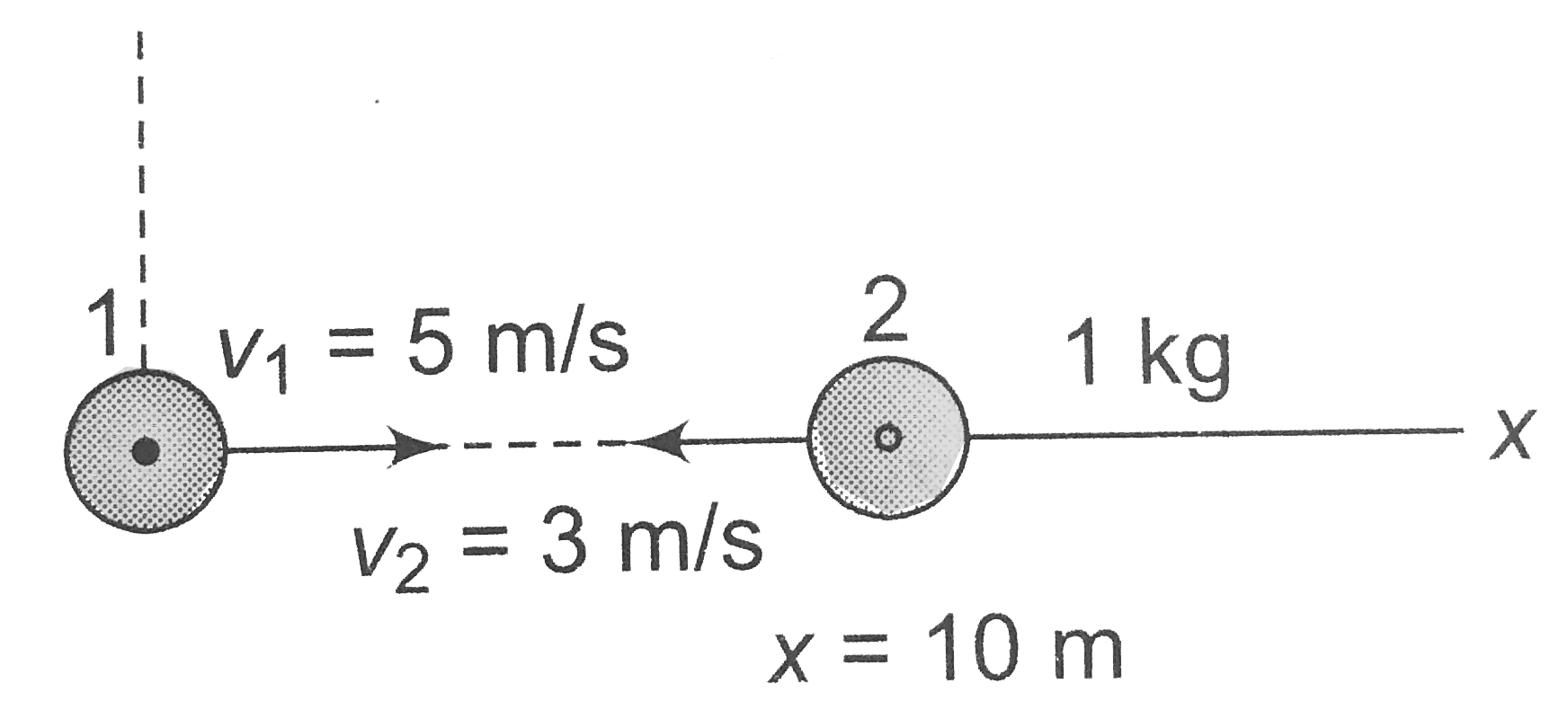 .AX = 5 mBX = 7 mCX = 3 mDX = 2 m
.AX = 5 mBX = 7 mCX = 3 mDX = 2 m - Question 3 - Select One
A particle is resting over a smooth horizontal floor. At t=0, a horizontal force starts acting on it. Magnitude of the force increases with the time according to law F=at, where 'a' is a constant. For figure which of the following statement is/are correct ?
 ACurve B indicates velocity against timeBCurve B indicates velocity against accelerationCCurve A indicates acceleration against timeDNone of these
ACurve B indicates velocity against timeBCurve B indicates velocity against accelerationCCurve A indicates acceleration against timeDNone of these
The acceleration-time graph of a particle moving along x-axis is shown in the figure. If the particle starts with velocity 3 m/s at t = 0, find the velocity of particle at t = 4 s.
 View Solution
View SolutionThe acceleration of a particle varies with time t as
a=t2+t+2
where t is in seconds.
The particle starts with an initial velocity v-3m/s at t=0. find the velocity of the particle at the end of 5s.View SolutionA particle of m=5kg is momentarily at rest at x=att=. It is acted upon by two forces →F1 and →F2. Vec(F)1=70ˆj N. The direction and manitude of →F2 are unknown. The particle experiences a constant acceleration, →a,in the direction as shown in (figure) Neglect gravity.
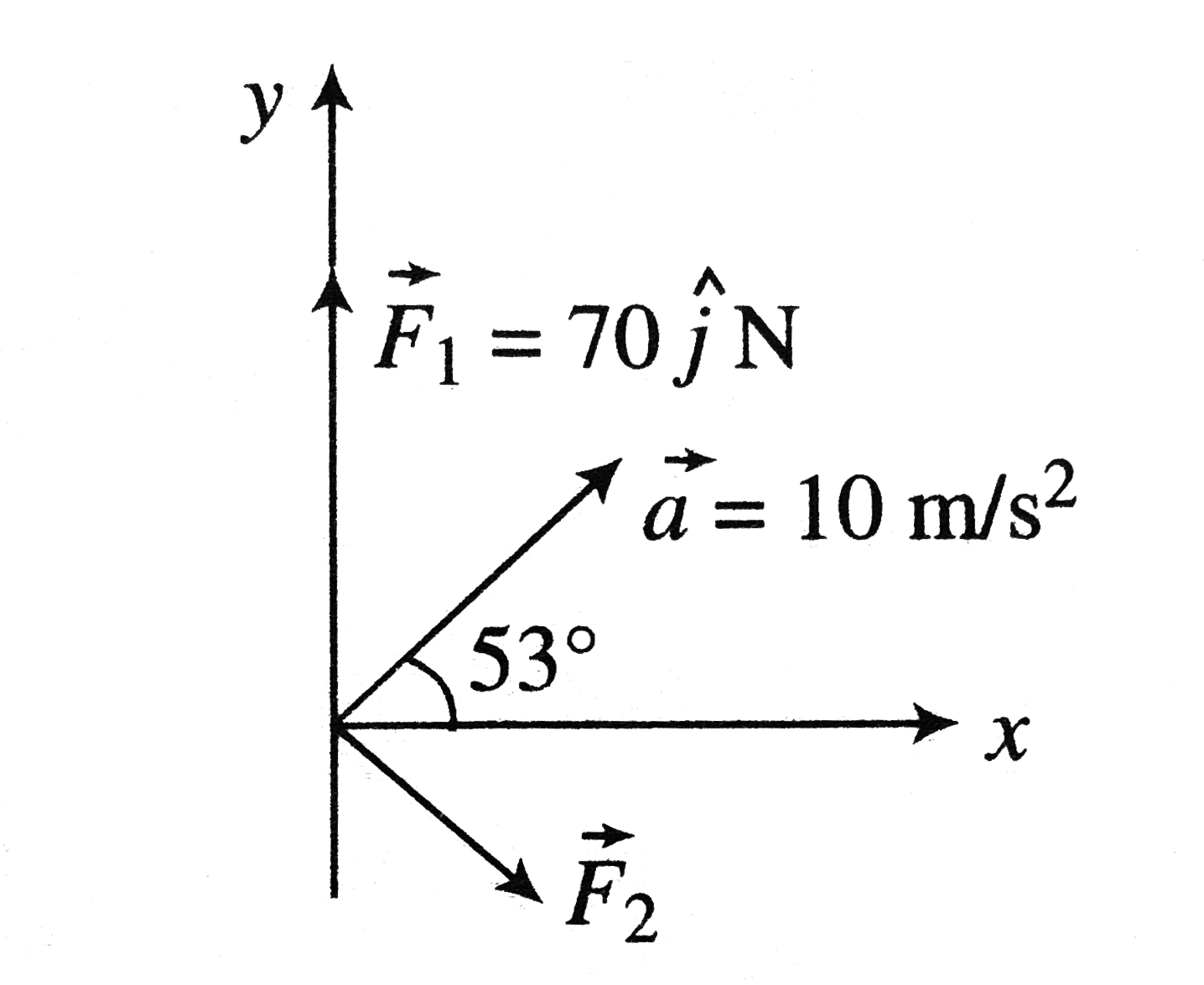
a.Find the missing force →F2.
b. What is the velocity vector of the particle at t=10s?
c. What third force, →F3 is required to make the acceleration of the particle zero? Either give magnitude and direction of →F3 or its components.View SolutionStarting from rest, acceleration of a particle is a=2t(t−1). The velocity of the particle at t=5s is
View SolutionA particle of mass 2kg is initially at rest. A force starts acting on it in one direction whose magnitude changes with time. The force time graph is shown in figure. Find the velocity of the particle at the end of 10s.
 View Solution
View Solution

