Two particles of mass 0.5 kg each go round a circle of radius 20 cm on accound of their mutual gravitational attraction. What will be the speed of each particle ?
The correct Answer is:6.45×10−6m/s
To find the speed of each particle in a circular motion due to their mutual gravitational attraction, we can follow these steps:
Step 1: Understand the System
We have two particles, each with a mass m=0.5kg, moving in a circular path of radius r=20cm=0.2m. The gravitational force between them provides the necessary centripetal force for their circular motion.
Step 2: Identify the Forces
The gravitational force Fg between the two particles can be expressed using Newton's law of gravitation:
Fg=Gm1m2d2
where G=6.67×10−11N m2/kg2 is the gravitational constant, and d is the distance between the two particles.
Step 3: Calculate the Distance Between the Particles
Since both particles are at a distance of r from the center of mass (which is at the midpoint between them), the total distance d between the two particles is:
d=2r=2×0.2m=0.4m
Step 4: Set Up the Equation for Centripetal Force
The centripetal force Fc required to keep each particle in circular motion is given by:
Fc=mv2r
where v is the speed of the particle.
Step 5: Equate Gravitational Force and Centripetal Force
Since the gravitational force provides the centripetal force, we can set them equal:
Gm2(2r)2=mv2r
Step 6: Simplify the Equation
Substituting d=2r into the gravitational force equation:
Gm24r2=mv2r
Now, we can cancel one m from both sides (since m≠0):
Gm4r2=v2r
Step 7: Rearrange to Solve for v2
Multiplying both sides by r:
Gm4r=v2
Step 8: Solve for v
Taking the square root gives us:
v=√Gm4r
Step 9: Substitute the Values
Now we substitute the known values into the equation:
- G=6.67×10−11N m2/kg2
- m=0.5kg
- r=0.2m
v=√6.67×10−11×0.54×0.2
Step 10: Calculate the Speed
Calculating the above expression:
v=√6.67×10−11×0.50.8=√3.335×10−110.8=√4.16875×10−11≈6.45×10−6m/s
Thus, the speed of each particle is approximately 6.45×10−6m/s.
We have two particles, each with a mass m=0.5kg, moving in a circular path of radius r=20cm=0.2m. The gravitational force between them provides the necessary centripetal force for their circular motion.
The gravitational force Fg between the two particles can be expressed using Newton's law of gravitation:
Fg=Gm1m2d2
where G=6.67×10−11N m2/kg2 is the gravitational constant, and d is the distance between the two particles.
Since both particles are at a distance of r from the center of mass (which is at the midpoint between them), the total distance d between the two particles is:
d=2r=2×0.2m=0.4m
The centripetal force Fc required to keep each particle in circular motion is given by:
Fc=mv2r
where v is the speed of the particle.
Since the gravitational force provides the centripetal force, we can set them equal:
Gm2(2r)2=mv2r
Substituting d=2r into the gravitational force equation:
Gm24r2=mv2r
Now, we can cancel one m from both sides (since m≠0):
Gm4r2=v2r
Multiplying both sides by r:
Gm4r=v2
Taking the square root gives us:
v=√Gm4r
Now we substitute the known values into the equation:
- G=6.67×10−11N m2/kg2
- m=0.5kg
- r=0.2m
Calculating the above expression:
v=√6.67×10−11×0.50.8=√3.335×10−110.8=√4.16875×10−11≈6.45×10−6m/s
Topper's Solved these Questions
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Conceptual Questions
19 Videos
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Tough & Tricky Problems
10 Videos
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Chapter Practice Test
15 Videos
- MATHEMATICAL TOOLSBOOK - MODERN PUBLICATIONCHAPTER - MATHEMATICAL TOOLSEXERCISE - PRACTICE PROBLEMS (10)
12 Videos
Similar Questions
_S01_003_Q01.png)
Two particles of equal mass (m) each move in a circle of radius (r) under the action of their mutual gravitational attraction find the speed of each particle.
View SolutionTwo particles of equal mass go round a circle of radius R under the action of their mutual gravitational attraction. Find the speed of each particle.
View Solution
Knowledge Check
Two identical particles of mass 1 kg each go round a circle of radius R, under the action of their mutual gravitational attraction. The angular speed of each particle is :
A√G2R3B12√GR3C12R√1GD√2GR3Two particles of equal mass m go around a circle of radius R under the action the of their mutual gravitational attraction . The speed v of each particle is
A12√GmRB√4GMRC12R√1GmD√Gm2RTwo particles of equal mass m go around a circle of radius R under action of their mutual gravitational attraction. The speed of each particle with respect to their centre of mass is
A√GmRB√Gm4RC√Gm3RD√Gm2R
Two particles of equal masses m go round a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is v=√GmnR. Find the value of n.
View SolutionTwo particles of equal mass (m) move in a circle of radius (r ) under the action of their mutual gravitational attraction. Find the speed of each particle.
View SolutionFour particles each of mass M, move along a circle of radius R under the action of their mutual gravitational attraction as shown in figure. The speed of each particle is :
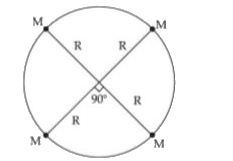
View SolutionTwo particles of equal mass 'm' go around a circle of radius R under the action of their mutual gravitaitonal attraction. The speed of each particle with respect to their centre of a mass is -
View SolutionTwo particles of same mass m go around a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is ,
View Solution
