Two point prticles $A$ and $B$ are placed in line on frictionless horizontal plane. If particle $A$ (mass $1 k g$ ) is move with velocity $10 m / s$ towards stationary particle $B$ (mass $2 k g$ ) and after collision the two move at an angle of $45^{\circ}$ with the initial direction of motion, then find

a. velocites of $A$ and $B$ just after collision.
b. coefficient of restitution

Video Solution

Text Solution

Verified by Experts

The very first step to solve such problems is to find the line of impact which is along the direction of force applied by $A$ on $B$ , resulting the stationary particle $B$ to move. Thus, by watching the direction of motion of $B$ , line of impact can be determined. in this case, the line of impact is along the direction of motion of $B$ i.e. $45^{\circ}$ with the initial diretion of motion of $A$ .
a. Let us apply the principle of conservation of momentum.
Along $x -$ direction:
$m_{A} u_{A} = m_{A} v_{A} {cos 45}^{\circ} m_{B} v_{B} {cos 45}^{\circ}$
or $1 (10) = 1 (v_{A} {cos 45}^{\circ}) + 2 (v_{B} {cos 45}^{\circ})$
or $v_{A} + 2 v_{B} = 10 \sqrt{2})$ ..........i
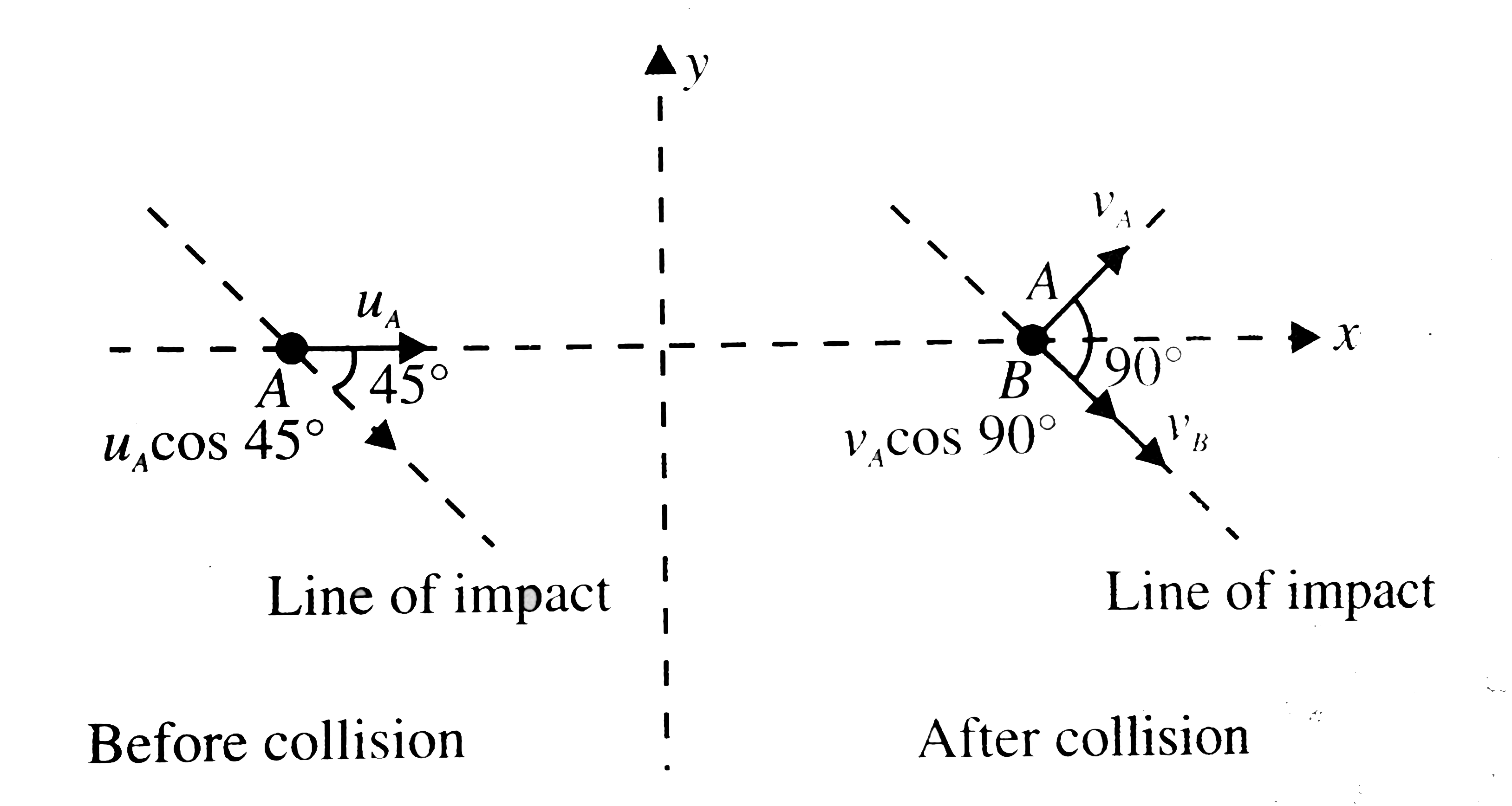Along $y$ direction
$0 = m_{A} v_{A} {sin 45}^{\circ} - m_{B} v_{B} {sin 45}^{\circ}$
or $0 = 1 (v_{A} {sin 45}^{\circ}) - 2 (v_{B} {sin 45}^{\circ})$
or $v_{A} = 2 v_{B}$
Solving the two equation $v_{A} = \frac{10}{\sqrt{2}} m / s$ and $v_{B} = \frac{5}{\sqrt{2}} m / s$
b. coefficient of restitution
$e = \frac{velocity of separation along line of impact}{velocity of approach along line of impact}$
$= \frac{v_{B} - v_{A} {cos 90}^{\circ}}{u_{A} {cos 45}^{\circ}} = \frac{\frac{5}{\sqrt{2}} - 0}{\frac{10}{\sqrt{2}}} = \frac{1}{2}$
hence, $P$ is constant.

Updated on:21/07/2023