Two satellites S1 and S2 are revolving round a planet in coplanar and concentric circular orbit of radii R1 and R2 in te same direction respectively. Their respective periods of revolution are 1 hr and 8 hr. the radius of the orbit of satellite S1 is equal to 104km. Find the relative speed in kmph when they are closest.
π/2×104
π×104
2π×104
4π×104
The correct Answer is:B
To solve the problem step by step, we will follow these guidelines:
Step 1: Identify Given Values
We have the following information:
- Period of satellite S1 (T1) = 1 hour
- Period of satellite S2 (T2) = 8 hours
- Radius of orbit of satellite S1 (R1) = 104 km
Step 2: Use Kepler's Third Law to Find R2
According to Kepler's Third Law, the square of the period of revolution of a satellite is proportional to the cube of the radius of its orbit. This can be expressed as:
T21T22=R31R32
Substituting the known values:
1282=(104)3R32
164=1012R32
Cross-multiplying gives:
R32=64×1012
Taking the cube root:
R2=√(3)64×104=4×104 km
Step 3: Calculate the Speeds of the Satellites
The speed of a satellite in circular orbit is given by the formula:
V=Rω
where ω=2πT.
For Satellite S1:
V1=R1⋅ω1=R1⋅2πT1
Substituting the known values:
V1=104⋅2π1=2π×104 km/hr
For Satellite S2:
V2=R2⋅ω2=R2⋅2πT2
Substituting the known values:
V2=4×104⋅2π8=2π×4×1048=π×104 km/hr
Step 4: Calculate the Relative Speed
The relative speed when the satellites are closest is given by:
Vrelative=V1−V2
Substituting the values we calculated:
Vrelative=(2π×104)−(π×104)=π×104 km/hr
Final Answer
The relative speed when the satellites are closest is:
π×104 km/hr
---
We have the following information:
- Period of satellite S1 (T1) = 1 hour
- Period of satellite S2 (T2) = 8 hours
- Radius of orbit of satellite S1 (R1) = 104 km
According to Kepler's Third Law, the square of the period of revolution of a satellite is proportional to the cube of the radius of its orbit. This can be expressed as:
T21T22=R31R32
1282=(104)3R32
164=1012R32
R32=64×1012
Taking the cube root:
R2=√(3)64×104=4×104 km
The speed of a satellite in circular orbit is given by the formula:
V=Rω
where ω=2πT.
V1=R1⋅ω1=R1⋅2πT1
Substituting the known values:
V1=104⋅2π1=2π×104 km/hr
V2=R2⋅ω2=R2⋅2πT2
Substituting the known values:
V2=4×104⋅2π8=2π×4×1048=π×104 km/hr
The relative speed when the satellites are closest is given by:
Vrelative=V1−V2
Substituting the values we calculated:
Vrelative=(2π×104)−(π×104)=π×104 km/hr
The relative speed when the satellites are closest is:
π×104 km/hr
Topper's Solved these Questions
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved Papers 2017(JIPMER)
28 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(NEET)
22 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Integer type questions
14 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Exercise 19.7
4 Videos
Similar Questions
Two satellities s1 a & s2 of equal masses revolves in the same sense around a heavy planet in coplaner circular orbit of radii R & 4R
View SolutionTwo satellites S1 and S2 revolve round a planet in coplaner circular orbit in the same sense. Their period of revolution are 1 hour and 8 hour respectively. The radius of the orbit of S1 is 104km. When S2 is closest to S1, find
(a) The speed of S2 relative to S1,
(b) The angular speed of S2 actually observed by an astronaut is S1
View Solution
Knowledge Check
Two satellites S1 and S2 revolve around a planet in coplanar circular orbits in the same sense their periods of revolution are 1 hour and 8hours respectively the radius of the orbit of S1 is 104 km when S1 is closest to S2 the angular speed of S2 as observed by an astronaut in S1 is :
Aπ×104B−π×104Cπ×105Dπ×105
Two satellite S1andS2 revolve round a planet in coplanar circular orbits in the same sense. Their periods of revolutions are 1 h nd 8 h respectively. The radius of the orbit of S1 is 104km. When S2 is closet to S1., find (a). The speed of S2 relative to S1 and (b). the angular speed of S2 as observed by an astronaut in S1.
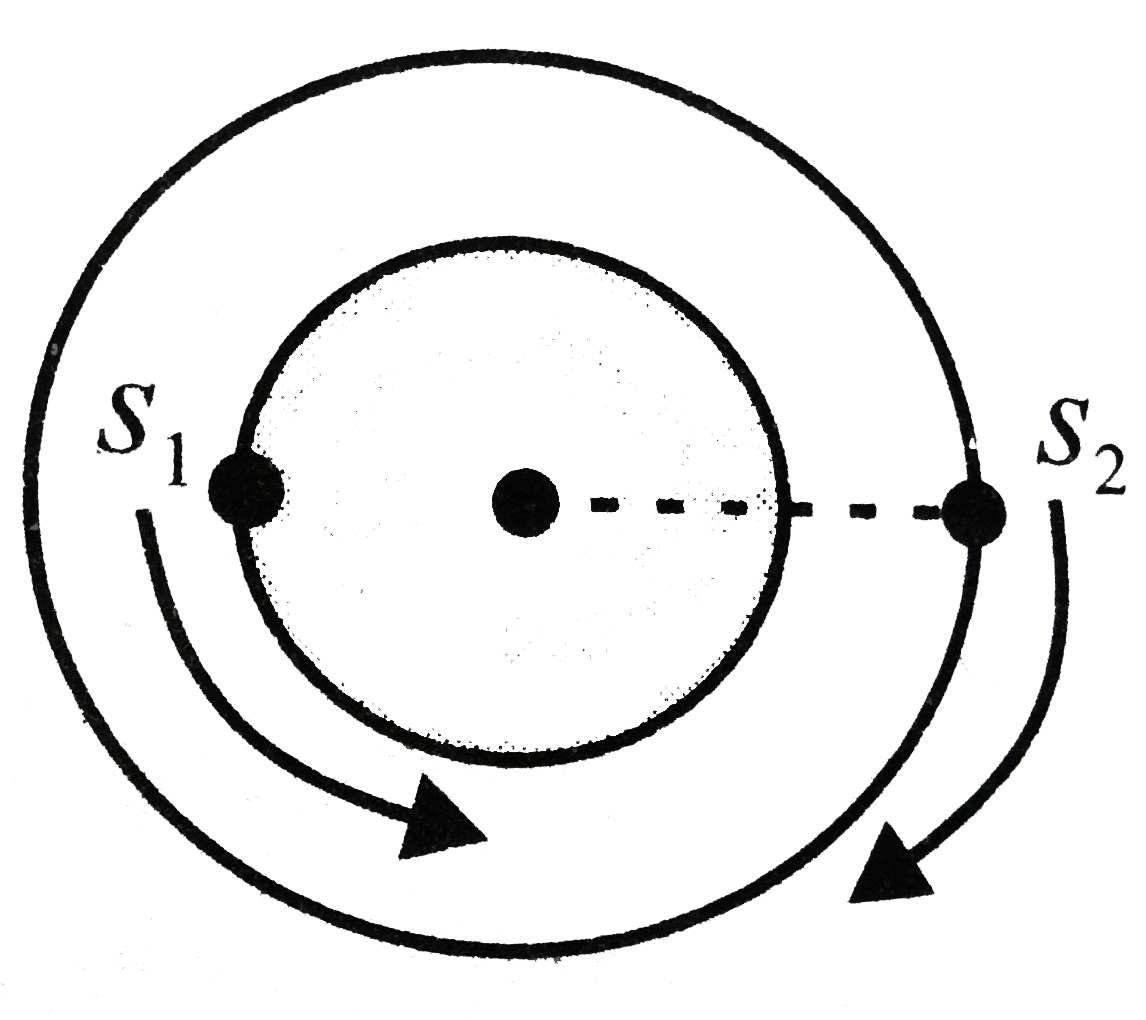
View SolutionTwo satellites S1 and S2 are revolving around the earth in coplanar concentric orbits in the opposite sense. At t=0, the position of satellites are shown in the diagram. The periods of S1 and S2 are 4h and 24h, respectively. The radius of orbit of S1 is 1.28×104km. For this situation, mark the correct statement (s).
View SolutionTwo satellites of masses of m1 and m2(m1>m2) are revolving round the earth in circular orbits of radius r1 and r2(r1>r2) respectively. Which of the following statements is true regarding their speeds v1 and v2?
View SolutionTwo satellites A and B revolve around a plant in two coplanar circular orbits in the same sense with radii 104km and 2×104km respectively. Time period of A is 28 hours. What is time period of another satellite?
View SolutionTwo satellites A and B go around a planet in circular orbits of radii 4 R and R respectively. If the speed of the satellite A is 3 V, then the speed of the satellite B will be
View SolutionTwo satellites P and Q go round a planet in circular orbits of radii 9R and R respectively. If the speed of the satellite P is 4V, then speed of satellite Q will be
View SolutionTwo satellites A and B of masses m1 and m2(m1=2m2) are moving in circular orbits of radii r1 and r2(r1=4r2), respectively, around the earth. If their periods are TA and TB, then the ratio TA/TB is
View Solution
