Two separated sources emit sinusoidal travelling waves but have the same wavelength λ and are in phase at their respective sources . One travels a distance l1 to get to the observeration point while the other travels a distance l2 . The amplitude is minimum at the observation point , l1−l2 is an
odd integral multiple of λ
even integral multiple of λ
odd integral multiple of λ/2
odd integral multiple of λ/4
The correct Answer is:C
To solve the problem, we need to analyze the conditions for destructive interference of two waves emitted from separate sources. The key points to consider are the path lengths traveled by the waves and the conditions for minimum amplitude at the observation point.
1. Understanding the Problem:
We have two sources emitting waves that are in phase. The distances traveled by the waves to the observation point are l1 and l2. The difference in these distances is Δl=l1−l2.
Hint: Identify the path difference between the two waves.
2. Condition for Minimum Amplitude:
The problem states that the amplitude is minimum at the observation point. This indicates that the waves are interfering destructively.
Hint: Recall the conditions for constructive and destructive interference.
3. Destructive Interference Condition:
For destructive interference to occur, the path difference must be an odd multiple of half the wavelength. Mathematically, this can be expressed as:
Δl=l1−l2=(2n−1)λ2
where n is an integer (0, 1, 2, ...).
Hint: Remember that n can take any integer value, and the factor (2n−1) ensures that the path difference is odd.
4. Conclusion:
Since the amplitude is minimum at the observation point, we conclude that the path difference l1−l2 must be an odd integral multiple of λ2. Therefore, the answer to the question is:
l1−l2=(2n−1)λ2
Hint: Verify that the answer matches the conditions for destructive interference.
Final Answer:
The path difference l1−l2 is an odd integral multiple of λ2.
We have two sources emitting waves that are in phase. The distances traveled by the waves to the observation point are l1 and l2. The difference in these distances is Δl=l1−l2.
The problem states that the amplitude is minimum at the observation point. This indicates that the waves are interfering destructively.
For destructive interference to occur, the path difference must be an odd multiple of half the wavelength. Mathematically, this can be expressed as:
Δl=l1−l2=(2n−1)λ2
where n is an integer (0, 1, 2, ...).
Since the amplitude is minimum at the observation point, we conclude that the path difference l1−l2 must be an odd integral multiple of λ2. Therefore, the answer to the question is:
l1−l2=(2n−1)λ2
The path difference l1−l2 is an odd integral multiple of λ2.
Topper's Solved these Questions
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Multiple
26 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Assertion - Reasoning
6 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Subjective
24 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 24
1 Videos
Similar Questions
Knowledge Check
A vehicle travels half the distance (L) with speed V1 and the other half with speed V2, then its average speed is .
Aa. v1+v22Bb. 2v1+v2v1+v2Cc. 2v1v2v1+v2Dd. v1+v2v1v2
A vehicle travels half the distance (L) with speed V1 and the other half with speed V2, then its average speed is .
View SolutionTwo interferring waves have the same wavelength, frequency and amplitude. They are travelling in the same direction but 90∘ out of phase compared to individual waves. The resultant wave will have the same.
View SolutionTwo light waves having the same wavelength λ in vacuum are in phase initially. Then the first ray travels a path of length L1 through a medium of refractive index μ1. Then second ray travels a path of length L2 throug a medium of refractive index μ2. The two waves are then combined to observed interference effects. The phase difference between the two, when they interfere, is
View SolutionTwo light waves having the same wavelength λ in vacuum are in phase initially. Then the first ray travels a path of length L1 through a medium of refractive index μ1. Then second ray travels a path of length L2 throug a medium of refractive index μ2. The two waves are then combined to observed interference effects. The phase difference between the two, when they interfere, is
View SolutionTwo coherent sources separated by distance d are radiating in phase having wavelength λ. A detector moves in a big circle around the two sources in the plane of the two sources. The angular position of n=4 interference maxima is given as

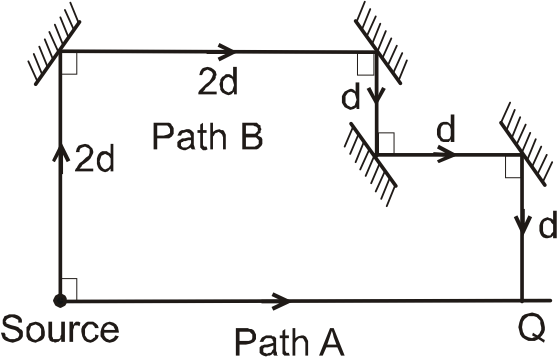
View SolutionA sound source emits two sinusoidal sound waves, both of wavelength λ, along paths A and B as shown in figure.The sound travelling along path B is reflected from five surfaces as shown and then merges at point Q, producing minimum intensity at that point.The minimum value of d in terms of λ is :
View SolutionTwo sinusoidal wave having the same frequency and travelling in the same direction are combined. What is the amplitude of the resultant motion if their amplitudes are 3cm and 4cm and they differ in phase by π/2 radian?
View Solution
