Question

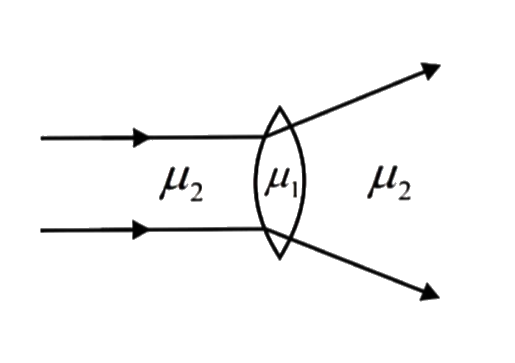
Two light waves having the same wavelength $\lambda$ in vacuum are in phase initially. Then the first ray travels a path of length $L_1$ through a medium of refractive index ${\mu }_1$. Then second ray travels a path of length $L_2$ throug a medium of refractive index ${\mu }_2$. The two waves are then combined to observed interference effects. The phase difference between the two, when they interfere, is

Options

A. `(2pi)/(lambda) (n_2L_1 - n_1 L_2)`
B. `(2pi)/(lambda) (n_1 L_1 - n_2 L_2)`
C. `(2pi)/(lambda) ((L_2)/(n_1) - (L_1)/(n_2))`
D. `(2pi)/(lambda) ((L_(1))/(n_(1)) - (L_(2))/(n_(2)))`

Explanation

To find the phase difference between two light waves that have traveled through different media, we can follow these steps:

1. Identify the Given Parameters:
- Wavelength in vacuum: \( \lambda \)
- Path length of the first wave in medium 1: \( L_1 \)
- Refractive index of medium 1: \( \mu_1 \)
- Path length of the second wave in medium 2: \( L_2 \)
- Refractive index of medium 2: \( \mu_2 \)

2. Calculate the Optical Path Lengths:
- The optical path length for the first wave traveling through medium 1 is given by:
\( \text{Optical Path Length}_1 = \mu_1 \cdot L_1 \)
- The optical path length for the second wave traveling through medium 2 is given by:
\( \text{Optical Path Length}_2 = \mu_2 \cdot L_2 \)

3. Determine the Path Difference:
- The path difference (\( \Delta x \)) between the two waves when they are combined is:
\( \Delta x = \text{Optical Path Length}_1 - \text{Optical Path Length}_2 \)
- Substituting the expressions for the optical path lengths:
\( \Delta x = (\mu_1 \cdot L_1) - (\mu_2 \cdot L_2) \)

4. Relate Path Difference to Phase Difference:
- The phase difference (\( \Delta \phi \)) between the two waves is related to the path difference by the formula:
\( \Delta \phi = \frac{2\pi}{\lambda} \cdot \Delta x \)
- Substituting the expression for \( \Delta x \):
\( \Delta \phi = \frac{2\pi}{\lambda} \cdot \left( \mu_1 \cdot L_1 - \mu_2 \cdot L_2 \right) \)

5. Final Expression for Phase Difference:
- Thus, the phase difference between the two waves when they interfere is:
\( \Delta \phi = \frac{2\pi}{\lambda} \left( \mu_1 L_1 - \mu_2 L_2 \right) \)