Two small discs of masses m1 and m2 are connected by a weightless spring resting on a smooth horizontal plance. The discs are set in motion with initial velocities v1andv2 whose directions are mutually perpendicular and in the same horizontal plane. Find the total energy E of hte system with reference to the frame fixed to the centre of mass.
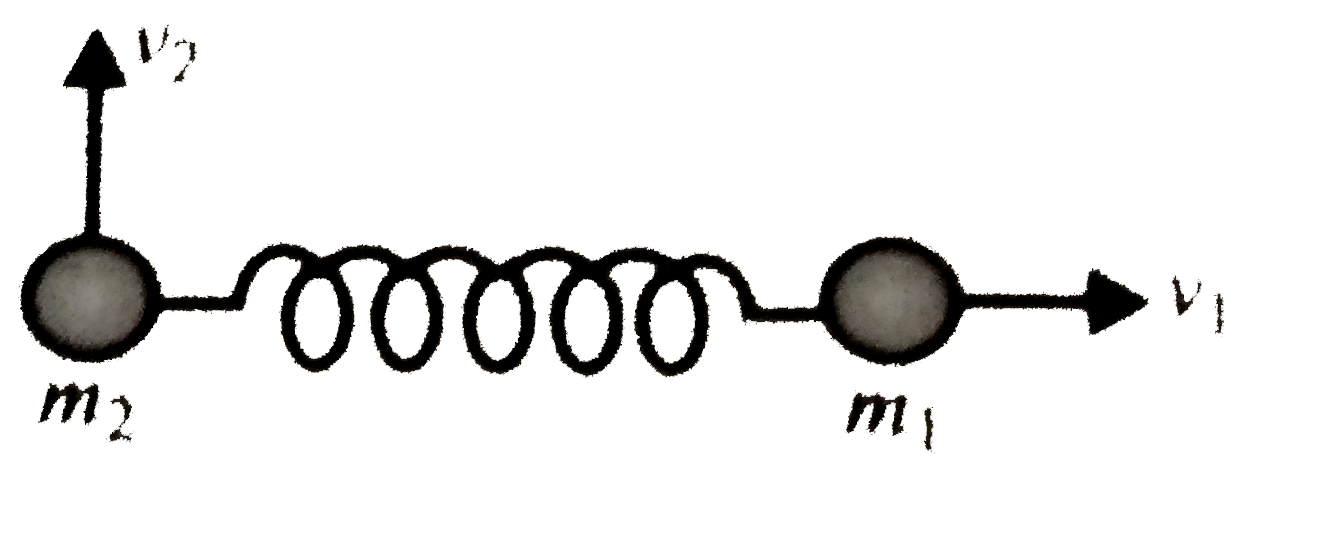
Let →vcm be the velocity of the centre of mass. Velcoity of m1 relative to thecentre of mas is (→v1−→vcm) and that of m2 relative to the centre of mass is (→v2−→v1)
Velocity of centre of mass →vcm=m1→v1m2→v2m1+m2
(→v1−→vcm)=m2m1+m2(→v1−→v2)
Hence |→v1−→vcm|=m2m1+m2(v21+v22)1/2
and →v2−→vcm=m1m1+m2(→v2−→v1)
Hence |→v2−→vcm|=m1m1+m2(v21+v22)1/2
total energy with respect to the centre of mass,
E=12m1|→v1−→vcm|212m1|→v2−→vcm|2
=12(m1m2m1+m2)(v21+v22)
where μ=m1m2m1+m2 is called reduced mass.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Solved Examples
13 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.1
20 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
Two small discs of masses m1 and m2 interconnected by a weightless spring rest on a smooth horizontal plane. The discs are set in motion with initial velocities v1 and v2 whose directions are mutually perpendicular and lie in a horizontal plane. Find the total energy ˜E of this system in the frame of the centre of inertia.
View SolutionA system consists of two small spheres of masses m1 and m2 interconnected by a weightless spring. At the moment t=0 the spheres are set in motion with the initial velocities v1 and v2 after which the system starts moving in the Earth's uniform gravitational field. Neglecting the air drag, find the time dependence of the total momentum of this system in the process of motion and of the radius vector of its centre of inertia relative to the initial position of the centre.
View Solution
Knowledge Check
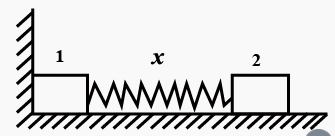
Two bars of masses m1 and m2 connected by a weightless spring of stiffness k, rest on a smooth horizontal plane. Bar 2 is shifted by a small distance x0 to the left and released. The veloicyt of the centre of mass of the system when bar 1 breaks off the wall is
 A(a) x0√km2m1+m2B(b) x0m1+m2√km2C(c) x0km1+m2m2D(d) x0√km1(m1+m2)
A(a) x0√km2m1+m2B(b) x0m1+m2√km2C(c) x0km1+m2m2D(d) x0√km1(m1+m2)Two bodies of masses m1 and m2 are moving with velocity v1 and v2 respectively in the same direction. The total momentum of the system in the frame of reference attached to the centre of mass is (v is relative velocity between the masses)
Am1m2vm1−m2B2m1m2vm1+m2CzeroD4m1m2vm1+m2Two blocks of masses m1=1kg and m2 = 2kg are connected by a spring of spring constant k = 24 N/m and placed on a frictionless horizontal surface. The block m1 is imparted an initial velocity v0 = 12cm/s to the right. The amplitude of osciallation is
A2 cmB1 cmC3 cmD4 cm
Two blocks of masses m1 and m2, connected by a weightless spring of stiffness k rest on a smooth horizontal plane as shown in Fig. Block 2 is shifted a small distance x to the left and then released. Find the velocity of centre of mass of the system after block 1 breaks off the wall.

View SolutionTwo bars of masses m1 and m2 connected by a weightless spring of stiffness ϰ (figure) rest on a smooth horizontal plane.

Bar 2 is shifted a small distance x to the left and then released. Find the velocity of the centre of inertia of the system after bar 1 breaks off the wall.
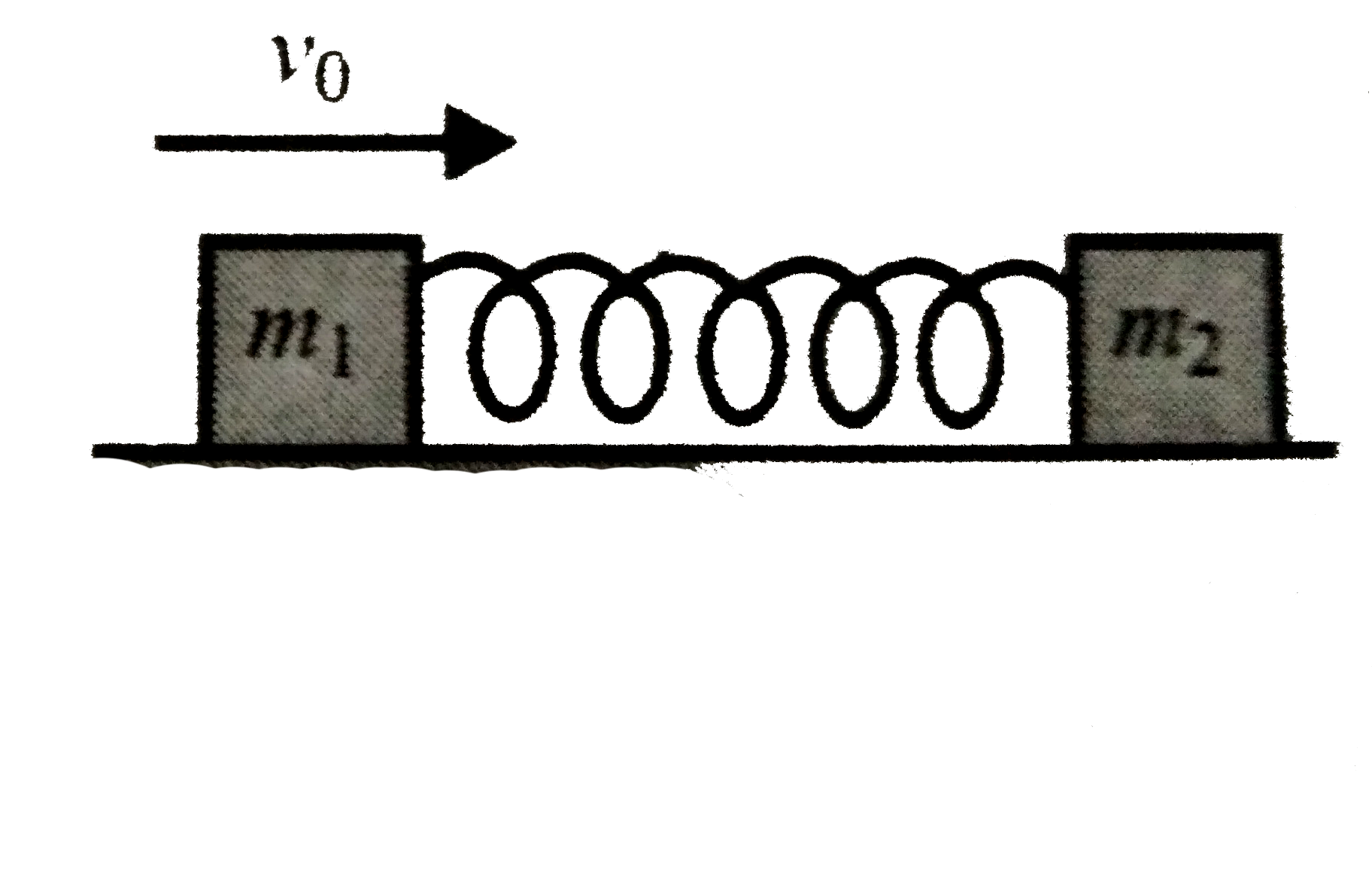
View SolutionTwo smooth blocks of mases m1andm2 attached with an ideal spring of stiffness k and kept on hrozontal surface. If m1 is projected with a horizontal velocity v0. Find the maximum compression of the spring.
View SolutionA closed system consists of two particles of masses m1 and m2 which move at right angles to each other with velocities v1 and v2. Find:
(a) the momentum of each particle and
(b) the total kinetic energy of the two particles in the reference frame fixed to their centre of inertia.
View SolutionTwo masses, m1 and m2 , are moving with velocities v1 and v2. Find their total kinetic energy in the reference frame of centre of mass.
View Solution
