Two smooth spheres. $A$ and $B$ . having equal radii, lie on a horizontal table. $A$ is of mass $m$ and $B$ is of mass $3 m$ . The spheres are projected towards each other with velocity vector $5 ˆ i + 2 ˆ j$ and $2 ˆ i - ˆ j$ , respectively, and when they collide the lige joining their centres is parallel to the vector $ˆ i$ . If the coefficient of restitution between $A$ and $B$ is $1 / 3$ , find the velocities after impact and the loss in kinetic energy caused by the collision. Find also the magnitude of the impulses that act at the instant of impact.

Text Solution

Verified by Experts

The velocity component of $A$ and $B$ perpendicular to the line connecting centres are unchaned by the impact. It is because no force acts on $A$ and $B$ in a direction perpendicular to the line connecting the centre

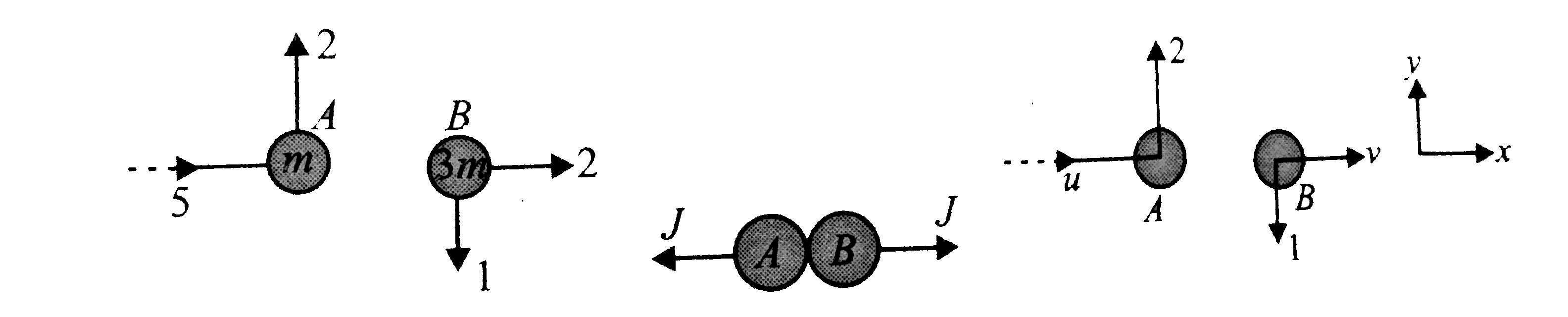
Applying conservation of inear momentum nd the law of restitution we have in $x$ direction
$5 m + (3 m) (2) = mu + 3 m v$ ............i
$and \frac{1}{3} (5 - 2) = v - u$ .........ii
Solving these equations, we have $u = 2$ and $v = 3$
The velocities of $A$ and $B$ after the impact are $2 ˆ i + 2 ˆ j$ and $3 ˆ i - ˆ j$ respectively.
Before the kinetic energy of $A$ is
$\frac{1}{2} m (5^{2} + 2^{2}) = 29 m / 2$
and of $B$ is
$\frac{1}{2} (3 m) (2^{2} + 1^{2}) = 15 m / 2$
After the impact the kinetic energy of $A$ is
$\frac{1}{2} m (2^{2} + 2^{2}) = 4 m$
and that of $B$ is
$\frac{1}{2} (3 m) (3^{2} + 1^{2}) = 15 m$
Therefore, the loss in $K E$ at impact is
$\frac{29}{2} m + \frac{15}{2} m - 4 m - 15 m = 3 m$
To find the value of $J$ we consider the change in momemtum along $ˆ i$ for one sphere only.
For sphere $B, J = 3 m (3 - 2)$ or $J = 3 m,$

Updated on:21/07/2023