Two thin convex lenses of focal lengths f1 and f2 are separated by a horizontal distance d(d<f1 and d<f2) and their centers are displaced by a vertical separation as shown in the figure. A parallel beam of rays coming from left. Take the origin of coordinates O at the center of first lens.
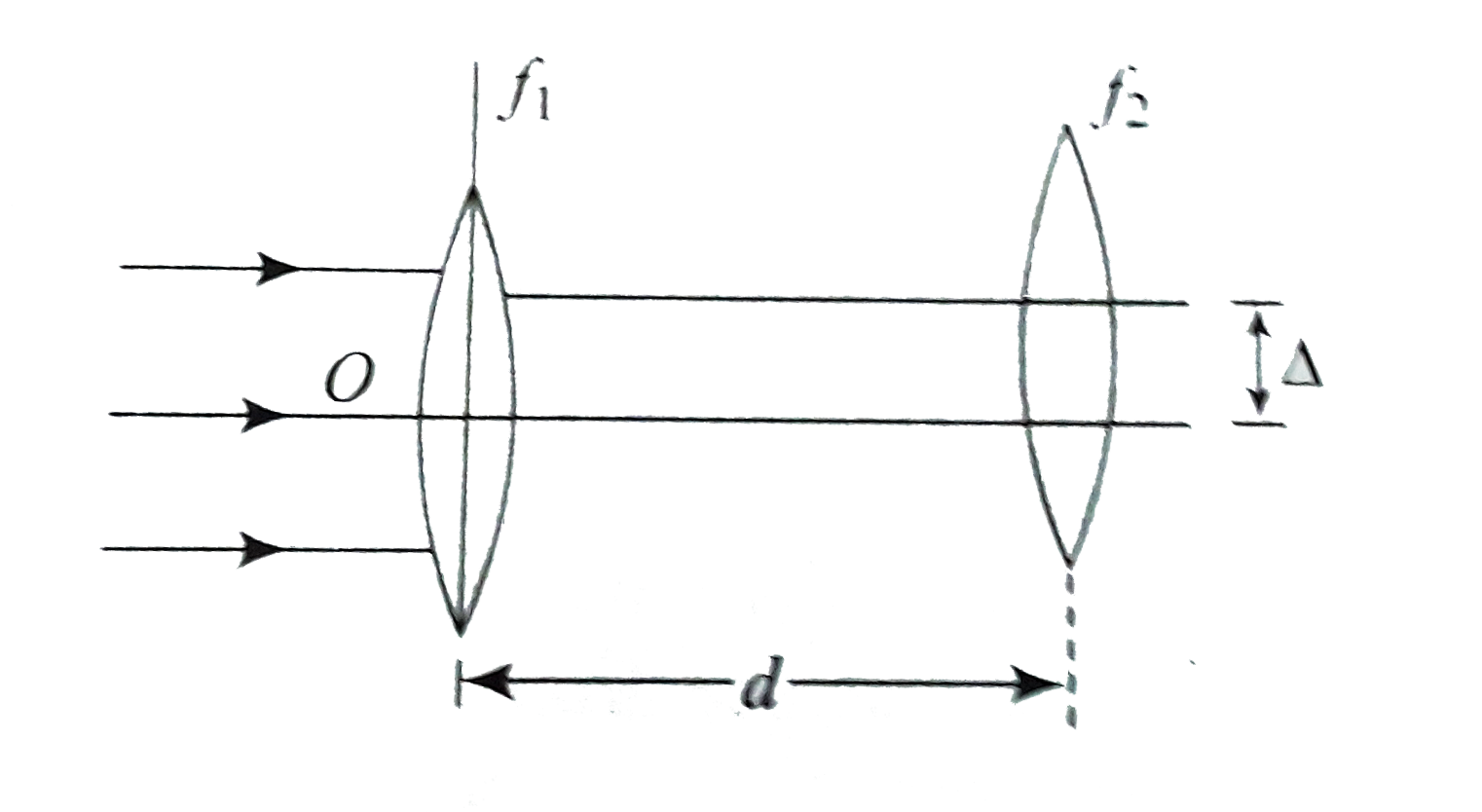
Q. Find the y-coordinate of the focal point of this lens system.
x=f1f2f1+f2,y=Δ
x=f1(f2+d)f1+f2−d,y=Δf1+f2
x=f1f2+d(f1−d)f1+f2−d,y=Δ(f1−d)f1+f2−d
x=f1f2+d(f1−d)f1+f2−d,y=Δ(f1−d)f1+f2−d
The correct Answer is:c.
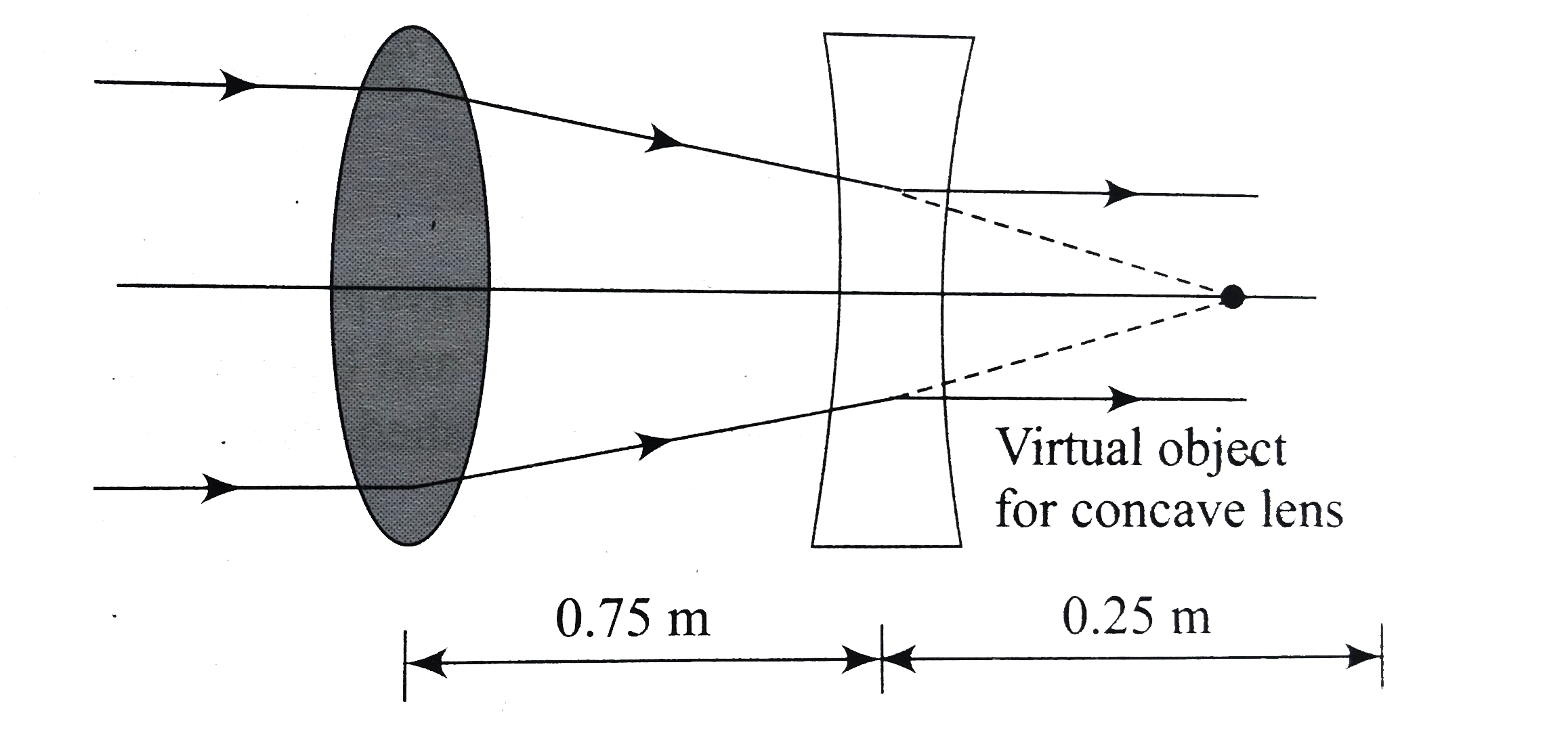
The image I′ of parallel rays formed by lens 1 will act as a virtual object.
Applying lens formula for lens 2,
1v−1u=1f
⇒1v−1f1−d=1f2
⇒v=f2(f1−d)f2+f1−d
The horizontal distance of the image O is
x=d+f2(f1−d)f2+f1−d
=df2+df1−d2+f2f1−df2f2+f1−d
=f1f2+d(f1−d)f2+f1−d
To find teh y-coordinate, we use magnification formula for lens 2,
m=vu=f2(f1−d)f1+f2−df1−d=f2f1+f2−d. Also
m=h2Δ⇒h2=Δ×f2f1+f2−d
Therefore, the y-coordinate,
y=Δ−h2
=Δ−Δf2f1+f2−d
=Δf1+Δf2−Δd−Δf2f1+f2−d=Δ(f1−d)f1−f2−d
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Multiple Correct Answers Type
8 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig Type
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - True/False
5 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
Two thin convex lenses of focal lengths f1 and f2 are separated by a horizontal distance d (whered<f1,d<f2) and their centres are displaced by a vertical separation △ as shown in the fig.
Taking the origin of coordinates O, at the centre of the first lens the x and y coordinates of the focal point of this lens system, for a parallel beam of rays coming form the left, are given by:
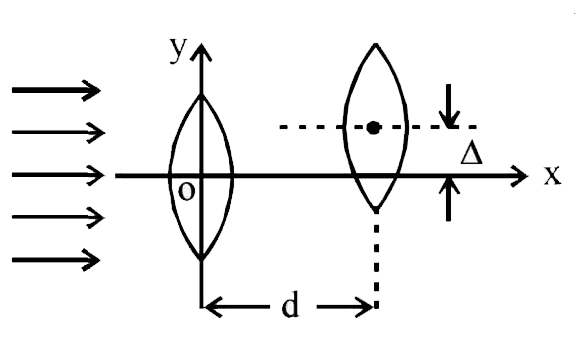
View SolutionTwo thin convex lenses of focal lengths f1 and f2 are separated by a horizontal distance d (whered<f1,d<f2) and their centres are displaced by a vertical separation △ as shown in the fig.
Taking the origin of coordinates O, at the centre of the first lens the x and y coordinates of the focal point of this lens system, for a parallel beam of rays coming form the left, are given by:
View SolutionTwo thin lenses of focal lengths f1 and f2 are in contact. The focal length of this combination is
View SolutionTwo thin lenses of focal length f1 and f2 are in contact and coaxial. The power of the combination is
View SolutionTwo converging lenses of focal lengths f1=10cm and f2=20cm are placed at some separation. A parallel beam of light is incident on first lens. Then,
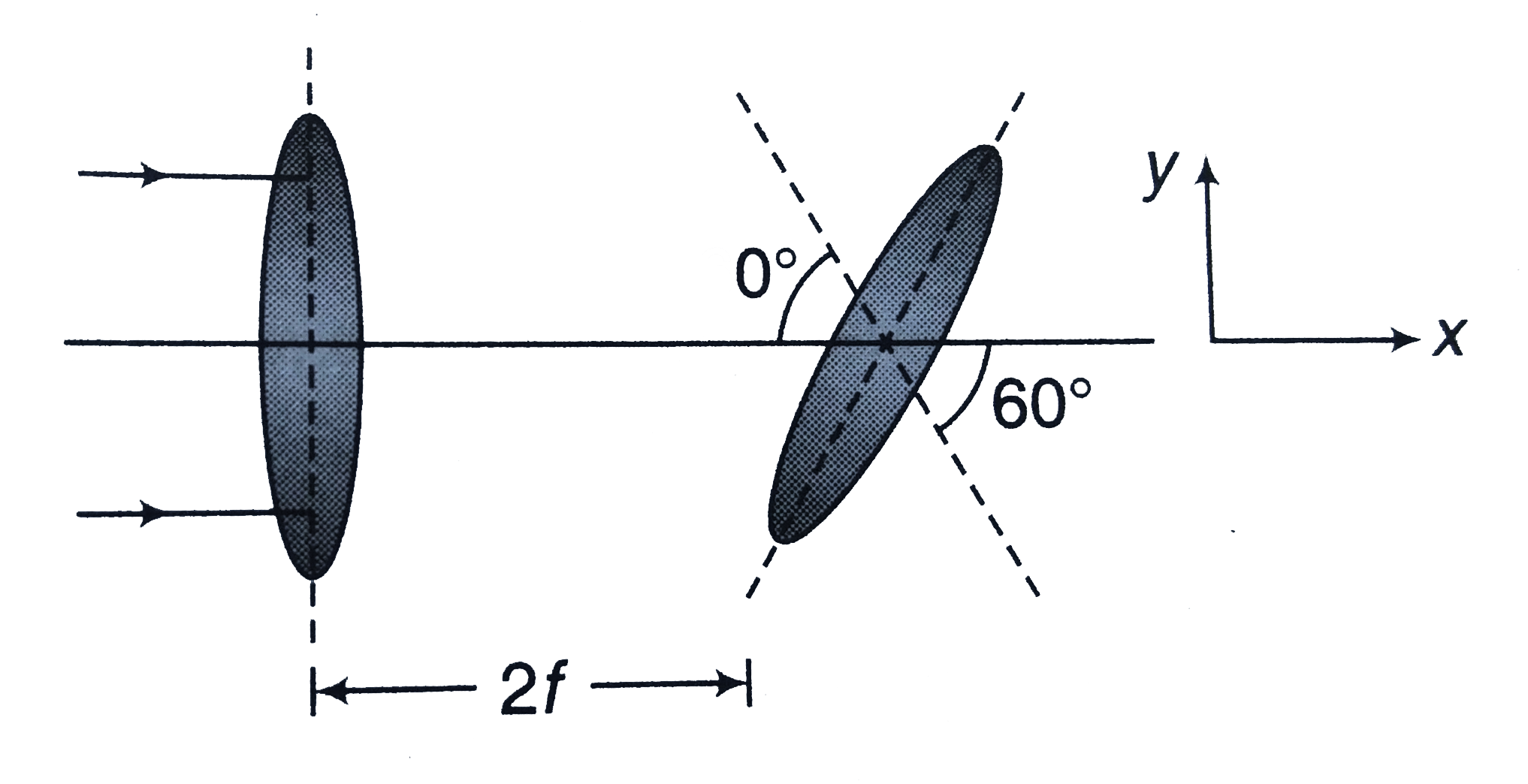
View SolutionTwo converging lenses of the same focal length f are separated by a distance 2f. The axis of the second lens is inclined at angle θ=60∘ with respect to the axis of the first lens. A parallel paraxial beam of light is incident from left side of the lens. Find the coordinates of the final image with respect to the origin of the first lens.
View SolutionA convex lens of focal length f1 is kept in contact with a concave lens of focal length f2. Find the focal length of the combination.
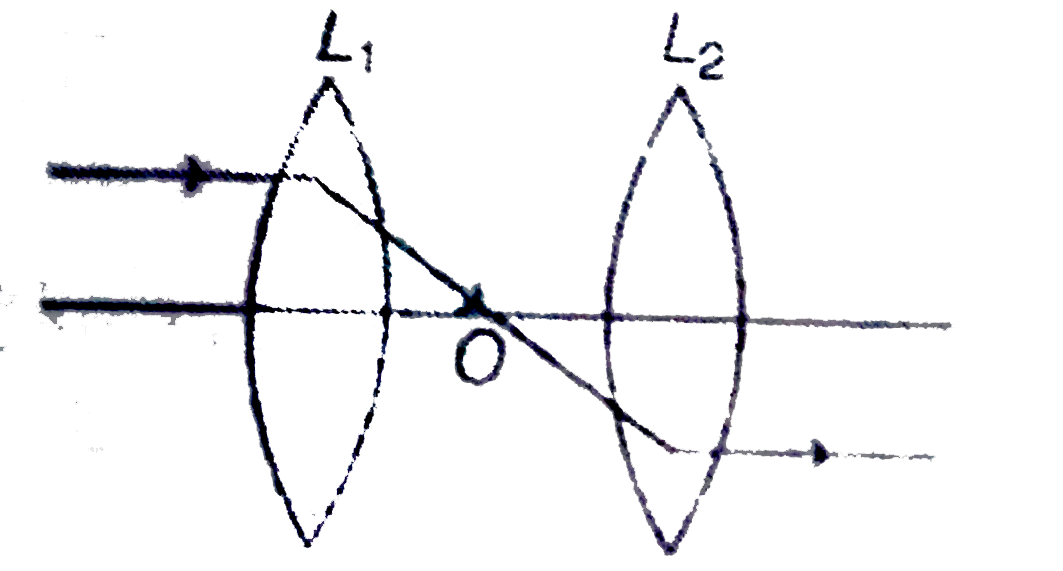
View SolutionIn the figure shown, there are two convex lenses L1 and L2 having focal. Lengths f1 and f2 respectively. The distance between L1 and L2 will be
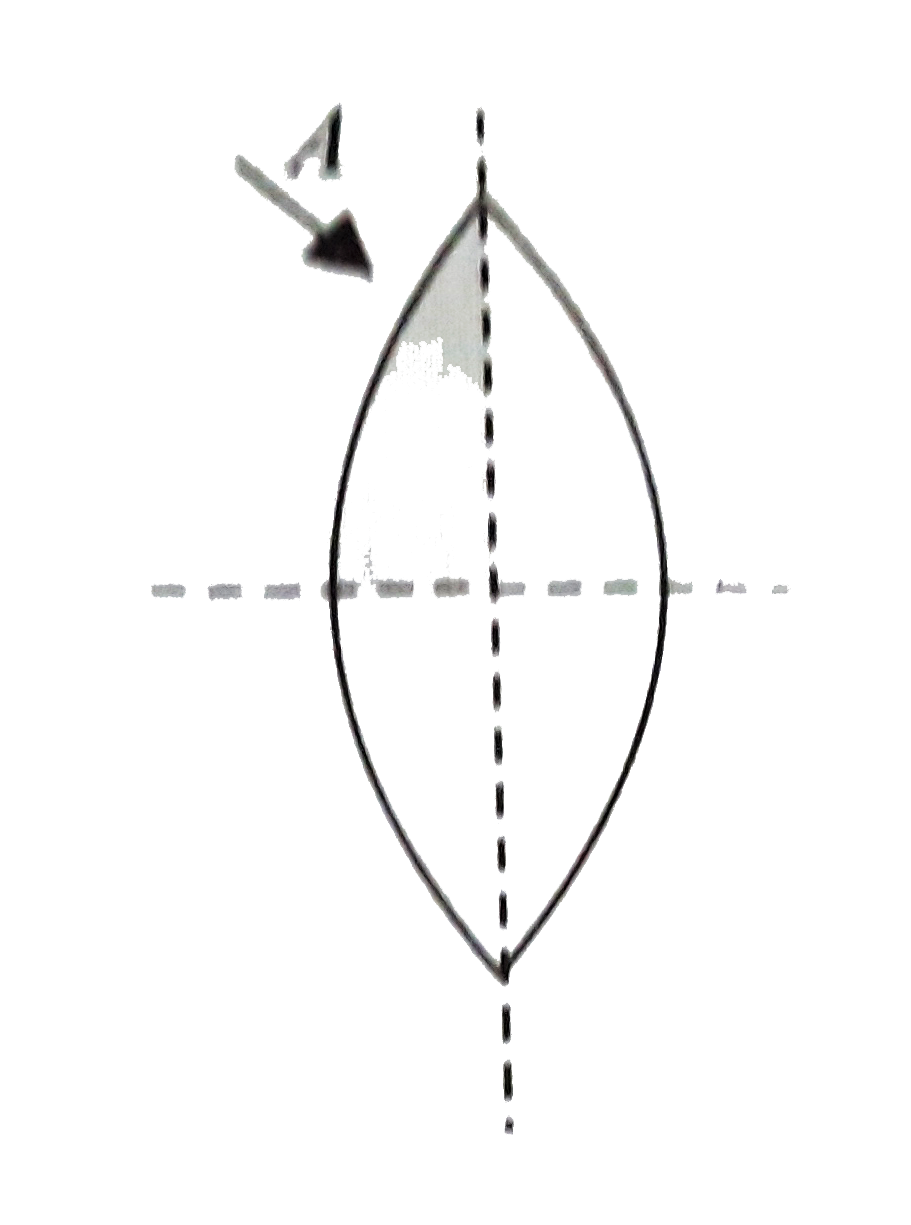
View SolutionA convex lens of focal length f cut into parts first horizontally and then vertically. Find the focal length of part A of the lens, as shown:
View SolutionA convex lens of focal length 10 cm and a concave lens of same focal length in value are placed co-axially at a separation of 20 cm as shown in figure. A point object is at infinity on the principal axis. Take the optic center of convex lens as origin of co ordinate system and the principal axis as x-axis as shown in figure.

The co-ordinates of the image formed after refraction through both the lenses is
View Solution
