Two towers AB and CD are situated a distance d apart as shown in Fig. 5.45. AB is 20m high and
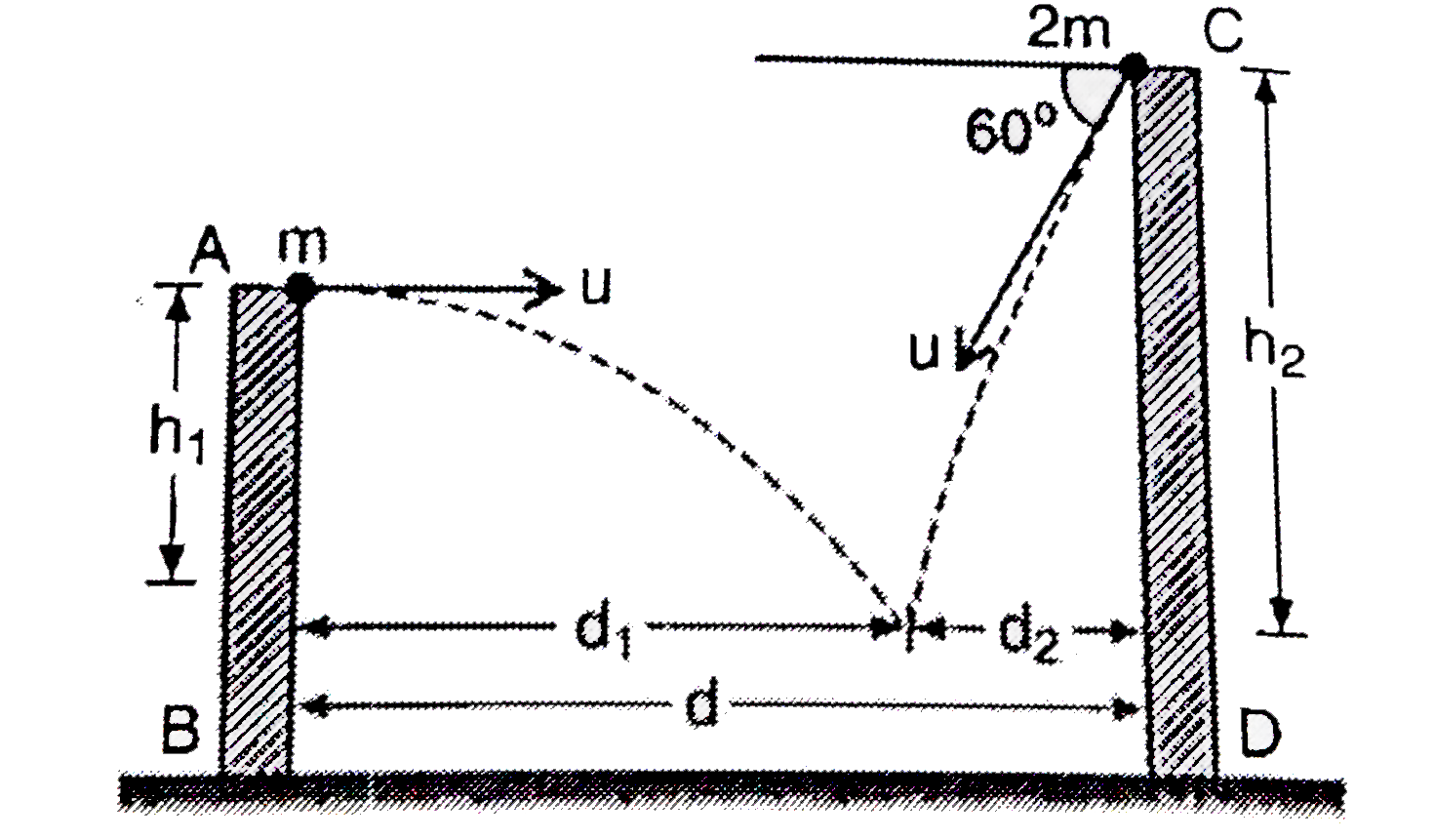
CD is 30m highfrom the ground . An object of mass m is thrown from the top of AB horizontally with a velocity 10m/s towards CD. simu ltaneously another object of mass 2 m is thrown from the top of CD at an angle of 60∘ to the horizotnal towards AB with the same magnitude of initial velocity as that of the first object. the two objects move in the same vertical plane, clollide in mid air and stick to each other, (a) Calcu late the distance d between the towers and (b) fin the position where the objects hit the ground.
Let the two particles collide after time r, then from 2nd equation of motion, i.e.,
s=ut+12at2
for vertical motion of A,
h1=12gt2 ........(i) ltbr> While for B,
h2=(usin60∘)t+12gt∘ ..........(ii)
But as the collision will take place at same level,
30−h2=20−h1,,i.e.,h2−h1=10
Which, in the light of Eqns. (i) and (ii), gives
10×(√32)t=10,i.e.,t=2√3s
(a) Now as horizontal motion is independent of vertical motion, so from 2nd Eqn. of motion. i.e. s=ut+(1/2)at2 distance travelled by m towards 2m horizotnall.
dt=10×2√3=20√3m
and distance travelled by 2m towards m horizontally,
d2=ucosθ×t
=10×12×2√3=10√3m.
So, distance between the towers AB and CD,
d=d1+d∘=20√3=30√3=10√3=17m
(b) Applying conservation of linear momentum along horizontal direction
μ−2μcos60∘=3mV, i.e., V=0
i.e., the velocity of combined mass fter collision along horizontal is zero, so after collision it will move vertically down from the point of collision and will hit the ground at a distance d1=20/√3 from AB or at a distance d2=10/√3 from CD between BD.
Topper's Solved these Questions
Similar Questions
Two towers AB and CD are situated at a distance d apart, as shown in figure. AB is 20 m high and CD is 30 m high from the ground. An object of mass m is thrown from the top of AB horizontally with a velocity of 10 m/s towards CD. Simultaneously another object of mass 2 m is thrown fromt the top of CD at an angle of 60∘ to the horizontal towards AB with the same magnitude of initial velocity as that oft he first object. The two objects move in the same vertical plane, collide in mid air and stick to each other (i) calculate the distance between the towers and (ii) find the position where the objects hit the ground.

View SolutionTwo towers AB and CD are situated at a distance d apart, as shown in figure. AB is 20 m high and CD is 30 m high from the ground. An object of mass m is thrown from the top of AB horizontally with a velocity of 10 m/s towards CD. Simultaneously another object of mass 2 m is thrown fromt the top of CD at an angle of 60∘ to the horizontal towards AB with the same magnitude of initial velocity as that oft he first object. The two objects move in the same vertical plane, collide in mid air and stick to each other (i) calculate the distance between the towers and (ii) find the position where the objects hit the ground.

View Solution
Knowledge Check
If a body A of mass m is thrown with velocity v at an angle of 30∘ to the horizontal and another body B of mass 2m is thrown with the same speed at an angle of 60∘ to the horizontal. Then
ARARB=11B(HA)max(HB)max=13CTATB=1√3D(1), (2), (3)A stone is thrown from the top of a tower at an angle of 30∘ above the horizontal level with a velocity of 40 m/s. it strikes the ground after 5 second from the time of projection then the height of the tower is
A20 mB25 mC40 mD55mA ball of mass m is thrown at an angle of 45∘ to the horizontal,, from the top of a 65m high tower AB as shown in Fig. at t=0. Another identical ball is thrown with velocity 20m/s horizontally towards AB from the top of a 30m high tower CD1s after the projection of the first ball. Both the balls move the same vertical plane. If they collide in mid-air,

the distance AC isA20mB30mC40mD20m
Two towers AB and CD are situated a distance d apart as: shown in Fig. 1E.139 (a). AB is 20 m high and CD is 30 m high from the ground. An object of mass mis thrown from the top of AB horizontally with a velocity of 10 m/s towards CD.
Simultaneously another object of mass 2m is thrown from the ·top of CD at an angle of 60∘ to the horizontal towards AB with the same magnitude of initial velocity as that of the_ first' objects . The two objects move in the same vertical plane, collide in mid-air and stick to each other.
Calculate the distance d between the towers.
View SolutionA ball is thrown from the top of a building with a velocity of 10 m/s and returns with a velocity of 30 m/s to the ground. Find the height of the building.
View SolutionA ball of mass 2kg is thrown with velocity 10ms at an angle of 30°to the horizontal and another ball with the same mass is thrown at an angel of 60° to the horizontal with the same speed.The ratio will be their range will be
View SolutionA particle of mass m is thrown horizontally from the top of a tower and anoher particle of mass 2m is thrown vertically upward. The acceleration of centre of mass is
View SolutionA ball of mass m is thrown at an angle of 45∘ to the horizontal,, from the top of a 65m high tower AB as shown in Fig. at t=0. Another identical ball is thrown with velocity 20m/s horizontally towards AB from the top of a 30m high tower CD1s after the projection of the first ball. Both the balls move the same vertical plane. If they collide in mid-air,

the two balls will collide at time t=
View Solution
