Two trains A and B are approaching each other on a straight track, the former with a uniform velocity of 25 m/s and other with 15m/s, when they are 225 m a part brakes are simultaneously applied to both of them. The deceleration given by the brakes to thetrain B increases linearly with time by 0.3m/s2 every second, while the train A is given a uniform deceleration, (a) What must be the minimum deceleration of the train A so that the trains do not collide ? (b) What is the time taken by the trains to come to stop ?
5m/s2
2.5m/s2
1.5m/s2
7.5m/s2
The correct Answer is:B
To solve the problem, we need to determine the minimum deceleration of train A so that the two trains do not collide, and then find the time taken by both trains to come to a stop.
Step 1: Understand the initial conditions.
- Train A has an initial velocity uA=25m/s.
- Train B has an initial velocity uB=15m/s.
- The distance between the two trains when brakes are applied is d=225m.
Step 2: Determine the deceleration of train B.
- The deceleration of train B increases linearly with time at a rate of 0.3m/s2.
- Therefore, the deceleration at time t is given by:
aB=−0.3tm/s2
Step 3: Calculate the time taken for train B to stop.
- The final velocity of train B when it stops is vB=0.
- Using the equation of motion:
vB=uB+aB⋅t
Substituting the values:
0=15−0.3t2
Rearranging gives:
0.3t2=15⟹t2=150.3=50⟹t=√50≈7.07s
Step 4: Calculate the distance traveled by train B during this time.
- Using the equation of motion:
sB=uB⋅t+12aB⋅t2
Substituting the values:
sB=15⋅7.07+12(−0.3)⋅(7.07)2
sB=106.05−0.15⋅50≈106.05−7.5=98.55m
Step 5: Calculate the distance remaining for train A to cover.
- The distance covered by train B is approximately 98.55m.
- Therefore, the distance remaining between the two trains when train B stops is:
d′=225−98.55≈126.45m
Step 6: Determine the deceleration required for train A.
- Train A must cover the distance d′ in the same time t that train B takes to stop.
- Using the equation of motion for train A:
sA=uA⋅t+12aA⋅t2
We know sA=126.45m and t=7.07s:
126.45=25⋅7.07+12aA⋅(7.07)2
126.45=176.75+12aA⋅50
Rearranging gives:
12aA⋅50=126.45−176.75⟹12aA⋅50=−50.3
aA=−50.3⋅250=−2.012m/s2
Step 7: Calculate the time taken by train A to stop.
- Using the equation of motion:
vA=uA+aA⋅t
Setting vA=0:
0=25−2.012⋅t
t=252.012≈12.43s
Final Answers:
(a) The minimum deceleration of train A must be approximately 2.012m/s2 to avoid a collision.
(b) The time taken by train A to come to a stop is approximately 12.43s.
- Train A has an initial velocity uA=25m/s.
- Train B has an initial velocity uB=15m/s.
- The distance between the two trains when brakes are applied is d=225m.
- The deceleration of train B increases linearly with time at a rate of 0.3m/s2.
- Therefore, the deceleration at time t is given by:
aB=−0.3tm/s2
- The final velocity of train B when it stops is vB=0.
- Using the equation of motion:
vB=uB+aB⋅t
Substituting the values:
0=15−0.3t2
Rearranging gives:
0.3t2=15⟹t2=150.3=50⟹t=√50≈7.07s
- Using the equation of motion:
sB=uB⋅t+12aB⋅t2
Substituting the values:
sB=15⋅7.07+12(−0.3)⋅(7.07)2
sB=106.05−0.15⋅50≈106.05−7.5=98.55m
- The distance covered by train B is approximately 98.55m.
- Therefore, the distance remaining between the two trains when train B stops is:
d′=225−98.55≈126.45m
- Train A must cover the distance d′ in the same time t that train B takes to stop.
- Using the equation of motion for train A:
sA=uA⋅t+12aA⋅t2
We know sA=126.45m and t=7.07s:
126.45=25⋅7.07+12aA⋅(7.07)2
126.45=176.75+12aA⋅50
Rearranging gives:
12aA⋅50=126.45−176.75⟹12aA⋅50=−50.3
aA=−50.3⋅250=−2.012m/s2
- Using the equation of motion:
vA=uA+aA⋅t
Setting vA=0:
0=25−2.012⋅t
t=252.012≈12.43s
(a) The minimum deceleration of train A must be approximately 2.012m/s2 to avoid a collision.
Topper's Solved these Questions
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - MATCH THE COLUMN
10 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - MCQ_TYPE
27 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
Similar Questions
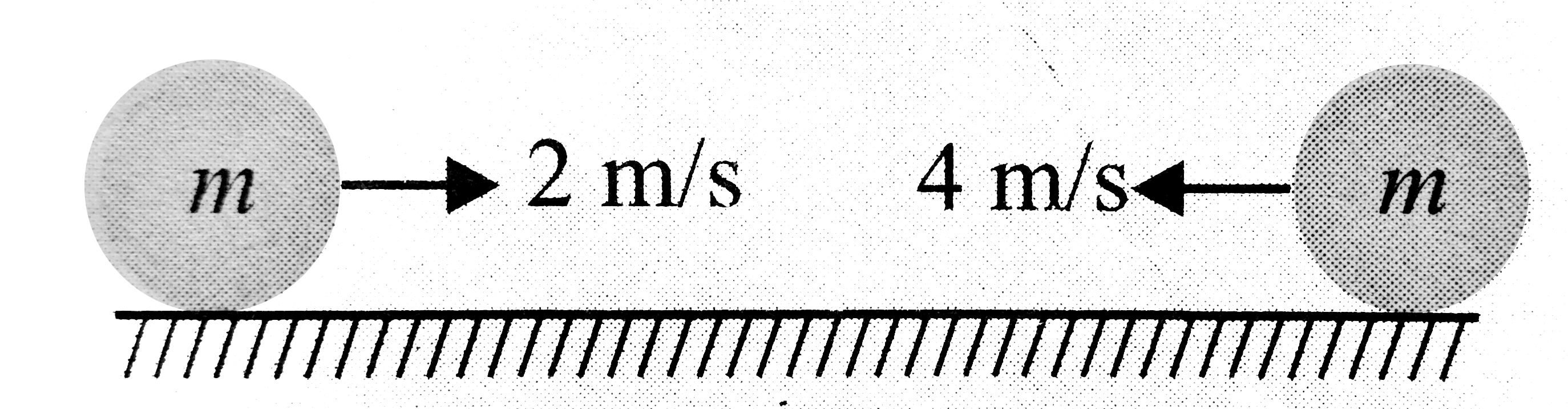
Two identical balls are approaching towards each other on a straight line with velocity 2m/s and 4m/s, respectiely. Find the final velocities after elastic collision between them.
View SolutionTwo trains one travelling at 54 kmph and the other at 72 kmph are headed towards one an other along a straight track. When they are 12 km apart, both drivers simultaneously see the other train and apply their brakes. If each train is decelerated at the rate of 1ms−2 , will there be collision ?
View Solution
Knowledge Check
Two trains, each 50 m long are travelling in opposite direction with velocity 10 m/s and 15 m/s. The time of crossing is
A2sB4sC2√3sD4√3 sTwo cars travelling towards each other on a straight road at velocity 10m/s and 12m/s respectively. When they are 150 metre apart, both drivers apply their brakes and each car decelerates at 2m/s2 until it stops. How far apart will they be when they have both come to a stop?
A89 mB98 mC108 mD150 m
Two waves are approaching each other with a velocity of 20m/s and frequency n. The distance between two consecutive nodes is
View SolutionAn open carriage in a goods train is moving with a uniform velocity of 10 m/s. If the rain adds water with zero velocity at the rate of 5 kg/s, then the additional force required by the engine to maintain the same velocity of the train is
View SolutionA 100 m long train is moving with a velocity of 10 m/s, then find out the time taken by train to cross a bridge of length 150 m.
View SolutionTrain A and B are approaching towards each other on a parallel track 99 m apart at t = 0 their position are shown. Train A whistles a short pulse of frequency of 596 Hz. Velocity of sound is 330 m/s.

View SolutionIf the velocity v of a particle moving along a straight line decreases linearly with its displacement from 20m/s to a value approaching zero at s=30m, determine the acceleration of the particle when s=15m.
View SolutionTwo trains approach each other along pentiel tancks each moving with a velocity 2 m/s. A whistle of frequency 250 Hz is sounded on ench train. Find the frequency of hers between the two suces as heard by the listener on the other train. Velocity of sound is 332 ms.
View Solution
