Two vectors have magnitudes 3 unit and 4 unit respectively. What should be the angel between them if the magnitude of the resultant is (a). 1 unit (b). 5 unit and (c). 7 unit.
The correct Answer is:A, B, C
To find the angle between two vectors with magnitudes 3 units and 4 units, given different resultant magnitudes (1 unit, 5 units, and 7 units), we can use the formula for the magnitude of the resultant vector:
R=√A2+B2+2ABcos(θ)
where:
- R is the magnitude of the resultant vector,
- A and B are the magnitudes of the two vectors,
- θ is the angle between the two vectors.
1. Substitute the values into the formula:
1=√32+42+2⋅3⋅4cos(θ)
2. Calculate 32+42:
32+42=9+16=25
3. Now, the equation becomes:
1=√25+24cos(θ)
4. Square both sides:
12=25+24cos(θ)
1=25+24cos(θ)
5. Rearranging gives:
24cos(θ)=1−25
24cos(θ)=−24
6. Therefore:
cos(θ)=−1
7. This implies:
θ=180∘
(b) For R=5 units:
1. Substitute the values into the formula:
5=√32+42+2⋅3⋅4cos(θ)
2. Using the previous calculation:
5=√25+24cos(θ)
3. Square both sides:
52=25+24cos(θ)
25=25+24cos(θ)
4. Rearranging gives:
24cos(θ)=25−25
24cos(θ)=0
5. Therefore:
cos(θ)=0
6. This implies:
θ=90∘
(c) For R=7 units:
1. Substitute the values into the formula:
7=√32+42+2⋅3⋅4cos(θ)
2. Using the previous calculation:
7=√25+24cos(θ)
3. Square both sides:
72=25+24cos(θ)
49=25+24cos(θ)
4. Rearranging gives:
24cos(θ)=49−25
24cos(θ)=24
5. Therefore:
cos(θ)=1
6. This implies:
θ=0∘
Summary of Results:
- For R=1 unit, θ=180∘
- For R=5 units, θ=90∘
- For R=7 units, θ=0∘
- R is the magnitude of the resultant vector,
- A and B are the magnitudes of the two vectors,
- θ is the angle between the two vectors.
1=√32+42+2⋅3⋅4cos(θ)
2. Calculate 32+42:
32+42=9+16=25
3. Now, the equation becomes:
1=√25+24cos(θ)
4. Square both sides:
12=25+24cos(θ)
1=25+24cos(θ)
5. Rearranging gives:
24cos(θ)=1−25
24cos(θ)=−24
6. Therefore:
cos(θ)=−1
7. This implies:
θ=180∘
5=√32+42+2⋅3⋅4cos(θ)
2. Using the previous calculation:
5=√25+24cos(θ)
3. Square both sides:
52=25+24cos(θ)
25=25+24cos(θ)
4. Rearranging gives:
24cos(θ)=25−25
24cos(θ)=0
5. Therefore:
cos(θ)=0
6. This implies:
θ=90∘
7=√32+42+2⋅3⋅4cos(θ)
2. Using the previous calculation:
7=√25+24cos(θ)
3. Square both sides:
72=25+24cos(θ)
49=25+24cos(θ)
4. Rearranging gives:
24cos(θ)=49−25
24cos(θ)=24
5. Therefore:
cos(θ)=1
6. This implies:
θ=0∘
- For R=1 unit, θ=180∘
- For R=5 units, θ=90∘
- For R=7 units, θ=0∘
Topper's Solved these Questions
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Example
40 Videos
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - check point 2.1
15 Videos
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Single Correct
39 Videos
- UNITS, DIMENSIONS & ERROR ANALYSIS BOOK - DC PANDEY ENGLISHCHAPTER - UNITS, DIMENSIONS & ERROR ANALYSIS EXERCISE - Medical entrances gallery
32 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
Similar Questions
Two vectors have magnitudes 3 unit and 4 unit respectively. What should be the angel between them if the magnitude of the resultant ils a. 1 unit, b. 5 unit and c. 7 unit.
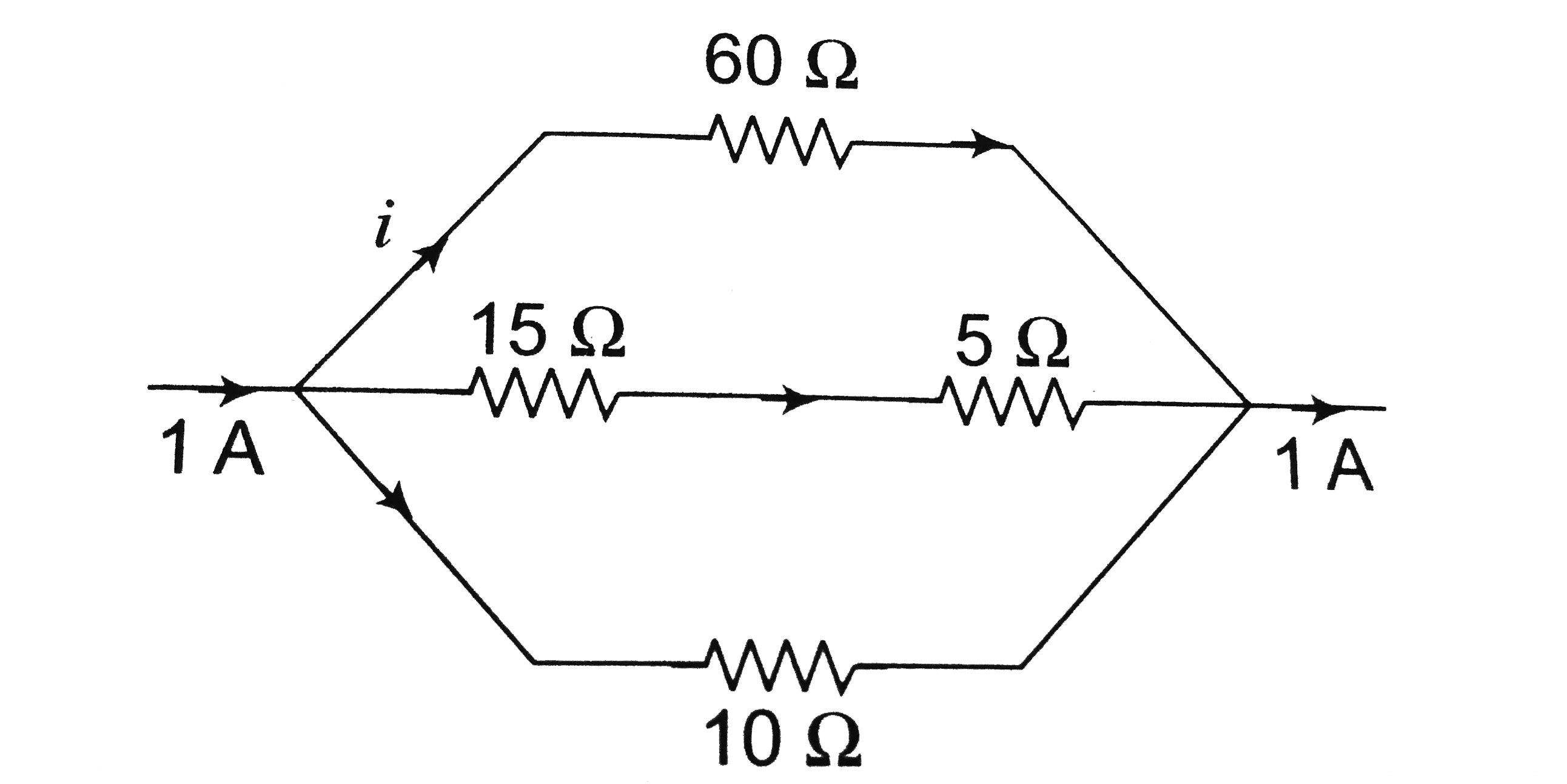
View SolutionThe magnitude in i in ampere unit is
View SolutionTwo vectors have magnitudes 6 and 8 units, respectively. Find the magnitude of the resultant vector if the angle between vectors is (a) 60∘ (b) 90∘ and ( c ) 120∘.
View SolutionTwo vectors A and B have magnitudes 2 units and 4 units respectively. Find A. B is angle between these two vectors is (a) 0∘ (b) 60∘ (c) 90∘ (d) 120∘ .
View SolutionIf A=B+C have scalar magnitudes of 5,4,3 units respectively , then find the angle between A and C.
View SolutionTwo vectors A and B have magnitudes 6 units and 8 units respectively. Find |A-B|, if the angle between two vectors is .(a) 0∘ (b) 180∘ (c) 180∘ (d) 120∘ .
View SolutionThe maximum and minimum magnitudes of the resultant of two vectors are 23 units and 7 units respectively. If these two vectors are acting at right angles to each other, the magnitude of their resultant will be
View SolutionThe maximum and minimum magnitudes of the resultant of two vectors are 23 units and 7 units respectively. If these two vectors are acting at right angles to each other, the magnitude of their resultant will be
View SolutionTwo vectors having equal magnitude of 5 units, have an angle of 60∘ between them. Find the magnitude of their resultant vector and its angle from one of the vectors.

View SolutionTwo vectors of equal magnitudes have a resultant equle to either of them, than the angel between them will be
View Solution
