Uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force is applied at the top point P of the cylinder so that it start pure rolling .In the above question, the frictional force on the cylinder is
towards right
towards left
towards right
towards left
To solve the problem of finding the frictional force acting on a uniform solid cylinder of mass m and radius R when a horizontal constant force F is applied at the top point P of the cylinder, we can follow these steps:
Step 1: Understand the System
We have a uniform solid cylinder placed on a rough horizontal surface. A horizontal force F is applied at the top of the cylinder, and we need to find the frictional force acting on it when it starts pure rolling.
Step 2: Identify Forces Acting on the Cylinder
1. The applied force F acts horizontally at the top point P.
2. The frictional force f acts at the point of contact with the ground, opposing the motion.
3. The weight of the cylinder mg acts downward at its center of mass.
4. The normal force N acts upward at the point of contact.
Step 3: Write the Equation of Motion
Using Newton's second law for translational motion:
F−f=ma(1)
where a is the linear acceleration of the center of mass of the cylinder.
Step 4: Write the Torque Equation
The torque τ about the center of mass due to the applied force F and the frictional force f is given by:
τ=F⋅R−f⋅R=Iα(2)
where I is the moment of inertia of the cylinder and α is the angular acceleration.
For a solid cylinder, the moment of inertia I is:
I=12mR2
Step 5: Relate Linear and Angular Acceleration
For pure rolling motion, the relationship between linear acceleration a and angular acceleration α is:
a=αR(3)
Step 6: Substitute α in the Torque Equation
From equation (2), substituting α using equation (3):
F⋅R−f⋅R=12mR2⋅aR
Simplifying gives:
F−f=12ma(4)
Step 7: Solve the System of Equations
Now we have two equations:
1. F−f=ma (equation 1)
2. F−f=12ma (equation 4)
Setting these equal to each other:
ma=12ma
Subtracting gives:
12ma=f
Thus, we can express f:
f=12ma
Step 8: Substitute a from Equation (1)
From equation (1):
f=F−ma
Substituting a from f=12ma:
f=F−2f
Rearranging gives:
3f=F⇒f=F3
Final Answer
The frictional force f acting on the cylinder is:
f=F3
We have a uniform solid cylinder placed on a rough horizontal surface. A horizontal force F is applied at the top of the cylinder, and we need to find the frictional force acting on it when it starts pure rolling.
1. The applied force F acts horizontally at the top point P.
2. The frictional force f acts at the point of contact with the ground, opposing the motion.
3. The weight of the cylinder mg acts downward at its center of mass.
4. The normal force N acts upward at the point of contact.
Using Newton's second law for translational motion:
F−f=ma(1)
where a is the linear acceleration of the center of mass of the cylinder.
The torque τ about the center of mass due to the applied force F and the frictional force f is given by:
τ=F⋅R−f⋅R=Iα(2)
where I is the moment of inertia of the cylinder and α is the angular acceleration.
I=12mR2
For pure rolling motion, the relationship between linear acceleration a and angular acceleration α is:
a=αR(3)
From equation (2), substituting α using equation (3):
F⋅R−f⋅R=12mR2⋅aR
Simplifying gives:
F−f=12ma(4)
Now we have two equations:
1. F−f=ma (equation 1)
2. F−f=12ma (equation 4)
ma=12ma
Subtracting gives:
12ma=f
Thus, we can express f:
f=12ma
From equation (1):
f=F−ma
Substituting a from f=12ma:
f=F−2f
Rearranging gives:
3f=F⇒f=F3
The frictional force f acting on the cylinder is:
f=F3
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 2 Multiple Correct
36 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 2
1 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 1 Subjective
32 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions

A uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force F is applied at the top point P of the cylinder so that it start pure rolling. The acceleration of the cylinder is
View Solution
A solid sphere of mass ma nd radis R is placed on a rough horizontal surface A horizontal force F is applied to sphere at a height h, (0≤h≤R) from centre. If sphere rolls slipping then,
View SolutionA cylinder of mass m and radius R is kept on a rough surface after giving its centre a horizontal speed v0. Find the speed of the centre of the cylinder when it stops slipping.
View SolutionA solid cylinder of mass M and radius R pure rolls on a rough surface as shown in the figure. Choose the correct alternative (s).

View SolutionA solid cylinder of mass M and radius R rolls without slipping on a flat horizontal surface. Its moment of inertia about the line of contact is
View SolutionA solid sphere of mass M and radius R is placed on a rough horizontal surface. It is stuck by a horizontal cue stick at a height h above the surface. The value of h so that the sphere performs pure rolling motion immediately after it has been stuck is
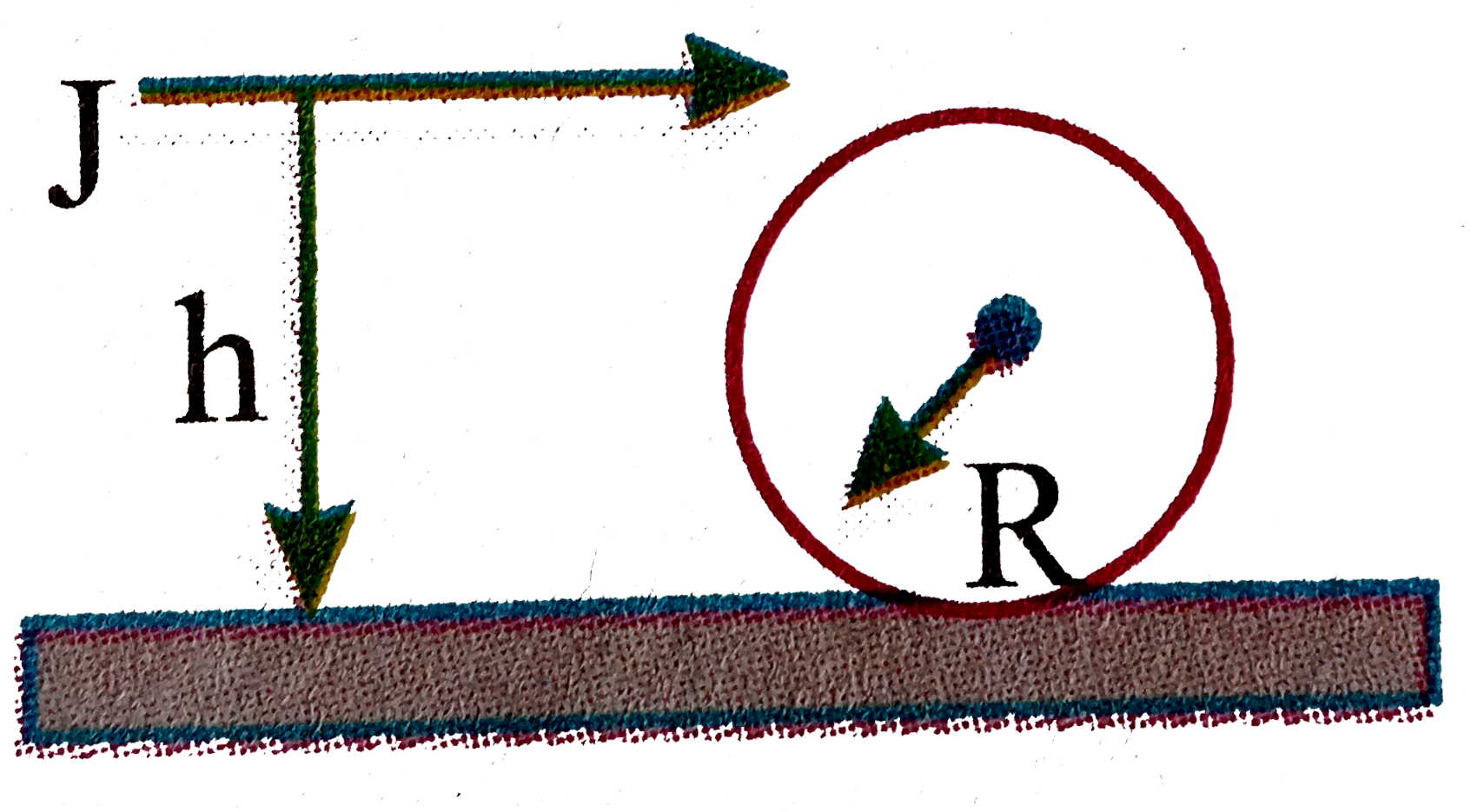
View SolutionA massless string is wrapped around a hollow cylinder having mass m and radius r. The cylinder is kept on a rough horizontal surface (coefficient of friction is μ). A constant force F is applied as shown in the figure. In case of pure rolling, the friction force acting on the bottom most point of the cylinder is
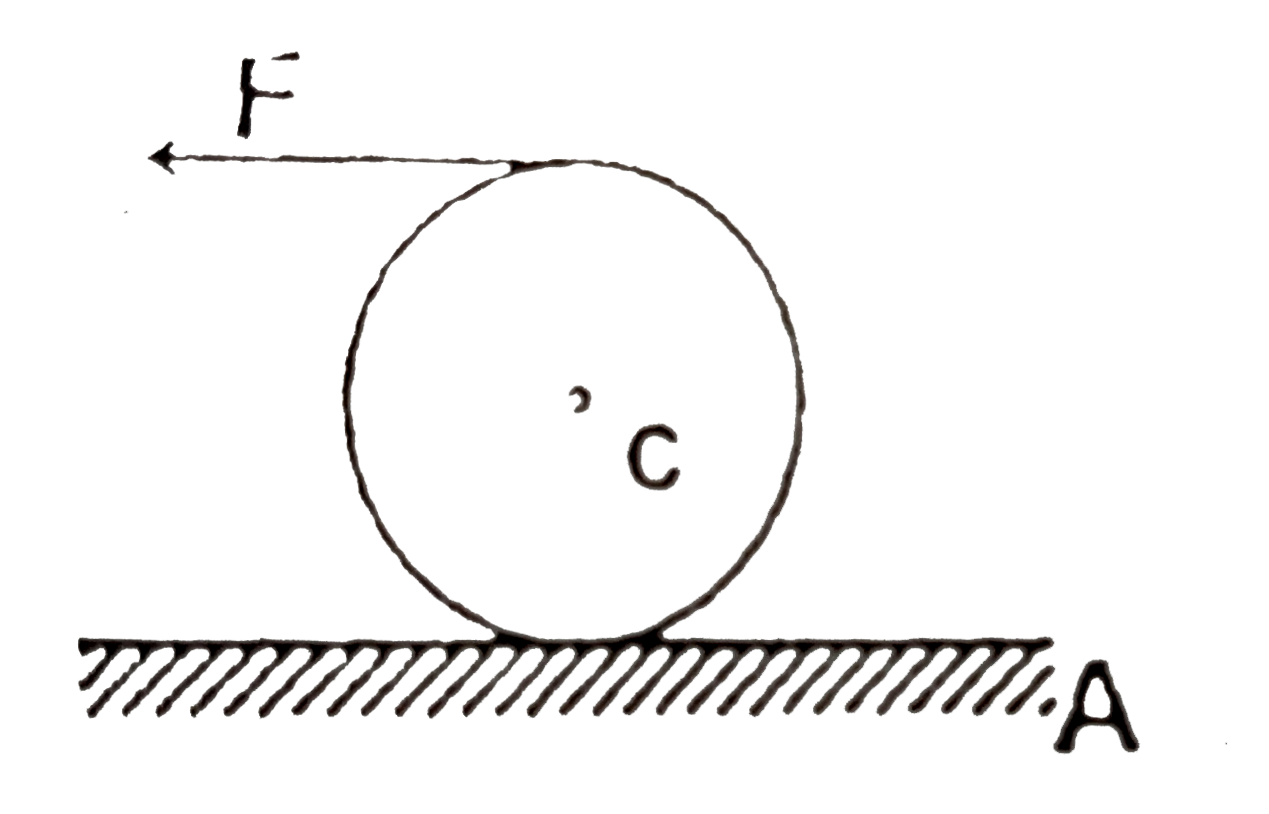
View SolutionA sphere of mass m and radius r is placed on a rough plank of mass M. The system is placed on a smooth horizontal surface. A constant force F is applied on the plank such that the sphere rolls purely on the plank. Find the acceleration of the sphere.

View SolutionA bullet of mass m moving with a velocity of u just grazes the top of a solid cylinder of mass M and radius R resting on a rough horizontal surface as shown and is embedded in the cylinder after impact. Assuming that the cylinder rolls without slipping, find the angular velocity of the cylinder and the final velocity of the bullet.

View SolutionA uniform solid cylindrical roller of mass 'm' is being pulled on a horizontal surface with force F parallel to the surface and applied at its centre. If the acceleration of the cylinder is 'a' and it is rolling without slipping, then the value of 'F' is :−
View Solution
