Using Planck's formula, find the power radiated by a unit area of a black body within a narrow wavelength interval Δλ=1.0nm close to the maximum of spectral radiation density at a temperature T=3000K of the body.
We write the required power in terms of the raadiosity by considering ∂only the enegry radiated in the given range. Then form the pervious problem
ΔP=c4≈u(λm,T)Δλ
= (4pi^(2)c^(2)cancelh)/(lambda_(m)^(5)) (Delta lambda)/(e^(2ch//klambda_(m)T)-1)
But λmT=b
so DeltaP = (4pi^(2)c^(2)cancelh)/(lambda_(m)^(5))(Delta lambda)/(e^(2ch//kb)-1)Delta lambda
Using the data
(2pic cancelh)/(kb) = (2pixx3xx10^(8)xx1.05xx0^(-34))/(1.38xx10^(-23)xx2.9xx10^(-3)) = 4.9643
1e2πch/kb−1=7.03×10−3
and ΔP=0.312W/cm2
Topper's Solved these Questions
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Optics Of Moving Sources
22 Videos
- MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - MECHANICSEXERCISE - Mechanics Problems
92 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
Similar Questions
Temperature of black body is 3000K. When black body cools, then change in wevelength Δλ=9 micron corresponding to maximum energy density. Now temperature of black body is :-
View SolutionPower emitted per unit area of a black body is R watt/m2. At what wavelength will the power radiated by black body be maximum is stefan's constant is σ and Wien's constant is b then.
View Solution
Knowledge Check
Temperature of black body is 3000K. When black body cools, then change in wevelength Δλ=9 micron corresponding to maximum energy density. Now temperature of black body is :-
A300KB2700KC270KD1800KThe power emitted per unit area of a black body is R watt/m2. At what wavelength will the power radiated by the black body be maximum. If the Stefan's constant is σ and Wien's constant is b, then
Aλ=(bsimgaR)1/4Bλ=(bR)1/4Cλ=(dRσ)1/4Dλ=b(σR)1/4Power emitted per unit area of a black body is R watt/m2. At what wavelength will the power radiated by black body be maximum is stefan's constant is σ and Wien's constant is b then.
Aλ=(bσR)14Bλ=(bR)14C(bRσ)14=λDλ=b(σR)14
If a black body is radiating at T=1650K at what wavelenght is the intensity maximum ? .
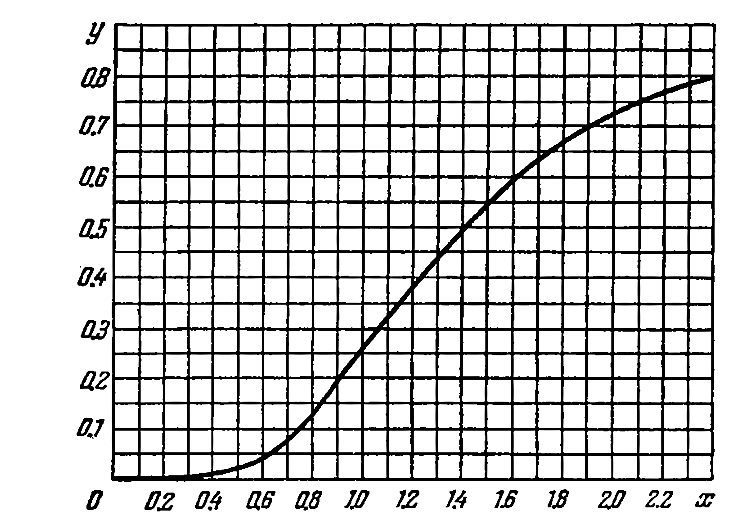
View SolutionFig. shows the plot of the function y(x) representing a fraction of the total power of thermal radiation falling within the spectral interval from 0 to x. Here x=λ/λm(λm is the wavelength corresponding to the maximum of spectral radiation density).
Using this plot, find:
(a) the wavelength which divides the radiation spectrum into two equal (in terms of energy) parts at the temperature 3700K,
(b) the fraction of the total radiation power falling within the visible range of the spectrum (0.40−0.76μm) at the temperature 5000K,
(c) how manu times the power radiated at wavelengths exceeding 0.76μm will increase if the temperature rises form 3000 to 5000K.
View SolutionThe power radiated by a blackbody is P at a certain temperature. If power radiated by this body becomes 116th the times. then wavelength corresponding to maximum intensity becomes
View SolutionThe power radiated by a blackbody is P at a certain temperature. If power radiated by this body becomes 116th the times. then wavelength corresponding to maximum intensity becomes
View SolutionA black body emits maximum radiation of wavelength λ1=2000 Å at a certain temperature T1. On increasing the temperature , the total energy of radiation emitted is increased 16 times at temperature T2. If λ2 is the wavelength corresponding to which maximum radiation emitted at temperature T2. Calculate the value of (λ1λ2)
View Solution
