Vector A is pointing eastwards and vector B northwards .If then match the following two columns.
The correct Answer is:Ap, Bs , C q, Ds
To solve the problem step by step, we will analyze the vectors A and B, their operations, and match the results with the options provided.
Step 1: Define the Vectors
- Vector A is pointing eastward, which we can represent as →A=|A|^i.
- Vector B is pointing northward, which we can represent as →B=|B|^j.
- Given that |→A|=|→B|, we can denote their magnitudes as |A|=|B|=k.
Step 2: Calculate →A+→B
- The sum of the two vectors is:
→A+→B=|A|^i+|B|^j=k^i+k^j
- This resultant vector is at a 45-degree angle to both the east and north directions, pointing towards the north-east.
- Match: This corresponds to option P ("north-east").
Step 3: Calculate →A−→B
- The difference of the two vectors is:
→A−→B=|A|^i−|B|^j=k^i−k^j
- This resultant vector is also at a 45-degree angle, but it points towards the south-east direction.
- Match: This corresponds to option R ("south-east").
Step 4: Calculate →A×→B
- The cross product of the two vectors is:
→A×→B=|A||B|(^i×^j)=k2^k
- The direction of this vector is vertically upwards (along the z-axis).
- Match: This corresponds to option Q ("vertically upwards").
Step 5: Calculate (→A×→B)×(→A×→B)
- Since →A×→B=k2^k, we have:
(→A×→B)×(→A×→B)=(k2^k)×(k2^k)=0
- The cross product of any vector with itself is zero, which has no direction.
- Match: This corresponds to option S ("None").
Final Matches
- A: →A+→B → P ("north-east")
- B: →A−→B → R ("south-east")
- C: →A×→B → Q ("vertically upwards")
- D: (→A×→B)×(→A×→B) → S ("None")
- Vector A is pointing eastward, which we can represent as →A=|A|^i.
- Vector B is pointing northward, which we can represent as →B=|B|^j.
- Given that |→A|=|→B|, we can denote their magnitudes as |A|=|B|=k.
- The sum of the two vectors is:
→A+→B=|A|^i+|B|^j=k^i+k^j
- This resultant vector is at a 45-degree angle to both the east and north directions, pointing towards the north-east.
- Match: This corresponds to option P ("north-east").
- The difference of the two vectors is:
→A−→B=|A|^i−|B|^j=k^i−k^j
- This resultant vector is also at a 45-degree angle, but it points towards the south-east direction.
- Match: This corresponds to option R ("south-east").
- The cross product of the two vectors is:
→A×→B=|A||B|(^i×^j)=k2^k
- The direction of this vector is vertically upwards (along the z-axis).
- Match: This corresponds to option Q ("vertically upwards").
- Since →A×→B=k2^k, we have:
(→A×→B)×(→A×→B)=(k2^k)×(k2^k)=0
- The cross product of any vector with itself is zero, which has no direction.
- Match: This corresponds to option S ("None").
- A: →A+→B → P ("north-east")
- B: →A−→B → R ("south-east")
- C: →A×→B → Q ("vertically upwards")
- D: (→A×→B)×(→A×→B) → S ("None")
Topper's Solved these Questions
Similar Questions
If θ is the abgle between two vectors A and B , then match the following two columns.
Column I Column II(A)A.B=|A×B|(P)θ=90∘(B)A.B=B2(Q)θ=0∘or180∘(C)|A+B|=|A−C|(r)A=B(D)|A×B|=AB(s)None
View SolutionTwo vector A and B have rqual magnitude x .Angle between tjem is 60∘ Then match the following two columns.
Column I Column II(A)|A+B|(P)√32x2(B)|A−B|(Q)x(C)A.B(r)√3x(D)|A×B|(s)None
View Solution
Knowledge Check
Match the Column I and Column II.,B
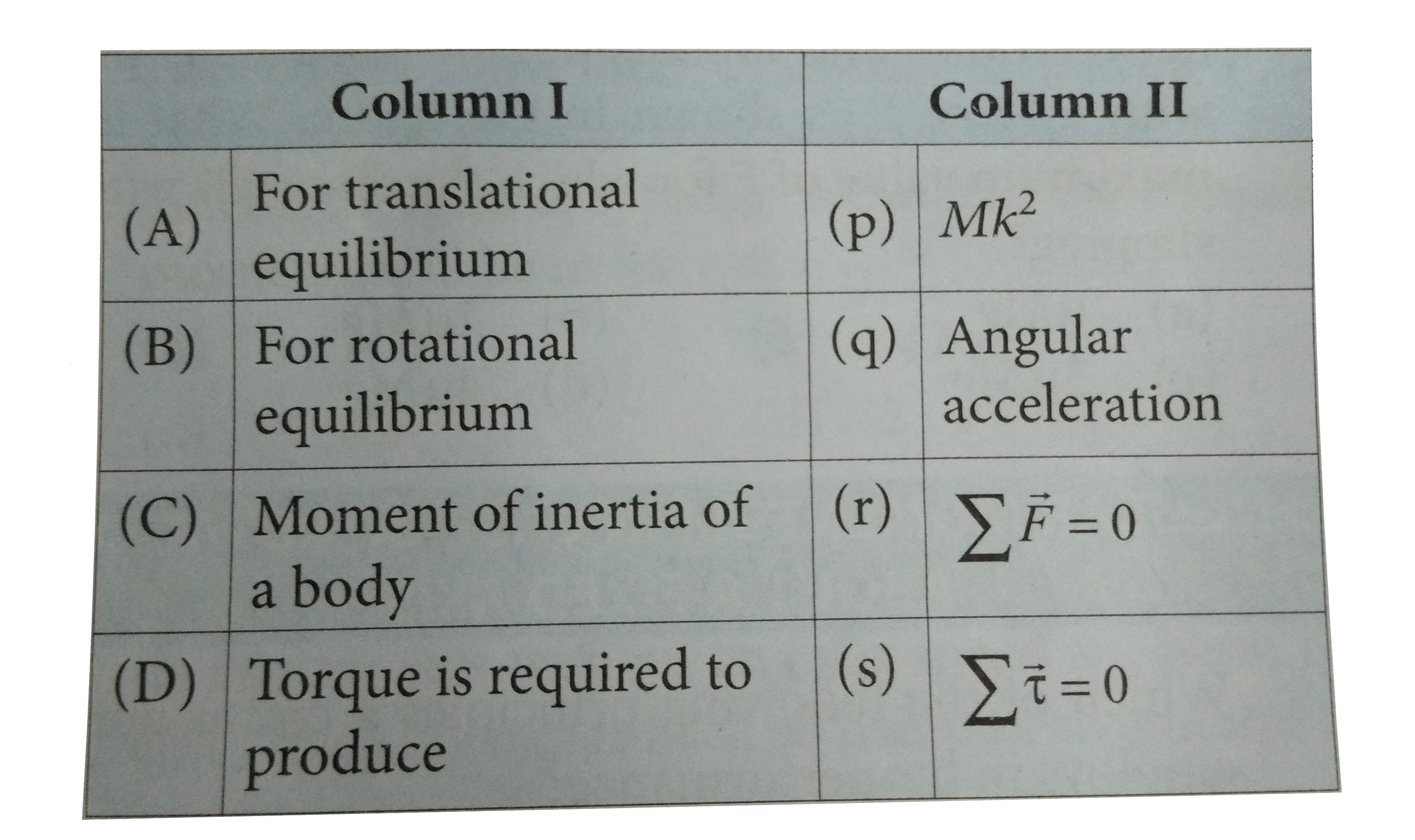 AA-p, B-q, C-r, D-sBA-q, B-r, C-s. D-pCA-r, B-q, C-p, D-pDA-r, B-s, C-p, D-q
AA-p, B-q, C-r, D-sBA-q, B-r, C-s. D-pCA-r, B-q, C-p, D-pDA-r, B-s, C-p, D-q
Match the following words in column A with those is column B :

View SolutionColumn IColumn II(A)R/L(p)Time(B)C/R(q)Frequency(C)E/B(r)Speed(D)√ε0μ0(s)None
View SolutionMatch the following columns.
∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣Column IColumn II(A)d v/dt(p)Acceleration(B)d|v|/dt(q)Magnitude of acceleration(C)drdt(r)Velocity(D)∣∣drdt∣∣(s)Magnitude of velocity(t)Rate of change of speed∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionMatch the columns
Column-AColumn-B(a)Ribs(i)26(b)Vertebrae(ii)14(c)Phalanges(iii)1(d)Sternum(iv)24
View SolutionMatch the following
Column-IColumn-II(A)Enthalpy(p)Extensive property(B)Entropy(q)H - TS(C)Free energy(r)E + PV(D)Pressure(s)Intensive property
View SolutionMatch the following Column -I Column -II (A) Aerosol (p) Optical property(B) Emulsion (q) Electrical property(C) Tyndall effect (r) Smoke(D) Dialysis (s) Medicines
View SolutionMatch the following columns.
Column IColumn IIA.Teslap[ML2A−2T−2]B.Weberq[MLA−2T−1]C.Weber m−2r.[MA−1T−2]D.Henrys.None
View Solution
