Water is boiled in a rectangular steel tank of thickness 2 cm by a constant temperature furnace. Due to vaporisation, water level falls at a steady rate of 1 cm in 9 minutes. Calculate the temperature of the furnace. Given K for steel 0.2cals−1m−1.∘C−1
The correct Answer is:110∘C
To solve the problem, we will follow these steps:
Step 1: Understand the Given Information
We have a rectangular steel tank with:
- Thickness of the tank, x=2 cm = 0.02 m
- Rate of water level falling = 1 cm in 9 minutes = 1cm9minutes=1cm540seconds
- The thermal conductivity of steel, K=0.2cal s−1m−1°C−1
Step 2: Calculate the Volume of Water Vaporized
The volume of water that vaporizes in 9 minutes can be expressed as:
- Volume = Area A (in cm²) × height of water vaporized (in cm)
- Height of water vaporized in 9 minutes = 1 cm
- Therefore, Volume = A×1cm3=Acm3
Step 3: Convert Volume to Mass
Using the density of water (1 g/cm³):
- Mass of water vaporized = Volume × Density = Ag
Step 4: Calculate the Heat Required for Vaporization
The heat Q required to vaporize this mass of water can be calculated using the latent heat of vaporization L:
- Q=mL=A×L
- Assuming L (latent heat of vaporization) = 540 cal/g (for water), we get:
- Q=A×540cal
Step 5: Apply Fourier's Law of Heat Conduction
According to Fourier's law:
Q=K⋅A⋅T1−T2x⋅t
Where:
- T1 = Temperature of the furnace
- T2 = Temperature of the water (assumed to be 100°C)
- x = Thickness of the tank (0.02 m)
- t = Time (540 seconds)
Step 6: Rearranging the Equation
Substituting Q into the equation:
A⋅540=K⋅A⋅T1−T2x⋅t
Cancelling A from both sides (assuming A≠0):
540=K⋅T1−T2x⋅t
Step 7: Substitute Known Values
Substituting K=0.2cal s−1m−1°C−1, x=0.02m, and t=540s:
540=0.2⋅T1−1000.02⋅540
Step 8: Simplify the Equation
Cancelling 540 from both sides:
1=0.2⋅T1−1000.02
1=10(T1−100)
T1−100=0.1
T1=100+10=110°C
Final Answer
The temperature of the furnace is T1=110°C.
---
We have a rectangular steel tank with:
- Thickness of the tank, x=2 cm = 0.02 m
- Rate of water level falling = 1 cm in 9 minutes = 1cm9minutes=1cm540seconds
- The thermal conductivity of steel, K=0.2cal s−1m−1°C−1
The volume of water that vaporizes in 9 minutes can be expressed as:
- Volume = Area A (in cm²) × height of water vaporized (in cm)
- Height of water vaporized in 9 minutes = 1 cm
- Therefore, Volume = A×1cm3=Acm3
Using the density of water (1 g/cm³):
- Mass of water vaporized = Volume × Density = Ag
The heat Q required to vaporize this mass of water can be calculated using the latent heat of vaporization L:
- Q=mL=A×L
- Assuming L (latent heat of vaporization) = 540 cal/g (for water), we get:
- Q=A×540cal
According to Fourier's law:
Q=K⋅A⋅T1−T2x⋅t
Where:
- T1 = Temperature of the furnace
- T2 = Temperature of the water (assumed to be 100°C)
- x = Thickness of the tank (0.02 m)
- t = Time (540 seconds)
Substituting Q into the equation:
A⋅540=K⋅A⋅T1−T2x⋅t
Cancelling A from both sides (assuming A≠0):
540=K⋅T1−T2x⋅t
Substituting K=0.2cal s−1m−1°C−1, x=0.02m, and t=540s:
540=0.2⋅T1−1000.02⋅540
Cancelling 540 from both sides:
1=0.2⋅T1−1000.02
1=10(T1−100)
T1−100=0.1
T1=100+10=110°C
The temperature of the furnace is T1=110°C.
Topper's Solved these Questions
- THERMODYNAMICSBOOK - SL ARORACHAPTER - THERMODYNAMICSEXERCISE - Exercise
342 Videos
- THERMODYNAMICSBOOK - SL ARORACHAPTER - THERMODYNAMICSEXERCISE - Problems from competitive examinations
22 Videos
- Thermal Properties of MatterBOOK - SL ARORACHAPTER - Thermal Properties of MatterEXERCISE - Exercise
449 Videos
- Units and MeasurementsBOOK - SL ARORACHAPTER - Units and MeasurementsEXERCISE - Exercise
499 Videos
Similar Questions
A rectangular steel tank of thickness 3 cm contains water. The water in the tank is boiled by a furnace. The level of water falls at a constant rate of 1.5 cm in 15 minutes. What will be the temperature of furnace if K for steel is 0.2cals−1m−1∘C−1 ?
View SolutionA rectangular steel tank of thickness 2.5 cm is used to boil water by a constant temperature furnace. If the level of water inside the tank falls at a steady rate of 0.25 cm in 2 minutes due to vaporisation, find the temperature of the furnace.
Take, conductivity of steel =0.2cals−1m−1∘C−1
Latent heat of steam = 540 cal/g
View SolutionCalculate the difference in temperature between two sides of an iron plate 20 m m thick, when heat is conducted at the rate of 6×105 cal/min/m2. K for metal is 0.2 cals−1cm−1.∘C−1
View SolutionSteam at 100∘C is passed into a copper cylinder 10 mm thick and of 200cm2 area. Water at 100∘C collects at the rate of 150g−1min. Find the temperature of the outer surface, if the conductivity of copper is 0.8cals−1cm−1.∘C−1 and the latent heat of steam is 540calg−1.
View SolutionA uniform steel rod of length 50 cm is insulated on its sides. There is a layer of water of thick ness 0.2 mm at each end of the rod. If the ends of the rod are exposed to ice at 0∘C, and steam at 100∘C, calculate the temperature gradient in the rod. Thermal conductivities of steel and water are 0.11calcm−1s−1∘C−1and1.5×10−3calcm−1s−1∘C−1
View SolutionWhat will be the difference in temperatures between two sides of a metallic plate 10 mm thick if heat is conducted at the rate of 5×105 cal/min/m2 . K for metal is 0.7cals−1cm−1∘C−1 .
View SolutionOne end of a brass rod 2m long and having 1cm radius is maintained at 250∘C. When a steady state is reached , the rate of heat flow acrss any cross-section is 0.5cals−1. What is the temperature of the other end K=0.26cals−1cm−1.∘C−1.
View SolutionA platinum balls of mas 100g is remoived from a furnace and immersed in a copper vessel of mass 100g containing water of mass 390g at 30∘C. The temperature of water rises to 40∘C. Caltulate the temperature of the furnace. (Given that the specific heat of platinum =168kg−1K−1, specific heat capacity of copper =420Jkg−1K−1 and specific heat capacity of water =4200Jkg−1K−1)
View SolutionThe height of Niagra falls is 50 m. Calculate the difference in temperature of water at the top and at the bottom of fall, if J=4.2Jcal−1.
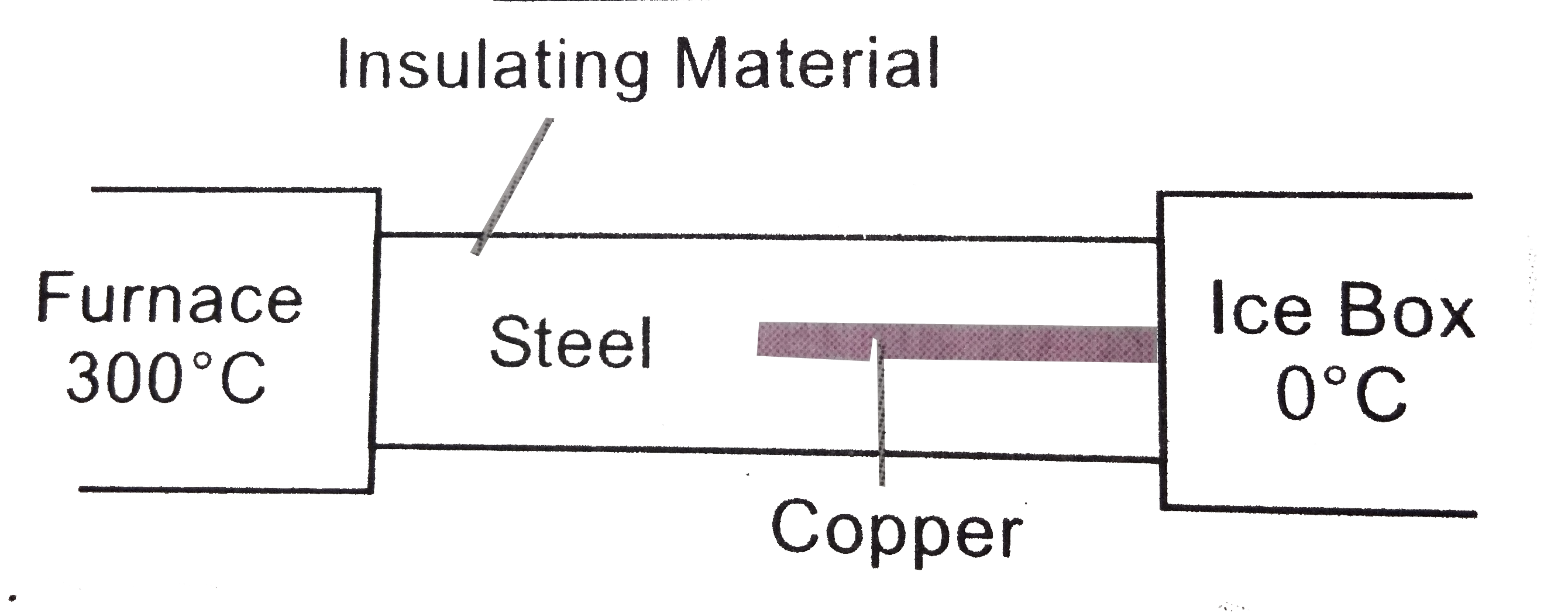
View SolutionWhat is the temperature of the steel-copper junction in the steady state system show in Fig. 7(e).17? Length of steel rod = 15.0 cm, length of the copper rod = 10.0 cm, temperature of the furnace =300∘C, temperature of other end 0∘C. The are of cross-section of the steel rod is twice that of the copper rod. (Thermal conductivity of steel =50.2js−1m−1K−1 and of copper =3895js−1m−1K−1).
View Solution
