A uniform steel rod of length 50 cm is insulated on its sides. There is a layer of water of thick ness 0.2 mm at each end of the rod. If the ends of the rod are exposed to ice at , and steam at calculate the temperature gradient in the rod. Thermal conductivities of steel and water are
To solve the problem of finding the temperature gradient in a uniform steel rod with water layers at both ends, we can follow these steps:
Step 1: Understand the Setup
We have a steel rod of length L=50cm=0.5m insulated on its sides. There are water layers of thickness x=0.2mm=0.0002m at each end. One end of the rod is at 0∘C (ice) and the other end is at 100∘C (steam).
Step 2: Identify Thermal Conductivities
The thermal conductivities are given as:
- Steel: ks=0.11calcm−1s−1∘C−1=0.11×104calm−1s−1∘C−1 (since 1cm=0.01m)
- Water: kw=1.5×10−3calcm−1s−1∘C−1=1.5×10−3×104calm−1s−1∘C−1
Step 3: Calculate the Total Thermal Resistance
The total thermal resistance Rth of the system can be calculated as the sum of the resistances of the water layers and the steel rod:
Rth=Rw1+Rs+Rw2
Where:
- Rw1=xkwA (resistance of the first water layer)
- Rs=LksA (resistance of the steel rod)
- Rw2=xkwA (resistance of the second water layer)
Substituting the values, we get:
Rth=0.0002kwA+0.5ksA+0.0002kwA
Rth=0.0004kwA+0.5ksA
Step 4: Apply Fourier's Law
According to Fourier's law of heat conduction, the heat flow DQDT is given by:
DQDT=T1−T2Rth
Where T1=100∘C and T2=0∘C.
Step 5: Calculate the Temperature Gradient
The temperature gradient T1−T2L can be expressed as:
T1−T2L=100Rth
Substituting Rth:
T1−T2L=1000.0004kwA+0.5ksA
Step 6: Solve for the Gradient
Now, substituting the values of kw and ks:
1. Convert kw and ks to consistent units.
2. Calculate Rth using the above equation.
3. Finally, compute T1−T2L.
Final Calculation
After substituting the values and simplifying, we find:
T1−T2L≈189.03°C/m
Conclusion
The temperature gradient in the rod is approximately 189.03°C/m.
---
We have a steel rod of length L=50cm=0.5m insulated on its sides. There are water layers of thickness x=0.2mm=0.0002m at each end. One end of the rod is at 0∘C (ice) and the other end is at 100∘C (steam).
The thermal conductivities are given as:
- Steel: ks=0.11calcm−1s−1∘C−1=0.11×104calm−1s−1∘C−1 (since 1cm=0.01m)
- Water: kw=1.5×10−3calcm−1s−1∘C−1=1.5×10−3×104calm−1s−1∘C−1
The total thermal resistance Rth of the system can be calculated as the sum of the resistances of the water layers and the steel rod:
- Rw1=xkwA (resistance of the first water layer)
- Rs=LksA (resistance of the steel rod)
- Rw2=xkwA (resistance of the second water layer)
According to Fourier's law of heat conduction, the heat flow DQDT is given by:
The temperature gradient T1−T2L can be expressed as:
Now, substituting the values of kw and ks:
2. Calculate Rth using the above equation.
3. Finally, compute T1−T2L.
After substituting the values and simplifying, we find:
The temperature gradient in the rod is approximately 189.03°C/m.
Topper's Solved these Questions
- HEAT TRANSFER BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - HEAT TRANSFER EXERCISE - Practice Exercise 4.2
8 Videos
- HEAT TRANSFER BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - HEAT TRANSFER EXERCISE - Practice Exercise 4.3
5 Videos
- HEAT TRANSFER BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - HEAT TRANSFER EXERCISE - Illustrative Example 4.29
1 Videos
- HEAT AND THERMAL EXPANSIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - HEAT AND THERMAL EXPANSIONEXERCISE - UNSOLVED NUMRICAL PROBLEMS FOR PREPARATION OF NSEP, INPhO & IPhO
82 Videos
- Kinetic Theory of Gases and Gas LawsBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - Kinetic Theory of Gases and Gas LawsEXERCISE - Unsolved Numerical Problems for Preparation of NSEP, INPhO & IPhO
64 Videos
Similar Questions
A uniform copper rod 50 cm long is insulated on the sides, and has its ends exposed to ice and steam, respectively. If there is a layer of water 1 mm thick at each end, calculate the temperature gradient in the bar. The thermal conductivity of copper is 436Wm−1K−1 and that of water is 0.436Wm−1K−1.
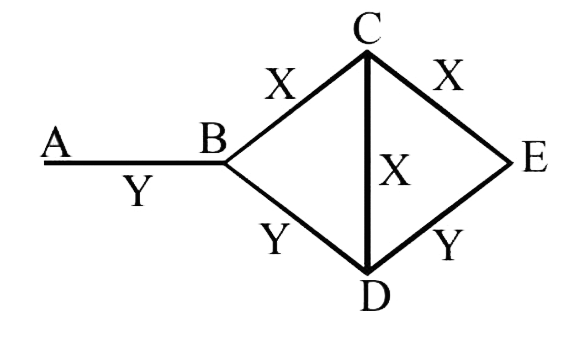
View SolutionThree rods of material X and three rods of material Y are connected as shown in the figure. All the rods are of identical length and cross-sectional area. If the end A is maintained at 60∘C and the junction E at 10∘C. Calculate the temperature of the junction B, C and D. The thermal conductivity of X is 0.92cal/sec−cm∘C and that of Y is 0.46cal/sec−cm−∘C.
View Solution
Knowledge Check
A steel rod of length 100 cm is clamped at the middle. The frequency of the fundamental mode for the longitudinal vibrations of the rod is
(Speed of sound in steel = 5 km s−1)A1.5 KHzB2 KHzC2.5 KHzD3 KHzThe end A of rod AB of length 1 m is maintained at 80∘C and the end B at 0∘C. The temperature at a distance of 60 cm from the end A is
A16∘CB32∘CC48∘CD64∘CIn a steady state of heat conduction the temperature of the ends A and B of a rod 100cm long per 0∘C and 100∘C The temperature of the rod at a point 60cm distant from the end A is .
A0∘CB40∘CC60∘CD100∘C
A uniform copper bar 100 cm long is insulated on side, and has its ends exposed to ice and steam respectively. If there is a layer of water 0.1 mm thick at each end, calculate the temperature gradient in the bar. KCu=1.04 and Kwater=0.0014 in CGS units.
View SolutionThe ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View SolutionFigure shows a copper rod joined to a steel rod. The rods have equal length and and the equal cross sectional area. The free end of the copper rod is kept at 0∘C and that of the steel rod is kept at 100∘C . Find the temperature at the junction of the rods. conductivity of copper =390WM−1^(@)C^(-1)andtˆofsteel=46Wm^(-1)(∘)C−1 .

View SolutionA large cylindrical rod of length L is made by joining two identical rods of copper and steel of length (L2) each. The rods are completely insulated from the surroundings. If the free end of copper rod is maintained at 100∘C and that of steel at 0∘C then the temperature of junction is (Thermal conductivity of copper is 9 times that of steel)
View SolutionThe ends of copper rod of length 1m and area of cross section 1cm2 are maintained at 0∘C and 100∘C. At the centre of rod, there is a source of heat of 32W. The thermal conductivity of copper is 400W/mK

View Solution
