What are binary stars? Describe their motion in terms of centre of mass.
Step-by-Step Solution:
1. Definition of Binary Stars:
Binary stars are systems consisting of two stars that revolve around a common center of mass due to their mutual gravitational attraction.
2. Understanding the Center of Mass:
In a binary star system, both stars orbit a point called the center of mass (COM). The position of the center of mass can be calculated using the formula:
m1r1=m2r2
where m1 and m2 are the masses of the two stars, and r1 and r2 are the distances from the center of mass to each star.
3. Distance Between the Stars:
The total distance r between the two stars is given by:
r=r1+r2
4. Finding Distances r1 and r2:
From the center of mass equation, we can express r1 and r2 in terms of the total distance r:
r1=m2m1+m2r
r2=m1m1+m2r
5. Orbital Motion and Centripetal Acceleration:
Each star moves in a circular orbit around the center of mass. The orbital velocity V1 of star 1 and the centripetal acceleration ac1 can be expressed as:
ac1=V21r1
6. Gravitational Force:
The gravitational force Fg between the two stars is given by:
Fg=Gm1m2r2
where G is the gravitational constant.
7. Equating Forces:
The gravitational force acting on star 1 can be equated to the centripetal force:
Gm1m2r2=m1ac1
Substituting ac1 gives:
Gm1m2r2=m1V21r1
8. Solving for Orbital Velocity:
Rearranging the equation allows us to find the square of the orbital velocity V21:
V21=Gm2r1r2
9. Time Period Calculation:
The time period T1 for star 1 can be calculated using:
T1=2πr1V1
Similarly, for star 2:
T2=2πr2V2
10. Conclusion:
The motion of binary stars can be described in terms of their center of mass, the gravitational forces acting between them, and their respective orbital velocities and periods.
---
Binary stars are systems consisting of two stars that revolve around a common center of mass due to their mutual gravitational attraction.
In a binary star system, both stars orbit a point called the center of mass (COM). The position of the center of mass can be calculated using the formula:
m1r1=m2r2
where m1 and m2 are the masses of the two stars, and r1 and r2 are the distances from the center of mass to each star.
The total distance r between the two stars is given by:
r=r1+r2
From the center of mass equation, we can express r1 and r2 in terms of the total distance r:
r1=m2m1+m2r
r2=m1m1+m2r
Each star moves in a circular orbit around the center of mass. The orbital velocity V1 of star 1 and the centripetal acceleration ac1 can be expressed as:
ac1=V21r1
The gravitational force Fg between the two stars is given by:
Fg=Gm1m2r2
where G is the gravitational constant.
The gravitational force acting on star 1 can be equated to the centripetal force:
Gm1m2r2=m1ac1
Substituting ac1 gives:
Gm1m2r2=m1V21r1
Rearranging the equation allows us to find the square of the orbital velocity V21:
V21=Gm2r1r2
The time period T1 for star 1 can be calculated using:
T1=2πr1V1
Similarly, for star 2:
T2=2πr2V2
The motion of binary stars can be described in terms of their center of mass, the gravitational forces acting between them, and their respective orbital velocities and periods.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - REVISION EXERCISES (Long Answer Questions Carrying 5 marks)8videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - REVISION EXERCISES (Numerical Problems)20videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - REVISION EXERCISES (Fill in the Blanks Carrying 1 mark )9videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)6videos
PHYSICAL WORLD
- BOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST15videos
THERMAL PROPERTIES OF MATTER
Similar Questions
Knowledge Check
- Question 1 - Select One
List-I shows some arrangements in which motion of masses are described and list-II defines motion of centre of mass of the system (m + M). Match appropriate possible options in list-II
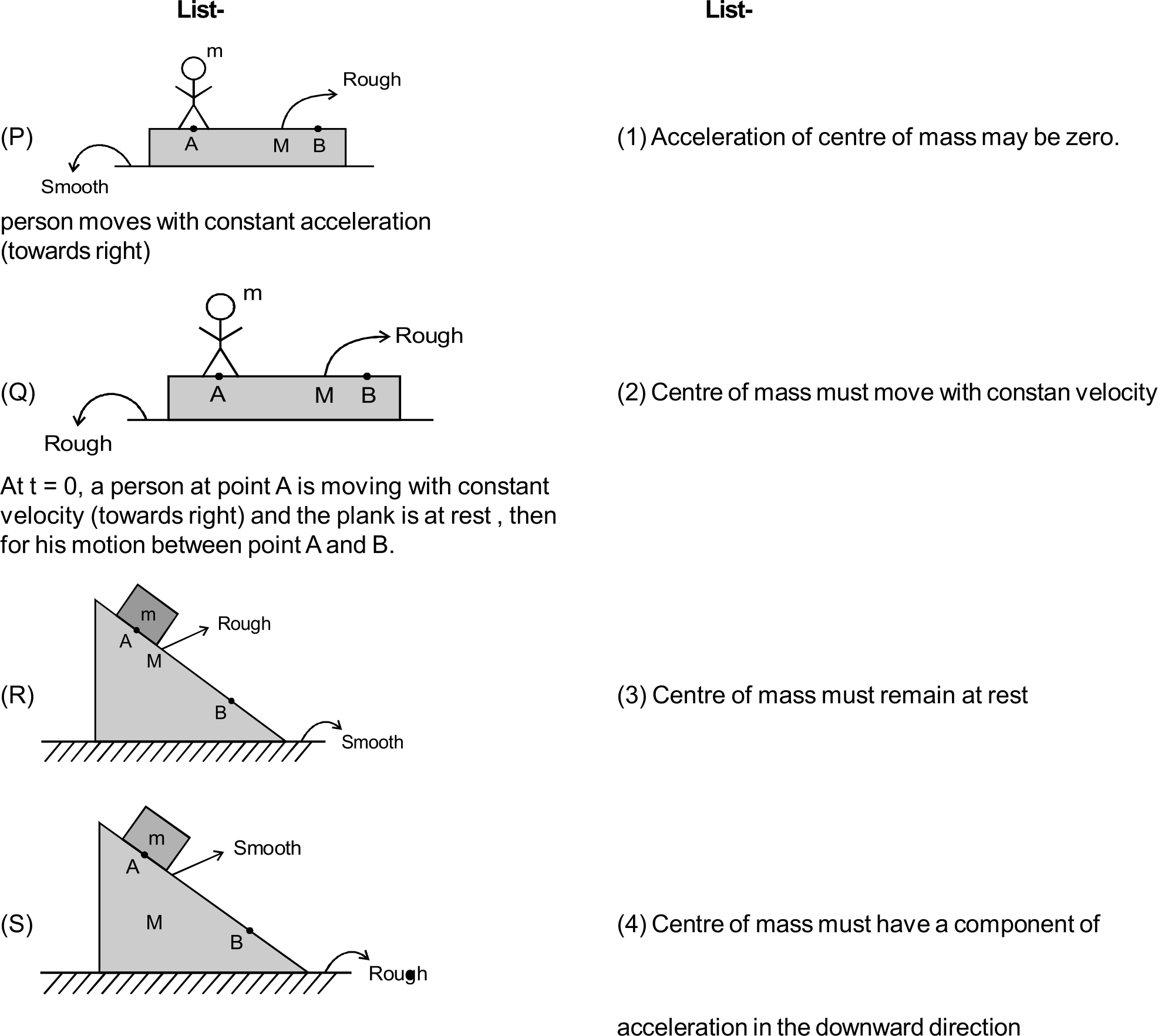 APQRS(A)3124BPQRS(B)3214CPQRS(C)4213DPQRS(D)4123
APQRS(A)3124BPQRS(B)3214CPQRS(C)4213DPQRS(D)4123 - Question 2 - Select One
Two bodies AandB initially at rest are attrached towards each other due to gravitation. Given that A is much heavier. Than B. Which of the followings correctly describes the relative motion of the centre of mass of the bodies ?
Ait moves towards ABit moves towards BCit moves perpendicular to the line joining the particlesDit remains at rest. - Question 3 - Select One or More
A double star is a system of two stars of masses m and 2m, rotating about their centre of mass only under their mutual gravitational attraction. If r is the separation between these two stars then their time period of rotation about their centre of mass will be proportional to
Ar32BrCm12Dm−12
Motion Of Centre Of Mass
View SolutionMotion OF centre OF mass
View SolutionDiscuss atleast three examples of the motion of centre of mass.
View SolutionQuestions|Motion Of Centre Of Mass
View SolutionIf there are no external forces, the centre of mass of a double star moves like a free particle.
If we go to the centre of mass frame, then we find that the two starts are moving in a circle about the centre of mass, which is at rest.View Solution

