Which of the following transitions gives photon of maximum energy
n=1 to n=2
n=2 to n=1
n=2 to n=6
n=6 to n=2
The correct Answer is:B
To determine which transition gives a photon of maximum energy, we need to analyze the energy transitions between different energy levels in an atom. The energy of a photon emitted during a transition can be calculated using the formula for the energy levels of a hydrogen atom:
En=−13.6eVn2
where En is the energy of the level corresponding to the principal quantum number n.
1. Identify the transitions: The given transitions are:
- Transition 1: n=1 to n=2
- Transition 2: n=2 to n=1
- Transition 3: n=2 to n=6
- Transition 4: n=6 to n=1
2. Determine the energy change for each transition:
- For the transition n=1 to n=2:
E1→2=E2−E1=(−13.622)−(−13.612)=−13.64+13.6=13.6(1−14)=13.6×34=10.2eV
- For the transition n=2 to n=1:
E2→1=E1−E2=(−13.612)−(−13.622)=−13.6+13.64=13.6(−1+14)=13.6×−34=−10.2eV
(Note: This transition emits a photon, so we take the absolute value for energy.)
- For the transition n=2 to n=6:
E2→6=E6−E2=(−13.662)−(−13.622)=−13.636+13.64=13.6(14−136)
=13.6(936−136)=13.6×836=3.02eV
- For the transition n=6 to n=1:
E6→1=E1−E6=(−13.612)−(−13.662)=−13.6+13.636=13.6(−1+136)
=13.6(−3636+136)=13.6×−3536≈−13.2eV(taking absolute value)
3. Compare the energies:
- E1→2=10.2eV
- E2→1=10.2eV
- E2→6=3.02eV
- E6→1≈13.2eV
4. Conclusion: The transition that gives the photon of maximum energy is n=6 to n=1 with an energy of approximately 13.2eV.
Final Answer:
The transition that gives a photon of maximum energy is from n=6 to n=1.
- Transition 1: n=1 to n=2
- Transition 2: n=2 to n=1
- Transition 3: n=2 to n=6
- Transition 4: n=6 to n=1
- For the transition n=1 to n=2:
E1→2=E2−E1=(−13.622)−(−13.612)=−13.64+13.6=13.6(1−14)=13.6×34=10.2eV
E2→1=E1−E2=(−13.612)−(−13.622)=−13.6+13.64=13.6(−1+14)=13.6×−34=−10.2eV
(Note: This transition emits a photon, so we take the absolute value for energy.)
E2→6=E6−E2=(−13.662)−(−13.622)=−13.636+13.64=13.6(14−136)
=13.6(936−136)=13.6×836=3.02eV
E6→1=E1−E6=(−13.612)−(−13.662)=−13.6+13.636=13.6(−1+136)
=13.6(−3636+136)=13.6×−3536≈−13.2eV(taking absolute value)
- E1→2=10.2eV
- E2→1=10.2eV
- E2→6=3.02eV
- E6→1≈13.2eV
The transition that gives a photon of maximum energy is from n=6 to n=1.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - EXERCISE - II19videos
ATOMS
- BOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - PRACTICE EXERCISE21videos
ATOMS
- BOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - PROBLEM24videos
ATOMS
- BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - LAW OF MOTION128videos
APPENDICES (REVISION EXERCISE)
- BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - PRACTICE SHEET (ADVANCED) (Integer Type Questions)2videos
CAPACITORS
Similar Questions
Which of the following transition will emit maximum energy in hydrogen atom ?
View SolutionThe energy required for transition in a Hydrogen atom from 1st energy level to the 2nd energy level is E. Then , which of the following transitions is possible for the same energy in Helium atom ?
View Solution
Knowledge Check
- Question 1 - Select One
Which of the following transitions involves maximum amount of energy?
AM−(g)→M(g)BM(g)→M+(g)CM+(g)→M2+(g)DM2+(g)→M3+(g) - Question 2 - Select One
Which of the following transitions involves maximum amount of energy?
AMtoM++e−BM−toM++2e−CM2+toM3++e−DM+toM2++e−
In figure E1andE2 represent some of the energy levels of the hydrogen atom.
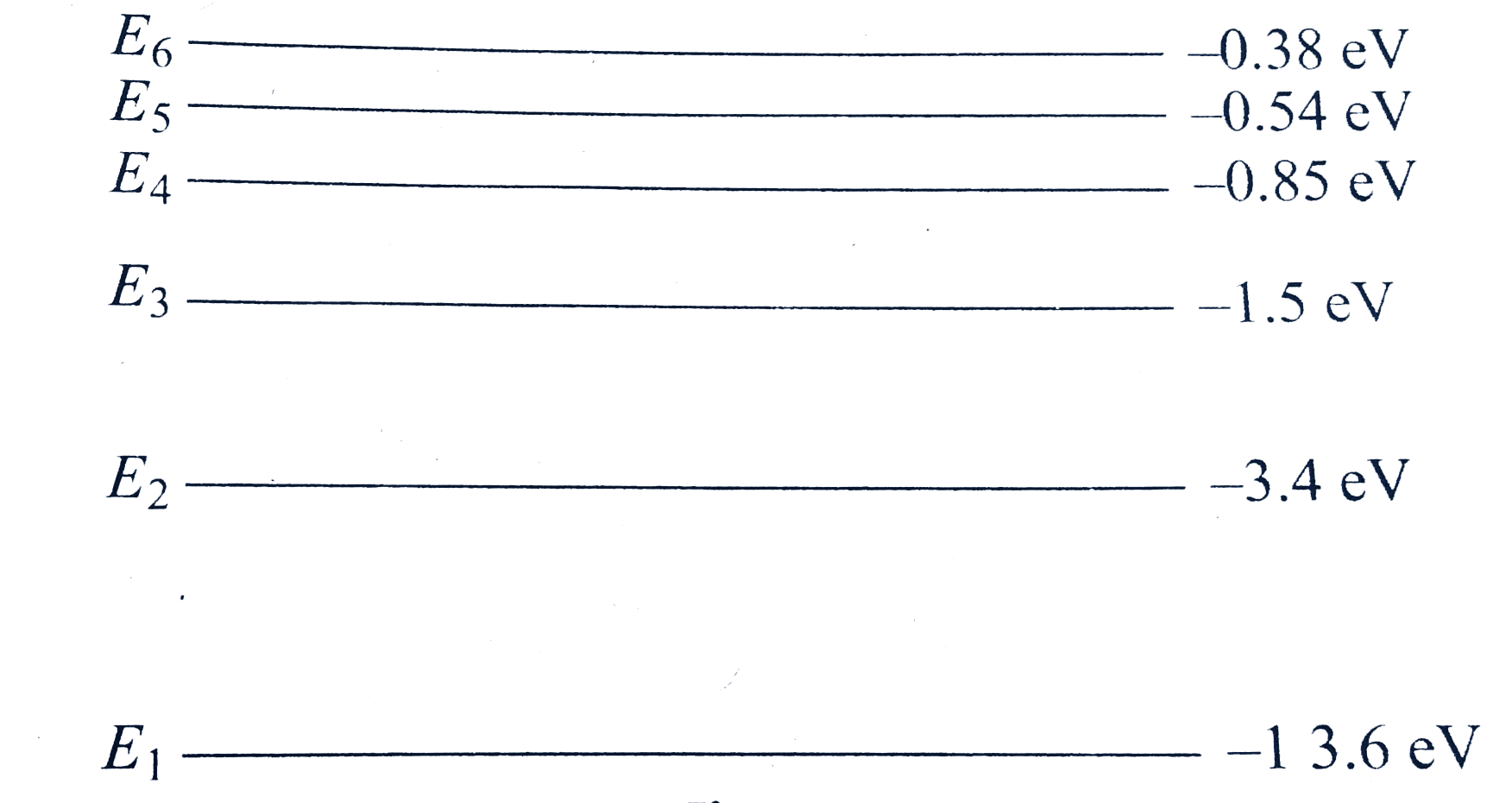
Which one of the following transitions produced a photon of wavelength in the ultraviolet region of the electromagnetic spectrum?View SolutionWhich of the following transitions in a hydrogen atom emits photon of the highest frequency ?
View SolutionWhich of the following ions has the maximum hydration energy?
View SolutionWhich of the following ions has the maximum hydration energy?
View SolutionWhich of the following species absorb maximum energy in its HOMO−LUMO electronic transition?
View SolutionWhich of the following species absorb maximum energy in its HOMO−LUMO electronic transition?
View Solution

