With the help of suitable diagram, sign conventions and assumptions, derive lens Maker's formula for a convex lens.Solution in Punjabi

Video Solution

Text Solution

Verified by Experts

Lens maker.s formula, A formula which relates the focal length of a lns, refractive index of the material of the lens and radii of curvature of two refracting spherical surfaces is known as lens maker.s formula.
The lens manufacturers use this formula to design lens of required focal lengh and that is why it is known as lens maker.s formula.
Assumptions:
Consider a convex lens made of a material of refractive index $μ_{2}$ the and placed in optically rarer medium of absolute refractive index $μ_{1}$ . Let $P_{1}$ and $P_{2}$ be the poles: $C_{1}$ and $C_{2}$ the centres of curvature and $R_{1}$ and $R_{2}$ the radii of curvature of the two surfaces $X P_{1} Y$ and $X P_{2} Y$ of the lens respectively. Let C be the optical centre of the lens.

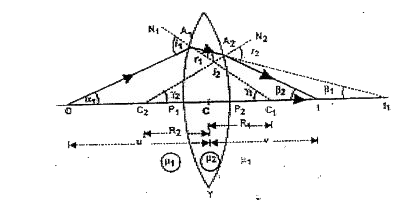
Suppose that O is a point object placed on the principal axis of the lens. The surface $X P_{1} Y$ and forms the real image $I_{1}$ (assuming that material of the lens extends beyond the face $X P_{1} Y$ as such). It can be obtained that
$\frac{μ_{1}}{P_{1} I} + \frac{μ_{2}}{P_{1} I_{1}} = \frac{μ_{1} - μ_{1}}{P_{1} C_{1}}$ ..........i
Since lens is thin the point $P_{1}$ lies very close to the optical centre C of the lens.Therefore, we may write
$P_{1} O \approx C O, P_{1} I_{1} \approx C I_{1}$ and $P_{1} C_{1} \approx {CC}_{1}$
Therefore eqn (i) becomes
$\frac{μ_{1}}{C O} + \frac{μ_{2}}{C I_{1}} \frac{μ_{2} - μ_{1}}{{CC}_{1}}$ ........i
Let us now consider refraction from the other surfacse $X P_{2} Y$ of the convex lens. Actually, material of lens does not extend beyond the surfacd $X P_{2} Y$ . Therefore before the refracted ray from point $A_{1}$ could meet the principal axis, it will suffer refraction at the point $A_{2}$ on the second surface $X P_{2} Y$ and the ray of light will actually come to meet the principal at the point I. Thus, point I may be considered as the real image of the virtual object $I_{1}$ (placed in the material of the lens) is formed due to refraction from the surface $X P_{2} Y$ of the lens. It can be deduced that
$- \frac{μ_{2}}{P_{2} I_{1}} + \frac{μ_{1}}{P_{2} I} = \frac{μ_{2} - μ_{1}}{P_{2} C_{2}}$ ..........iii
Again as the lens is thin we may write
$P_{2} I_{1} \approx C I_{1}, P_{2} I \approx C I$ and $P_{2} C_{2} \approx {CC}_{2}$
Therefore the equation iii may be written as
$- \frac{μ_{2}}{C I_{1}} + \frac{μ_{1}}{C I} = \frac{μ_{2} - μ_{1}}{{CC}_{2}}$ .....iv
Adding the equations ii and iv we have
$\frac{μ_{1}}{C O} + \frac{μ_{2}}{C I_{1}} - \frac{μ_{2}}{C I_{1}} + \frac{μ_{1}}{C I} = \frac{μ_{2} - μ_{1}}{{CC}_{1}} + \frac{μ_{2} - μ_{1}}{{CC}_{2}}$
or $\frac{μ_{1}}{C O} + \frac{μ_{1}}{C I} = (μ_{2} - μ_{1}) (\frac{1}{{CC}_{1}} + \frac{1}{{CC}_{2}})$ .......v
Applying new cartesian sign conventions
$C O = - u$
$C I = + v$
${CC}_{1} = + R_{1}$
and ${CC}_{2} = - R_{2}$
There eqn (v) becomes
$\frac{μ_{1}}{- u} + \frac{μ_{1}}{+ v} = (μ_{2} - μ_{1}) (\frac{1}{+ R_{1}} + \frac{1}{- R_{2}})$
or $\frac{μ_{1}}{u} + \frac{μ_{1}}{v} = (μ_{2} - μ_{1}) (\frac{1}{R_{1}} - \frac{1}{R_{2}})$
Dividing both sides of the above eqn by $μ_{1}$ we have
$- \frac{1}{u} + \frac{1}{v} = (\frac{μ_{2}}{μ_{1}} - 1) (\frac{1}{R_{1}} - \frac{1}{R_{2}})$
Since $\frac{μ_{2}}{μ_{1}} = μ$ we have
$- \frac{1}{u} + \frac{1}{v} = (μ - 1) (\frac{1}{R_{1}} - \frac{1}{R_{2}})$

|

Updated on:21/07/2023

Class 12 PHYSICS GEOMETRICAL OPTICS

Topper's Solved these Questions

Similar Questions

BETTER CHOICE PUBLICATION-REFRACTION OF LIGHT-LONG ANSWER TYPE QUESTIONS

Home
Ask Doubt
Profile