Write the general equation for the instantaneous e.m.f of a 50 Hz generator whose peak voltage is 250 V.
To find the general equation for the instantaneous e.m.f of a 50 Hz generator with a peak voltage of 250 V, we can follow these steps:
Step 1: Identify the given values
- Peak voltage (E0) = 250 V
- Frequency (f) = 50 Hz
Step 2: Write the formula for instantaneous e.m.f
The general equation for the instantaneous e.m.f (E) in an AC circuit is given by:
E(t)=E0sin(ωt)
where:
- E0 is the peak voltage,
- ω is the angular frequency,
- t is time.
Step 3: Calculate the angular frequency (ω)
The angular frequency (ω) is related to the frequency (f) by the formula:
ω=2πf
Substituting the given frequency:
ω=2π×50Hz=100πrad/s
Step 4: Substitute values into the e.m.f equation
Now, we can substitute the values of E0 and ω into the equation for instantaneous e.m.f:
E(t)=250sin(100πt)
Final Answer
Thus, the general equation for the instantaneous e.m.f of the generator is:
E(t)=250sin(100πt)
---
- Peak voltage (E0) = 250 V
- Frequency (f) = 50 Hz
The general equation for the instantaneous e.m.f (E) in an AC circuit is given by:
E(t)=E0sin(ωt)
where:
- E0 is the peak voltage,
- ω is the angular frequency,
- t is time.
The angular frequency (ω) is related to the frequency (f) by the formula:
ω=2πf
Substituting the given frequency:
ω=2π×50Hz=100πrad/s
Now, we can substitute the values of E0 and ω into the equation for instantaneous e.m.f:
E(t)=250sin(100πt)
Thus, the general equation for the instantaneous e.m.f of the generator is:
E(t)=250sin(100πt)
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - AAKASH SERIESCHAPTER - ALTERNATING CURRENTEXERCISE - Exercises (Long Answer Questions)
2 Videos
- ALTERNATING CURRENTBOOK - AAKASH SERIESCHAPTER - ALTERNATING CURRENTEXERCISE - Exercies (Short Answer Questions)
4 Videos
- APPENDICES ( REVISION EXERCISE )BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (MAGNETISM AND MATTER )
52 Videos
Similar Questions
Write the general equation of a plane parallel to X-axis.
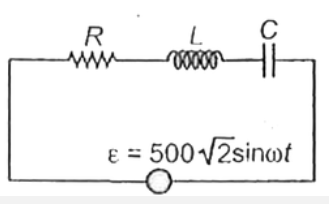
View SolutionIn a series RLC circuit the r.m.s. voltage across the resistor and the inductor are respectively 400 V and 700 V. If the equation for the applied Voltage is ε=500√2sinωt. then the peak voltage across the capacitor is
View SolutionObtain an expression for the instantaneous value of the induced emf in an ac generator.
View SolutionIn general gas equation, PV=nRT,V is the volume of :
View SolutionDescribe briefly, with the help of a labell diagram, the basic elements of an A.C. generator. State its underlying principle. Show diagrammatically how an alternating emf is generated by a loop of wire rotating in a magnetic field. Write the expression for the instantaneous value of emf induced in the rotating loop.
View SolutionAn inductor (L=200mH) is connected to an AC source of peak emf210V and frequency 50Hz. Calculate the peak current. What is the instantaneous voltage of the source when the current is at its peak value?
View SolutionAn inductor (L=200mH) is connected to an AC source of peak emf 210V and frequency 50Hz. Calculate the peak current. What is the instantaneous voltage of the source when the current is at its peak value?
View SolutionFor the given equation write the general solution tan22x=1
View SolutionFind the general solutions of the following equation: secθ=√2
View SolutionFind the general solutions of the following equation: cosecθ= −√2
View Solution
